Bayesian evaluation for the likelihood of Christ's resurrection (frozen copy)
This was the state of the "Bayesian evaluation for the likelihood of Christ's resurrection" post, as of Easter 2018, in the "second draft" form. Some of the formatting has been lost in the blog migration, particularly in the Jupyter notebooks, but the content has been retained. This post will remain unchanged, while the other post will have further edits.
Contents:
PART I: The basic argument
Chapter 1: The priors
- The prior odds against a resurrection
Chapter 2: The evidence
- The nature of the evidence for Christ's resurrection
-- Various scenarios
-- The Bayes factor for a human testimony
-- Double checking the Bayes factor: Lottery winner
Chapter 3: Assembling the basic argument
- Is the evidence enough?
- There is far more than enough evidence to overcome the prior
PART II: Double checks
Chapter 4: Double checking our evaluation of human testimonies
- Why are we double checking? What are we double checking?
- Double check: the Bayes factor of a human testimony.
-- The frequency of lies
-- Car accidents
-- Human death
-- LinkedIn claim
-- Fake 9/11 victims
-- One red dot in a million
-- One in a million events happen every month
-- Video of a lottery winner
-- Summary of the findings
-- The strength of a human testimony is firmly established and understood.
Chapter 5: A deeper understanding of human testimony
- Questions about human testimony
-- The first step in a testimony: the inception of the idea
-- The "human honesty" step, and dependence factors in multiple testimonies
-- The "stretchiness" of human testimony
-- The maximally unlikely, worst case scenario: when the testimony can't stretch
- The resurrection story revisited, with dependence factors
-- Paul's testimony, with full dependence factor
-- Back to the resurrection story
-- Human testimonies stretch to cover the rest of the Bible
-- A fuller understanding of human testimonies validates our previous calculations
Chapter 6: Double checking against the other resurrection reports in history
- Can naturalistic explanations account for the resurrection testimonies?
-- Well, can you demonstrate that empirically?
-- Validating even larger Bayes factors with historical records
-- But what about dependence factors?
- The conditions: the requirements for "matching" a testimony.
-- What are we expecting? Which results would vindicate which hypothesis?
- The other historical records:
-- Apollonius of Tyana
-- Zalmoxis
-- Aristeas
-- Mithra
-- Horus and Osiris
-- Dionysus
-- Krishna
-- Bodhidharma
-- Puhua
- Our previous calculations are fully validated
PART III: Answering simplistic objections
Chapter 7: The usual barrage of objections
- What, if anything, is wrong with the previous argument?
-- Is the prior too large, especially for a supernatural event?
-- "But Science!"
-- Can human testimonies be trusted?
-- Could the disciples have been genuinely mistaken?
-- Or actively deceptive?
-- Or actually crazy?
-- Or some combination of the above, or something else entirely?
Chapter 8: The strength of a Bayesian argument: why none of these objections work
- The nature of Bayesian arguments.
-- Bayesian arguments are not deductive arguments.
-- Bayes factors do not require certainty.
-- Bayesian arguments compel belief.
-- Bayesian arguments are robust.
-- Only evidence moves the odds. Speculations do nothing.
- The lack of evidence against the resurrection.
PART IV: Addressing all possible alternatives
Chapter 9: Time to address the crackpot theories
- The next steps
- Examining crackpot theories, in general
Chapter 10: The "skeptic's distribution" approach.
- Using the historical data to construct the skeptic's distribution
-- What should be the form of this "skeptic's distribution"?
-- Details of the distribution: generalized Pareto distribution and its parameters
-- But how should we determine the value of the shape parameter?
-- More non-Christian resurrections reports make Jesus's resurrection more likely
Chapter 11: Calculation and confirmation using the "skeptic's distribution"
- The calculation plan: obtaining and using the "skeptic's distribution"
-- Simulation and code: The number of "outliers" decides the case.
-- The list of outliers. These put the chance of Christ's resurrection over the top.
Chapter 12: Tuning the "skeptic's distribution" approach
- We were far too generous for the "skeptic's distribution"
-- The power law distribution
-- The uniform distribution over shape parameters
-- The sample size, in the number of reportable deaths
-- The quality of the other resurrection reports
-- The number of non-Christian outliers
-- The region of integration
-- A better estimate of the probability
- The simulation and code, revisited with more likely values
Chapter 13: Defenses against crackpot theories
- The pro-resurrection arguments we have yet to consider
- Defenses against crackpot theories built in to Christianity
-- Apostle Paul
-- Apostle James
-- The diversity among the 12 disciples
-- The diversity among the earliest converts
-- The inclusion of women
-- The divisions in early Christianity
-- Persecution and further division
-- The "final" odds for the resurrection
- Conclusion: the resurrection is still certain, even after taking every possibility into account
PART V: More double checks
Chapter 14: Double check: reports of miracles in other religions
- The stance on non-Christian miracles
- Ichadon
- Vespasian
-- "Something happened" vs. "a miracle happened"
- Splitting the Moon
- Accounts in Josephus
-- Honi the Circle-drawer
-- Eleazar the exorcist
-- "Something happened" vs. "a miracle happened", again
Chapter 15: Double checks of the "skeptic's distribution" approach
- More double checks
- Double checks: conclusion
PART VI: Challenge and conclusion
Chapter 16: The final challenge: replicate the results
- The rationale for this challenge
- The conditions for the challenge
Chapter 17: Conclusion and epilogue
- Conclusion
- Epilogue
PART 1:
The basic argument
Chapter 1:
The prior
The prior odds against a resurrection
What is the probability that Jesus rose from the dead?
Here I'm going to construct a rather foolish partner to advance certain arguments. This is just a rhetorical device. I have to be careful to not commit a straw man here, nor do I wish to insult anyone. I don't intend to imply that anyone actually thinks like my partner. But while he's too foolish to actually stand in for any specific person, he can therefore be useful, by standing in as the lower bound on what a reasonable person may think. Please just understand him as the artificial rhetorical construction that he is.
Now, my foolish partner may say, "the probability that Jesus rose from the dead is zero. What's there to talk about?" But by doing so, he has committed the cardinal sin in Bayesian reasoning. Any real, non-theoretical probability CANNOT be absolutely zero or one. Think about what a zero probability value means: this represents a state of mind where absolutely NOTHING - no amount of possible evidence - can alter their beliefs. There is no reasoning with such a person.
I am very certain that the sun will rise tomorrow. I may be 99.9999...% certain, but I cannot be 100% certain. That tiny difference between 99.9999...% and 100% represents possibilities like a super-advanced alien race stopping the rotation of the earth, or me being momentarily confused about what is meant by "the sun". And I am not 100% certain, because I can, at least in theory, be shown evidence that such an alien race exists, or that I had momentarily confused "the sun" with "the north star".
My partner may then say, "well, the probability may not be actually zero, but it's very close to it. Like, 0.000.....001%. Nobody has ever come back from the dead before." But actually, isn't that the very thing we're talking about? Whether Jesus had come back from the dead? Furthermore, it's presumptuous to think Jesus was just like everyone else, that he wasn't special in any way. Even if nobody else came back from the dead, we would need to do some additional thinking in the case of Jesus.
My partner would reply, "see, that's just special pleading. I don't see why Jesus should be special. Empirically, people do not come back from the dead. Therefore it's also highly unlikely that Jesus came back."
At this point, I'm going to simply give away the point about whether Jesus was special or not. I obviously believe that he was - but quite frankly, the argument for the resurrection is so strong that I can just handicap myself in several different ways like this without materially affecting the conclusion. I'll be doing this multiple times throughout this post.
Now, back to how many people came back from the dead, "empirically": how many different people have you seen die and stay dead? Remember, we're talking about "empirical" evidence here, meaning that we only count people that you, yourself, have seen die in person. For many people, that number is probably zero. It might be one or two - maybe you've seen a grandparent pass away. Maybe more, if you're a healthcare worker or something like that.
My partner may say, "Even if I didn't see someone die in person, if there was a real resurrection, it would be all over the news. And there hasn't been any such reports, because people do not rise from the dead."
Well, at this point, my partner is begging the question on whether there has in fact been such reports, and is becoming slippery about what "empirically" means. But again, I will simply handicap myself and give away these points. "Empiricism" in the sense of "I only believe what I can see" is fundamentally flawed, anyway (It's self-defeating). So let's adopt a more reasonable form of empiricism, and say that news reports are enough, and a direct observation is unnecessary. So, how many people have been covered in the news that you've seen? Thousands? Millions? If the argument is that Jesus was no different than these thousands or millions of other people, then I freely acknowledge that this does in fact establish an upper bound on the probability of the resurrection. However, this does NOT prove that the probability is zero, no more than a dozen coin flip of heads proves that the coin will always land heads. Instead, it merely says that the probability for the resurrection is likely to be below a certain level.
For example, say that you've examined a thousand swans, and they all turned out to be white. You want to use this fact to investigate the report of a black swan. Now, your study of a thousand white swans don't prove that black swans are an impossibility, corresponding to a probability of zero. Instead, it impose a prior probability against that reported black swan: there is only somewhat less than 1/1000 chance that the reported swan is actually black. If you've examined a million swans, and all of them were white, then your probability of observing a black swan would correspondingly drop to around 1/1 000 000 as the upper limit.
Now, the modern media is pretty comprehensive, so my partner may say, "The world news covers many millions of other people. And none of them have come back from the dead. So the chance that Jesus came back from the dead is, at best, one in a million. That's basically zero. How could you believe in something that has only one in a million chance of being true? That's irrational."
Well, one in a million is a pretty small probability. But actually, I think we can just go ahead and say that out of the entire world population of 7 billion people, none of them are going to be raised from the dead. So, the probability for the resurrection has now dropped to 1 in 7 billion. I'm just giving away everything here. I've almost dropped the condition about an "empirical" probability. I'm making a blanket statement that absolutely nobody in the world, independent of anything that may be know about them, will rise from the dead. So, if we apply this general "observation" to the likelihood of Jesus's resurrection, that probability must be below 1 in 7 billion.
My partner may respond, "Um... So now you're making my argument for me. So yeah. The probability of the resurrection is less than 1 in 7 billion. Obviously you can't believe in something that unlikely to be true. This is why any naturalistic explanations must always be preferred to a supernatural one in these discussions of miracles, because the supernatural is always so unlikely."
Oh, but I'm not done yet. I'm going to give away even more of the argument. Why not just drop all pretense of an "empirical" probability? Why not say that everyone who has EVER lived - about 100 billion people in total - have all died, without a single one of them being raised from the dead? Forget saying anything about "empirical observations". Forget any semblance of reasoning from our direct experiences. Ignore anything about reported resurrections. I will simply grant that every single one of these 100 billion people have died and stayed dead. And against the weight of those 100 billion people, we'll estimate the probability of Jesus's resurrection. According to our previous line of thinking, this puts that probability at 1 out of 100 billion.
My foolish partner may say, upon the strength of this evidence that I have made up for him, "One in a hundred billion! Do you know how unlikely that is? That's 1 out of 100 000 000 000. That's a probability value of 0.000 000 000 01. That's basically zero. Just concede the argument - it's virtually impossible that Jesus rose from the dead. Absolutely any naturalistic explanation is going to be more likely than that."
Well, should I just concede? That does seem like a very impressive number, no? How did we even get to this point? I started with the "Nobody rises from the dead, so Jesus also didn't rise from the dead" argument. I then stretched it to its strongest form, to include the entire current world population. I ignored all objections about the specifics in Jesus's case, or the exact meaning of "empiricism". But all that wasn't enough - I wanted it to be stronger still. So I then added some made-up stuff on top, to strengthen it even further, to a level beyond any possible empirical justification.
So now, as it stands, the probability of Jesus actually having risen from the dead is 1 out of 100 000 000 000 - essentially zero. That's game over, right? How could I, or anyone, believe in something so unlikely to be true? How could any hypothesis with a probability of 0.000 000 000 01 ever be taken seriously?
"Um... so yeah. What are you doing?", my partner may ask.
You'll see. Read on, and you'll behold and understand the power of evidence.
Chapter 2:
The evidence
The nature of the evidence for Christ's resurrection
That probability value for the resurrection - 0.000 000 000 01 (which can be written as 1e-11, employing scientific notation) - is a prior probability. That is, it's a probability based on the background information, taking into consideration the fact that Jesus was human, and that humans don't rise from the dead.
However, it is just the starting point. To proceed from this point on, let us first consider some scenarios - they may seem like a detour, but we'll be back on track soon enough.
Various scenarios
Let's say that you're meeting someone new. You talk for a while, and the conversation turns to birthdays. You reveal that you were born in January, and your new friend says, "Oh, really? I was born in January too!" He seems earnest - he's not obviously joking, sarcastic, or ingratiating. From the little you know of him, he's not any more likely to be delusional or deceptive than anyone else.
Now, based only on his earnest word, would you be willing to believe that your new friend really was born in January? Note that I'm not looking for 100% certainty here. A willingness to entertain the idea, to give it at least a 50-50 shot of being true, is all that's required.
Also note that I'm not asking whether this event is likely to happen. Obviously, the probability that you and a random other person shares the same birth month is about 1/12, so it may be said to be "unlikely". Rather, I'm asking whether you would believe this person, given that this unlikely event has already occurred.
So, how would you respond? Would you say, "I find your claim to be highly dubious. There's only 1/12 chance that you were born in the same month as me"? Or would you simply reply, "Oh, hey, that's neat!"
I'm going to assume that you're willing to believe your new friend. I think you'll agree that it takes a special kind of jerk to say "I don't believe you. You must be lying or mistaken. It's just too unlikely for us to share the same birth month". In that case, what if it turned out that you share the exact same birthday? You mention that you were born January 23rd, and he claims the same. Would you still believe him?
Let's continue the same line of thought: what if you revealed to him both your and your mother's birthdays, and it turned that they matched his and his mother's birthdays? What if you had shared your whole family's birthdays, and his mother, father, and his own birthdays all matched with your family's? "Wow", he says, "so the three members of our family all share the same birthdays - amazing!" Would you be willing to believe him on this?
If so, at what point in comparing family birthdays would it become too unlikely for you to believe? That is, if you continued on to compare your grandparents and uncles and cousins, and they all continued to have the same birthdays, at what point would you say "I cannot believe this - this is too unlikely to be true", in spite of your friend's sincere and insistent claim?
Decide on an answer, and remember it. Write it down somewhere. We'll come back to this answer soon. Make a firm statement like, "I would be willing to believe up to 3 shared birthdays - myself, mother, and father - but if he claimed 4 or more shared birthdays I would begin to be skeptical".
Let's try another example. Let's say that you run into an acquaintance whom you haven't seen in a while. You exchange greetings and ask how he's been, and he excitedly tells you - "Guess what! I've actually won the jackpot in the lottery last month! I'm rich!" As before, he seems earnest - he's not obviously joking, sarcastic, delusional, or deceptive. Would you believe him, based only on his earnest word? Again, only a willingness to entertain the idea, just granting a 50 - 50 chance of it being true, is all we're looking for here. Would you give him at least even odds that he's telling the truth?
And if you would, how about if he claimed to have won two consecutive jackpots? How about three? At which point would you say "That's just too much for me to believe"?
Next, let's switch over to other gambling games. Say that a friend claims to have had a very lucky night at the card tables. He says that he got a royal flush in a 5-card stud poker game. Would you believe him? What if he claims to have gotten two royal flushes last night? What if he claims three? At what point would you say, "I don't believe you. You seem earnest and all, but the chances of that happening are just too small"?
How about if he were playing Texas Hold'em, and claims to have had multiple pocket aces? Say that he claims to have had two, three, four, or five pocket aces last night. At what number does it become too unlikely to be true, despite your friend's sincere claim?
We can ask similar kinds of questions in many different ways. What if someone claims to be born as a part of twins, triplets, quadruplets, or quintuplets? What if someone claims to have recently been struck by lightning? Or that they were a victim of two or three such strikes?
Remember, in all these cases, that we're not looking for certainty. Just a willingness to grant even odds - a 50-50 likelihood for the statement is true - is enough to say that you'd believe your friend. Also, we're not asking whether these scenarios are likely; rather we're asking if you'd continue to believe this earnest person, despite the fact that he's claiming that an unlikely event happened.
Answer these questions. Give a specific number in each case: we want answers like "four royal flushes" and "two lightning strikes". Write them down somewhere - we'll come back to them later.
Now, we'll turn to the question of Jesus's resurrection.
The Bayes factor for a human testimony
Recall that we had rather generously put the odds against Jesus's resurrection at a prior odds of 1 out of 100 billion (that is, 0.000 000 000 01, or 1e-11).
Recall also that this was only the starting point. It does not take into account any evidence we have specifically about Jesus's resurrection. Remember Bayes' rule: the final, posterior odds is the prior odds times the Bayes factor, which is the likelihood ratio of the hypotheses predicting the evidence. The number we have now is just the prior odds. We now need a numerical value for the Bayes factor of the evidence, and then we can get our posterior odds.
But what kind of evidence is there for Christ's resurrection? And how could it possibly overcome a prior odds of 1 to 100 000 000 000 against it? Well, as for the evidence, we have the writings of the New Testament, where Jesus's resurrection and his follower's testimonies are documented. Okay, but is this "evidence" any good? How can we put numerical likelihood ratios to these things?
What we need is the numerical strength of a human testimony. As it turns out, we can actually get a not-unreasonable, order of magnitude estimate of this value. Remember your answers to the probability questions in the previous section? I hope you have them written down or otherwise recorded, because we will use them to calculate the Bayes factors that you would personally assign to a typical human testimony.
Let's use my personal answers, given below, as an example for how to do these calculations. These are my gut answers to the questions, before doing an actual probability calculations. Remember, "believe" here means that I'm willing to give at least even odds (50/50 chance) on the claim. It doesn't mean certainty, and it doesn't mean that I'd stop looking for more evidence. It only points to how much I'm willing to adjust my beliefs based on someone saying "yes, I know it's unlikely, but it really happened".
For the shared birthday question, I would easily believe that my friend shared a birthday with me. I would also not have any real problem believing that our mothers also shared birthdays. At three people - myself, mother, and father - I would start becoming skeptical, but would probably give my friend the benefit of doubt. Starting with four shared birthdays in the family, I would start leaning more heavily towards skepticism.
On winning the lottery, I would not really doubt that my friend won the lottery. I would start doubting if he says that he won two consecutive lotteries.
On getting a royal flush, I think I could almost believe that my friend got two such hands in a very lucky night at the table. I feel like three would be entering the realm of the fantastical, and I would doubt my friend at around this number.
On pocket aces, I would be willing to believe that my friend had up to four or five pocket aces in a lucky night of Hold'em.
On the multiple births, I would not have any real problems believing that someone was a part of quadruplets. A claim to be in a quintuplet would start to cause a little bit of doubt to me, and a claim of sextuplets would need additional evidence.
On being struck by lightning, I actually had someone around me claim that this had recently happened to her. I had no problem believing it. Even if she had claimed two such accidents I don't think I would have really doubted her. If she had claimed three, I would start to be skeptical.
Now, calculating the numerical probability values for all these things is pretty straightforward:
The probability of sharing a single birthday is 1/365, or 1/3.65e2. The probability of sharing the three birthdays for your family is then simply this number cubed - 1 in 4.86e7.
The probability of winning the lottery varies by exactly which lottery you're talking about, but the odds for the jackpot are generally somewhere around 1 in 1e8.
The probability of getting a single royal flush is 1 in 6.5e5. The probability of getting two in two hands is therefore this number squared, 1 in 4.2e11. We can then take it down by a couple orders of magnitude, to account for the fact that there's dozens of hands played in a poker night. That gives us something like 1 in 1e9 for the odds.
The probability for getting pocket aces is 1 in 221. Getting five would then be 1 in 5.3e11. Taking it down again by several orders of magnitude to account for multiple hands, that brings us to something like 1 in 3e7.
The probability of quadruplets is about 1 in 1e6, and for quintuplets it's about 1 in 5.5e7. We'll split the difference here and call it 1e7.
The probability of getting struck by lightning in a given year is about 1 in 1e6. If we count "recently" as the last 5 years, that would bring it down to 1 in 2e5. Getting struck twice would then be 1 in 4e10, then maybe take off an order of magnitude for possible dependency factors to give us 1 in 4e9.
So, looking at the final numbers above - 1/4.9e7, 1/1e8, 1/1e9, 1/3e7, 1/1e7, 1/4e9 - we seem to be getting a reasonably consistent estimate for how I value the strength of an earnest, personal testimony. There are a lot of small details we can go over again (how many hands of poker did you play last night? Is your friend someone likely to play the lottery, or to be outdoors during a thunderstorm?), but these will largely be random, small, unknowable effects that will get washed out in this order-of-magnitude calculation.
So, we'll take the geometric mean of the above values(1/1.3e8), and then conservatively round down to get 1/1e8, or 1e-8, as their "average" probability. In other words, even if an event had only a 1e-8 prior chance of happening, I would be willing to give even odds on that event having occurred based on someone's earnest, personal testimony.
At such small probability values, "probability" is nearly synonymous with "odds". Therefore, I can re-state the above as saying that an earnest, personal testimony will shift the odds from 1/1e8 to 1/1. Or, to put it yet another way: the typical Bayes factor for an earnest, personal testimony is around 1e8. That is my numerical value for the strength of a human testimony.
It is important to note that this number is not something that I just made up. The math that gives this value is described above in its entirety. What answer did you get when you plugged in the numbers? That is the number that you, personally, must be willing to assign to the strength of a personal testimony, if you are to be consistent. I believe that most reasonable people will be within a couple of orders of magnitude of my answer.
Double checking the Bayes factor: Lottery winner
Now, we don't want to just take someone's personal answers and simply run with it - even if that someone is ourselves. It is important to double check our answers. Fortunately, there are a number of different ways to do that.
The simplest way is to perform a thought experiment on yourself. Imagine a future where you yourself are telling someone, "I just hit the jackpot in the lottery". You are being sincere and insistent. Now, what is the probability that you're telling the truth here, in your own hypothetical future?
Given that the odds of winning the lottery is about 1/1e8, if you agree with my assessment that personal testimony should be valued at a Bayes factor of around 1e8, then you are about equally likely to be telling the truth or lying in this scenario. However, if you disagree with that assessment - for example, if you think that personal testimony should only be valued at 1e6 - then you're saying that the posterior odds of you having won the lottery is still only 1/100, and so you're 99% likely to be lying in that scenario. Which is it?
In fact, this thought experiment suggests a way to empirically verify this value, completely apart from your own answers. Simply investigate a random sample of the people who claimed to have won the lottery. Remember, we're only counting earnest, personal claims to the jackpot. What fraction of them are telling the truth? How many of them are actual lottery winners? If you say "maybe around half?", then you're agreeing with my Bayes factor of 1e8. If you want the Bayes factor to be 1e6 instead, then you need 99% of these people to be liars.
Do you still doubt that you can assign a numerical value to the strength of a personal, human testimony? Or maybe worry that the correct value is far from 1e8? Well, fortunately for us, this "lottery liars" experiment has actually been naturally conducted, and we can compare its result with our numbers.
On January 13, 2016, the Powerball lottery produced the largest jackpot in history (as of the time of this writing): 1.6 billion dollars. This jackpot ended up being split three ways. But - were there people who lied about having won this jackpot? As a matter of fact, there were. Several people on social media claimed to be a winner, presumably in an attempt at some quick, cheap fame. How many such people were there?
I couldn't get an exact number for the number of Powerball jackpot liars, but we can still get a sense, an order-of-magnitude estimate. Snopes, for example, mentions two people by name, and "several" or "numerous" others. Another report claims "a number" of similar hoaxes. So - it sounds like maybe ten people lied about winning the jackpot? It's certainly not in the hundreds or thousands.
How does that compare with the estimates from my probability calculation? Well, the odds of hitting the jackpot in Powerball are about 1/3e8. However, people may buy multiple tickets - which many people certainly did on such a well-publicized jackpot. In the end, there were 3 actual winners, out of the total American population of 3e8 people. So the prior odds for a specific person in the United States being a winner was 3/3e8, or 1/1e8.
Now, if the Bayes factor for an earnest personal testimony is 1e8, then the posterior odds is just the product of 1/1e8 and 1e8, which is 1. That translates into 1 actual winner for every liar. So, given that there were 3 actual winners to the jackpot, we should expect around 3 liars - and that is roughly what we actually appear to have, within an order of magnitude.
You can again nitpick at this example (the great publicity of this jackpot, the people who made an earnest claim offline, the relative certainty of a short-lived notoriety for lying, etc.) But as an order-of magnitude estimate, the results of this natural experiment are about as good as I can possibly hope for. So, the proper Bayes factor for an earnest, personal testimony is typically about 1e8, and this has now been validated through multiple lines of thought. It is certainly not several orders of magnitude less than that.
There are many other ways to check this number, which we will return to later. They all converge around 1e8. But for now, let's proceed with the rest of the calculation, using 1e8 as the Bayes factor of a human testimony.
Chapter 3:
Assembling the basic argument
Is the evidence enough?
Now that we have all the necessary numerical values, we can finally calculate the probability that Jesus rose from the dead.
To begin, I gave the prior odds for Jesus's resurrection as 1e-11. This number was obtained from the argument that "empirically, people do not rise from the dead. Therefore, Jesus also couldn't have risen from the dead." I took that argument, then made it as strong as possible, then gave away everything that it asked for, then gave away even some more things that it didn't ask for, to the point of strengthening it beyond all bounds of empiricism. This number is equivalent to a prior obtained by individually checking and confirming that every single person who has ever existed has failed to resurrect. In other words, this 1e-11 is a smaller probability than anything that any skeptic can reasonably ask for.
Next, we calculated a typical value for Bayes factor, for a seemingly earnest, sincere, personal testimony. It worked out to be about 1e8. It's certainly not much less than that in the relevant cases.
Now, we simply apply Bayes' rule: posterior odds are prior odds times Bayes factors (the likelihood ratio). So, we'll just look through the New Testament, and see if we can find people who made an earnest, personal claim that Jesus rose from the dead. Let's start in 1 Corinthians 15, because that's a famous passage on the resurrection, recognized even by skeptical scholars as originating within a few years of Jesus's death. It's a good partial summary of all the other resurrection-related testimonies in the New Testament. It reads:
For I delivered to you as of first importance what I also received: that Christ died for our sins in accordance with the Scriptures, that he was buried, that he was raised on the third day in accordance with the Scriptures, and that he appeared to Cephas, then to the twelve. Then he appeared to more than five hundred brothers at one time, most of whom are still alive, though some have fallen asleep. Then he appeared to James, then to all the apostles. Last of all, as to one untimely born, he appeared also to me.
So, who in this passage can be said to have made an earnest, personal claim of Jesus's resurrection? Well, there's Cephas, also known as the apostle Peter. He's a major character in the New Testament, and every one of the numerous accounts of him says that he did, in fact, testify that Jesus rose from the dead. Certainly, that's one witness. The odds of Christ's resurrection after taking Peter's testimony into account is now 1e-11 * 1e8 = 1e-3.
Anyone else we can find here? Well, there's Paul, the author of the very text we're reading, and one of the most prolific writers of the New Testament. He himself says in this passage that the risen Christ appeared to him. Furthermore, Paul was initially a dedicated opponent of Christianity, before his miraculous conversion. So barring some crackpot conspiracy theories, there's little worry about any strong dependency factors which would significantly reduce the impact of his testimony. In fact, his testimony would naturally expect to be anti-correlated with Peter's, so there may actually be an even larger Bayes factor associated with it. But let's just give that away. We'll just count his testimony at a Bayes factor of 1e8. The odds of Christ's resurrection after taking it into account is now 1e-3 * 1e8 = 1e5, or 100 000 to 1 FOR the resurrection.
Huh, would you look at that. After taking just two witnesses into account, the odds are now in FAVOR of the resurrection. And this is literally using just a fraction of the first passage we chose in the New Testament! Even within this passage, we still haven't taken into account James, or the other members of the twelve disciples, or the other apostles, or the five hundred that are mentioned. And then, we still have the rest of the New Testament to still go through!
What happened? The prior odds was 1e-11 - that's 1 in 100 000 000 000! Wasn't that supposed to be an impossibly small odds? Wasn't it suppose to be insurmountable? Wasn't it something that enabled atheists to simply say, "therefore any naturalistic explanation is bound to be more likely"? Wasn't it a bulwark for skepticism, based on some kind of empiricism? How could it have just... evaporated like that?
That is the power of evidence. Evidence can cause swings in probability that seem ridiculously large to people who are not actually familiar with the mathematics. Did you think that a billion is a large number, or that a probability of one in a billion is too small to ever care about? It is not. In some kinds of math, even numbers like a googol (1e100) can disappear to nothing in just a few lines of calculation. And probability is one example of that kind of math.
Just the other day at my work (Bayes' theorem and probability calculations are part of my day job), a Bayes factor of 1e-10 came up. It merited no comment beyond "that's pretty small". Another time, 1e-40 appeared as a Bayes factor, again with little commentary on its magnitude. Numbers like that are not atypical in probability calculations. Do you realize that, if I specify the order of cards in a shuffled deck of playing cards, that I'm doing so against an odds of 1 to 8e67? That if I hand you a record of a chess game (which can fit on a single post-it note), I'm specifying one out of at least 1e120 possibilities? So, a billion - which is only 1e9 - is not a large number. And the prior odds against the resurrection - which is only 1e-11 - gets completely blown away when it's set against the evidence.
Here, it's important to again note that I'm handicapping the argument for the resurrection. I already mentioned how the prior probability of 1e-11 was far smaller than anything that a skeptic can reasonably ask for. As it turns out, the Bayes factor of 1e8 for a personal testimony is also smaller than it could have been. It's the right value for the general case, but in specific situations it may be far larger. Note the above example of recording a chess game: if you choose to believe that my record of the game is accurate, you're giving me a Bayes factor of around 1e120 for my testimony.
So, as it stands for the moment, the odds are around 100 000:1 in FAVOR of the resurrection, using only Peter and Paul's personal testimonies. The seemingly strong "nobody rises from the dead, so Jesus couldn't either" argument has been fully overcome, with only a tiny fraction of the evidence we have in the New Testament. At this point, the resurrection is already quite probable - but I suppose we might as well finish off the passage we've started on, to see how the odds grow from here.
From here, I'm going to be pretty sloppy for the rest of this calculation, because it just does not matter in the end. The case for the resurrection is just that strong. In particular I'll be setting aside some kinds of crackpot theories for now, which allows me to ignore some kinds of dependence factors. We will address those points more fully later. But for now, this won't affect our conclusion - we're just piling more evidence on top of an already near-certain proposition with the remaining testimonies in 1 Corinthians 15.
So, let's see who else comes up in 1 Corinthians 15. There's James, the brother of the Lord. He's another major character in the New Testament, another major player in early Christianity. We have no doubt that he professed that Jesus rose from the dead. So that's an additional named witness. Taking his testimony into account, the odds of Christ's resurrection is now 1e5 * 1e8 = 1e13 for the resurrection.
Furthermore, 1 Corinthians 15 says that Jesus appeared to "the twelve", and also to "all the apostles", which form two distinct groups. Let's first consider "the twelve": to compute their Bayes factor, we'll go ahead and cut down their number to include only those disciples who are mentioned more often in the New Testament. Say that leaves us with 4 disciples. With some dependency factors and all, let's give each of these disciples a Bayes factor of 100 for their testimony. That value represents a rather low opinion of their trustworthiness: you wouldn't believe such a person even if they told you their own birthday.
Even so, the overall Bayes factor for "the twelve" is still 100^4, or 1e8. If we give also give "all the [other] apostles" the same Bayes factor, the odds of Christ's resurrection now becomes 1e13 * 1e8 * 1e8 = 1e29.
1 Corinthians 15 also mentions Jesus appearing to "more than five hundred brothers at one time". It's clear that Paul had a specific set of people in mind, as they are part of this early central creed, and Paul mentions that some of these people have died. The number 500, too, is not something anyone just made up - it seems as if the passage is extra careful to mention that some have died, because this may have reduced the actual number of living witnesses to below 500. But let's just ignore all that. Let's pretend that Paul (and the early Christians) exaggerated this number by a factor of ten, so that there were only 50 people claiming to have seen the resurrected Christ. Let's furthermore give them a Bayes factor of 1.5 for their testimonies - meaning you wouldn't trust them to report their own gender correctly. Again, even with these low values, their overall Bayes factor is 1.5^50, which is still well over 1e8. The odds of Christ's resurrection, after taking these people's testimonies into account, is now over 1e29 * 1e8 = 1e37.
Now, as I said there's a lot of sloppiness in the above calculation. The dependency factors need to be handled more carefully, and one should be careful of making claims with numbers like 1e37 as the actual, final odds in a real-world context, for at those levels even the crackpot conspiracy theories can come into play. But really, the fact that we have to even worry about that is a testament to the strength of the evidence. We will come back to all these points later - but what IS completely clear, even at this early point, is that the evidence for the resurrection completely overwhelms the prior odds. Jesus almost certainly rose from the dead.
There is far more than enough evidence to overcome the prior
This brings us to the end of the 1 Corinthians 15 passage. We can go through the remainder of the New Testament, but that'd be lot of work to improve an odds that's already near certainty - so this is a good place to stop for now. What have we achieved? Consider:
We have only used the strength of personal testimonies. That is, we've only used the fact some people have said that they have personally witnessed to the resurrected Christ. We have not yet taken into account any other kinds of evidence, such as the fulfillment of Old Testament prophecies, or physical facts like the currently empty tomb, or historical facts like Christianity's explosive early growth, or anything else.
We have used conservative numbers in each step of our calculations.
We have only focused on a single passage from the entire New Testament.
We have only considered a rather weak version of a human testimony, like someone being earnest in a single meeting. That is how we calculated our Bayes factor. But the disciples were more than merely earnest: they were sincere, insistent, and enduring in their claim, and they oriented the rest of their lives around that claim. Over the course of their entire lives, they made the same claim, with the same earnest seriousness, to everyone they would meet. That full set of conditions - sincere, insistent, enduring, and life-changing - merits the elevation of their claim to a whole new level, which we have not considered.
And even under these restrictions, the odds have easily overcome the 1e-11 prior odds against people in general rising from the dead, and has already reached values corresponding to near certainty. Furthermore, I have yet to carry out a more full and reasonable calculation, using all the different lines of evidence that a modern Christian has at his or her disposal, which would certainly add on many more orders of magnitude to the final odds. Jesus almost certainly rose from the dead.
PART II:
Double checks
Chapter 4:
Double checking our evaluation of human testimonies
Why are we double checking? What are we double checking?
My claim, at its heart, is very simple: the evidence of the many people claiming to have seen the risen Christ is abundantly sufficient to overcome any prior skepticism about a dead man coming back to life. My argument consists of backing up that statement with Bayesian reasoning and empirically derived probability values.
The emphasis on empirical probability values is important. Humans are notoriously bad at estimating probabilities, especially when the values reach extreme levels, like 1e-11. Some people, especially when discussing a controversial topic like the resurrection, will just pull numbers out of thin air to support their preconceptions. They'll make statements like "I'll grant a 23.599% chance that the disciples went to the wrong tomb". This can sometimes result in some pretty hilarious statements, like someone assigning a 1% chance for a generic conspiracy theory - as if they couldn't imagine anything less likely than a 1% probability.
This is why having an empirical basis for the probability values is crucial. Otherwise, you're likely to simply make up such worthless numbers, influenced only by your preconceived notions.
In my argument, none of the numbers I used are something I just made up. I gave each of them ample empirical backing. The two important numbers are the prior probability for the resurrection, and the Bayes factor for a human testimony. I set the prior probability at 1e-11: this is, as I said, far more conservative than any requirement of empiricism. One may be able to "empirically" argue that nobody alive today has ever seen a man come back from the dead. This rather generous definition of "empiricism" would set the prior odds at around 1e-9 or 1e-10. But I've gone further. I've chosen the value of 1e-11 by taking the total number of all the humans that have ever lived, then assuming that none of them have ever come back from the dead. As we have not actually checked every person who died recently - let alone remotely come close to checking every person who ever existed - this is a solid lower limit on the prior. There is no way to argue that it should be empirically set lower.
As for the Bayes factor of a typical human testimony, I've set at 1e8. I've given numerous lines of thought which demonstrate that this is about the correct value. These including several examples from everyday life where you choose to trust someone, and the results of a natural experiment with the 1.6 billion dollar lottery in 2016. All these empirically derived lines of thinking converge around 1e8 as the correct value for the Bayes factor of a typical human testimony.
But, this number is perhaps more difficult to accept than the prior probability. There is a large variance inherent in human testimony, and Bayes factors are less familiar and less intuitive as a concept than a prior probability. For these reasons, it'll be worthwhile to obtain a deeper understanding of human testimonies, and demonstrate with a few more real-life examples that the appropriate Bayes factor here is really around 1e8.
In addition, there is the question of how testimonies by multiple individuals stack together. One simple way is to assume independence - then the Bayes factors just multiply together. Now, this can be largely justified in cases like the independence between Peter and Paul's testimonies, as we have done in the argument above. But in the general case such an assumption would be naive.
We need not actually worry about this much, since the key step in the above argument - which takes the odds from "against" to "for" - relies only on that independence between Peter and Paul's testimony. The rest of the resurrection testimonies, with varying degrees of dependence, only serve to push the odds beyond a shadow of a doubt.
But we want to make sure that none of these lingering shadows could possibly hide any uncertainties which may change our conclusion. So the remainder of this chapter will consist of these double-checks: confirming the value of the Bayes factor for a human testimony, understanding the causes of the variance in that value, and demonstrating that the possible interdependence among the resurrection testimonies does not hamper our main argument.
Double check: the Bayes factor of a human testimony.
Our estimate for the Bayes factors for an earnest, personal human testimony can be further confirmed by the following lines of thought.
The frequency of lies
How often have you been lied to? Perhaps, upon considering this question, you may say, "way more than 1 out of a hundred million (1e8) times! There's no way that human honesty has odds of 1e8!" But this makes the mistake of confusing a Bayes factor with the posterior odds.
The math goes something like this: let's say that people have about 10 opportunities to nontrivially lie to you in a given day. Multiplied by about 300 days a year and assuming that you're about 30 years of age, this amounts to about 100,000 (1e5) opportunities for someone to tell you a nontrivial lie.
At this point, you might divide 1e5 by 1e8, and conclude that a Bayes' factor of 1e8 would imply a 1e-3 odds of having been lied to in your lifetime - an obviously absurd conclusion. But this 1e5/1e8 division is a mistake. The absurd conclusion comes from that mistake, and not because 1e8 is the wrong value for the Bayes factor. Remember that the Bayes factor is the ratio between the prior and the posterior odds. It is not the posterior odds itself.
The correct math here requires getting those prior and posterior odds. So, how often have you been nontrivially lied to, in your 30 years of life? Let's say 1,000 (1e3) times. That corresponds to roughly 3 nontrivial lie per month. That means that the posterior odds of a lie is 1e3 out of 1e5 opportunities, or 1e-2. The posterior odds of a truth-telling is therefore 1e2. That is, people generally turn out to have lied about 1% of the time.
But what are the prior odds? In normal situations where people are tempted to lie to you, it's generally quite small. Most specific events are improbable. This is especially the case when someone is making a positive assertion about something that happened - such as "I got into a car accident", "I went to Harvard", or "I was vacationing in France that day". Let us generously assign 1e-3 as the prior odds of the statement being true.
Then, the Bayes factor is the factor which turns that 1e-3 to the posterior odds of 1e2. In this case, it's 1e5, because 1e-3 * 1e5 = 1e2.
The remaining 3 orders of magnitude up to 1e8 are easily made up for by a number of additional factors. First, the kind of personal claim we're concerned with are not trite, everyday lies. With the resurrection, we're specifically concerned about sincere, insistent, enduring, and life-changing personal testimonies. This set of conditions could easily add a couple of orders of magnitude to the Bayes factor. Additionally, the prior odds for the types of events we're considering have far smaller prior odds than 1e-3. We're discussing events like winning the lottery, or being struck by lightning, or someone rising from the dead. So for such a testimony about such an event, 1e8 is quite appropriate, and its value is in fact borne out by this rough estimation.
Car accidents
Imagine that you've arranged to have an important meeting with me on a particular date, but I don't show up to the appointment. You're understandably peeved, but then you get a phone call from me saying, "I got into a car accident. I'm okay. But I'm really sorry that I couldn't make it to our meeting today. Can we reschedule?"
Now, would you believe my story? Did I really get into a car accident on the day of our appointment? What would you assign as the probability that I'm telling the truth?
The average driver gets into a car accident roughly once in 18 years. That's about once every 6500 days. So the prior probability for getting into a car accident on a particular day is 1/6500. If you choose to believe me - say, you think there's more than a 90% chance that I really was in an accident - then you've changed the odds for my car accident from 1/6500 to 10/1, and you've therefore granted my phone call a Bayes factor of 65000 - or nearly 1e5.
From our earlier calculation, a Bayes factor of 1e5 would already be easily sufficient to make Jesus's resurrection quite likely. Peter and Paul's independent testimonies would add up to 1e10, and the great mass of testimonies by everyone else - James, the other disciples and apostles, and the crowd of 500 - would easily contribute at least a factor of 1e1. That's amply sufficient to overcome the initial 1e-11 prior.
In other words, if you would believe that I got into a car accident, you ought also to believe in the resurrection. Otherwise you're being inconsistent. If you wish to disbelieve the resurrection, you must also be the kind of person who says, "I don't believe you. I think you're lying about the car accident. You need to give me additional evidence before I believe that something that unlikely happened".
Ah, but maybe the people who are skeptical of the car accident are right? Maybe we should be more skeptical in general? It might be the polite thing to do to believe someone in such situations, but how do we know that that's actually the mathematically right thing to do?
Well, this is where the fact that this actually happened to me comes into play. I once got into a car accident on my way to a wedding. I was not hurt, nor was my car seriously damaged - but the whole affair did cause me to miss the entire wedding ceremony. I only managed to show up for the reception afterwards. That day, I told numerous people that I had gotten into a car accident, and gave it as my excuse for missing the ceremony. Not a single one of these people doubted me in the slightest: they all believed me. And they were right to do so, because I had in fact gotten into a car accident.
In fact, I've never heard of anyone, anywhere falsely using the "I had a car accident" excuse for missing an appointment. There are simply no reports of it that I know of. This is in spite of the fact that I have heard of numerous car accidents, and have been in one myself, and have heard it used as a genuine excuse before. All this, combined with the great deal of trust that the others correctly put in me when I told them of my car accident, tells me that the earlier 90% chance for the accident is too conservative. If I were to hazard a guess, I would say that such car accident stories are trustworthy about 99.9% of the time. That means that the posterior odds for the car accident are about 1e3, and the Bayes factor from an earnest, personal testimony about a car accident is about 1e7 - although this is admittedly somewhat speculative.
So, if someone tells you about their car accident on a particular date, the Bayes factor for their testimony should at least be 1e5 as a lower bound, and probably (but more speculatively) around 1e7.
Now, what if someone claims to have gotten into two car accidents in one particular day? The prior odds for such an event, assuming independence, is about 1e-7.6. Now, I have not heard anyone make this claim exactly, but I have heard of somewhat comparable events, like two tire blowouts happening on the same day (this, too, actually happened to me once). The comparison is difficult to make, as there are strong dependence factors and statistics on blowouts are harder to come by. However, going on my intuition, and my experience with similar events like blowouts, I would be willing to believe someone who claimed to have had two car accidents on a particular day, or at least give them even odds that they're not lying. This gives their testimony a Bayes factor of about 1e8. While this is not a solid measure of the Bayes factor on its own, it does validate my earlier estimation of the Bayes factor being around 1e7.
Finally, note that this is all in the context of a single phone call, or a single conversation with wedding guests. The sincere, insistent, enduring, and life-changing claim of the early Christians must be accorded a correspondingly greater Bayes factor.
Human death
You're talking to a friend that you haven't seen in a year, and you're exchanging news about mutual acquaintances. You ask, "how's Emma doing?" Your friend then replies and says:
"Oh, you haven't heard? Emma... is dead. She was killed in a car accident. And you know how she was really close to her mom? Well, when her mom heard the news of Emma's death, she committed suicide - they say that they had the funeral ceremony for both of them together."
You may have guessed that this, too, actually happened to me. A friend of mine told me this tragic story about a girl we both knew. Don't be too concerned - the name of the girl has been changed, and this happened long ago - long enough ago that all the parties involved must have gotten well past the shock and the grief.
But, let us turn back to the question at hand. Should I trust my friend, on this very unlikely story? The yearly car accident fatality rate is about 1 per 10,000. The suicide rate is about the same. My friend's story, therefore, has a prior odds of about 1e-8 of being true. There is some dependence factors which increase the odds (a mother is more likely to commit suicide after her daughter's death), but the specifics of the story (the specific cause and timing of the suicide) would again decrease the odds. Let's say that they basically cancel each other out.
I'll go ahead and tell you that I did believe my friend. I did not really doubt his story. If I had to put down a number for my degree of belief, I would say that I gave his story about a 1e3 odds of being true. So the odds for this sequence of events went from a prior of 1e-8 to a posterior of 1e3, and therefore the Bayes factor for my friend's testimony is about 1e11.
But was I right to trust my friend? Maybe I should have said back to him, "I don't believe you. Your story is just too ludicrous"? Well, as it turns out, I did get independent verification for a good chunk of this story later on. I really was right to trust my friend. Given that this is only a single instance of verification, this only validates that I was right to trust my friend, but not necessarily that I was correct to give the story a posterior odds of 1e3. So, at a minimum, I was definitely justified in giving my friend at least 1e8 for the Bayes factor as a lower bound, and I feel that the correct value should actually be closer to 1e11.
LinkedIn claim
The previous examples were drawn from stories and experiences that I have personally lived through and verified. But perhaps you're not convinced by the stories from my past. Fair enough - they're event that I have directly experienced, so they're empirical for me, but they're not empirical for you.
Here, then, is a calculation that anyone on the internet can verify to get an empirical value for the Bayes factor of a human testimony. All of the raw numbers in the following calculation are provided for the time of this writing (June 2016).
Go on LinkedIn, and search for "PhD physics Harvard". You'll find many people who claim to be in the PhD program at Harvard University. You may need to upgrade your LinkedIn account to see the profiles for these people, if they're outside your network. Now, are these people telling the truth? And what ought we make of their claim that they're getting the most advanced degree in the most challenging field from the most prestigious university in the world? And what is the Bayes factor for that claim?
To address this, we first need to find the prior probability for someone on LinkedIn being in the Harvard physics PhD program. For this, we'll need to gather up some numbers - all of which are readily available online.
First, let's get the number of people in Harvard's physics PhD program. This is easy enough - their department's webpage tells you that they have about 200 graduate students.
It's also easy to find the number of people on LinkedIn. Their website will tell you that they have more than 128 million registered members in the United States.
Now, we'll make the generous assumption that all 200 people in Harvard's physics PhD program are on LinkedIn. This means that the prior probability for someone on LinkedIn actually being in the program is about 200/128 million, or about 1e-6.
What about the posterior probability? Well, we can take the people on LinkedIn who claim to be in the Harvard physics PhD program, and actually investigate them one by one. Many research groups have their rosters published online, so you can easily find out whether someone really is in a physics research group at Harvard. You may also find their scientific publications or teaching records online, all of which can confirm their status in the program.
So, I searched on LinkedIn for "PhD physics Harvard". I spot checked more than a dozen people from the search results who claimed to be in the Harvard physics PhD program. I chose my sample over many pages across the unfiltered LinkedIn search results, so that the "relevance" of the search results to me will not influence my sampling.
What was the result? I found that every single person I checked was telling the truth. I could verify each of their claim independently from the LinkedIn page, nearly always from an official Harvard physics department page. Since I had checked over a dozen people, this represents a posterior odds of 1e1 at a minimum for these people really being in the Harvard physics PhD program.
This means that, at a minimum, the mere claim of these individuals on LinkedIn changed the odds for that claim, from a prior value of 1e-6 to a posterior value of 1e1. Therefore, the Bayes factor for these claims have about 1e7 as a lower bound. The actual value is therefore well within range of the 1e8 value that we've been using.
It's also important to note how weak a claim on LinkedIn is compared to the kind of earnest, personal testimony that we're interested in. Anyone can get a LinkedIn account; they just have to sign up for it. They can then say whatever they want in that account. Furthermore, there is not much concrete negative consequences for lying, while the incentive of getting a job or a business contact can be quite appealing. At worst you'd lose a job that you'd have not gotten otherwise anyway. But even with all this going against it, the people on LinkedIn turn out to be quite trustworthy, with the Bayes factor for their claims having a value near 1e8.
The Bayes factor for the disciples testifying to Christ's resurrection must be worth at least that much.
Fake 9/11 victims
Here is yet another example from which we can empirically derive the Bayes factor for a human testimony.
The September 11 terrorist attacks killed about 3000 people. It is the worst terrorist attack in world history to date. As such, it caused a great deal of shared grief and an outpouring of sympathy for the survivors and the families of its victims.
Of course, human being being what they are, some people falsely claimed that a close loved one had died in the attacks. This got them a lot of sympathy - and more importantly, it got them a great deal of aid money, exceeding a hundred of thousand of dollars in some cases.
This naturally leads us to ask - how reliable was a person's claim that they had lost a loved one in the 9/11 attacks? What was the Bayes factor for such a claim? The numbers for this calculation are readily available. We just have to assemble them.
First, let's calculate the prior probability that someone really did lose a close loved one in the 9-11 attacks. We will assume that every one of the 3000 victims had about 4 loved ones (father, mother, sister, son, etc) whom we can consider "close", and that all of these loved ones lived in New York City. This gives 12,000, or about 1e4, close relation of the victims in a city with a population of 1e7. Therefore, the prior odds for a random person in New York City actually having a close loved one as a victim is about 1e-3.
Now, if someone claims that they had a close loved one die, what is the posterior odds that this person is actually telling the truth? One may assume that a vast majority of the 1e4 actual close relations of the victims made that claim. But how many false claims were mixed in with those? The specific number is not possible to determine (as someone could have lied so well that they were never suspected), but the article I previously linked mentions numbers like "dozens", "two dozen", or "37 arrests". Taking these numbers into account, let us be generous here and assume that there were 100, or 1e2, false claimants. The posterior odds are therefore 1e4:1e2, which is equal to 1e2.
Therefore, the Bayes factor for someone claiming to have lost a loved one in the September 11th terrorist attacks is sufficient to take the odds from an empirically calculated prior value of 1e-3 to an empirically calculated posterior value of 1e2 - so it must be given a value of 1e5.
Nearly all of the numbers here are from Wikipedia or the New York Times. You can follow up on their sources and verify the values yourself. In the few places where I had to make assumptions, they have a definitive bias towards reducing the Bayes factor - for example, the people who lost loved ones are not all confined to New York City, and 100 false claimants are a good deal more than two dozen. There's probably also a greater tendency for the truth-tellers to communicate their loss to more people in cases like these. Therefore, 1e5 is an underestimate of the true Bayes factor. The actual value is greater - 1e6 seems like a reasonable guess.
Consider what this means: even when there was a clear reason to lie - that is, even when there was cold, hard cash at stake as a tangible reward for lying - people turned out to be fairly reliable overall. The Bayes factor for their earnest claim about the personal tragedy of losing a loved one turned out to be about 1e6. Now, the types of testimonies we're interested in would not have the explicit possibility of fraud as a precondition, and we would not be constrained to only consider the minimum value. It would also have a lower prior, which we'll see is overcome more efficiently by human testimony. Therefore a value of 1e8 for the kind of claims we care about is quite appropriate. That is a good estimate of the Bayes factor for a sincere, insistent, enduring, and life-changing personal testimony.
One red dot in a million
(new material)
One in a million events happen every month
This phenomenon goes by the name of "Littlewood's law": we start by assuming that a person witnesses an "event" every second. There are about 3 million seconds in a month, and humans are alert for roughly 1/3 of those seconds, resulting in a typical person witnessing about a million events per month. So then, it's expected that one of these events will have a prior probability of one in a million.
This has been used against the idea of the miracles - and certainly, if you have the rather simplistic view where you define a "miracle" as "an unlikely event", Littlewood's law would show that such "miracles" are not exceptional.
However, that misses a more important point, that reports of such event are often reliable. One need not be an outstandingly honest individual to make an "one-in-a-million" claim. One simply needs to report the most unlikely, exceptional thing that happened to them in the last month - which people do rather regularly. Even if you make a large concession and say that half of such reports are lies, that means this event's prior odds of 1e-6 turned into a posterior odds of 1e0, giving 1e6 as the Bayes factor for such a report.
In fact, applying the raw form of Littlewood's law to the resurrection testimonies actually gives a Bayes factor in excess of 1e8. For the disciples maintained their testimonies not for a month, but for their whole lives - a period lasting decades. Theirs was a claim about the most exceptional thing they had witnessed in that long period. Using the same argument as above, the most exceptional event that happened in the last 30 years is 360 times less likely than the corresponding event in a month, because that's how many months are in 30 years. Therefore, a report of such an event would have a Bayes factor of 3.6e8, even if you make the rather large concession that half of such reports are lies.
Perhaps a more reasonable set of assumptions would be that a report of a resurrection would need a minute's worth of events to be actually observed, rather than a second. This would correspond to a length of a short conversation, or an extended greeting. This means that "events" of this type last a minute rather than a second, which reduces the Bayes factor by 60. But reports about the most exceptional minute over the course of 30 years probably has better than even odds of being true - a posterior odds 1e1 seems appropriate. Making these adjustments, the Bayes factor turns out to be 0.6e8.
But at this point we're discussing minutiae. In the end, a personal claim which endures for 30 years has a Bayes factor of around 1e8. We can then add the "sincere, insistent, and life-changing" conditions on top of that, and that makes 1e8 a good, safe value to use as the Bayes factor for the type of testimony in Jesus's resurrection reports.
Video of a lottery winner
As one more confirmation of that 1e8 number, take a look at this video - it shows a woman's reaction to an acquaintance who claims to have won the lottery. Now, does that woman seem like a gullible idiot to you? I don't feel that way. She starts off quite skeptical, but not dismissively so. Then you can see the man's sincerity working on her - her degree of belief is clearly somewhere around even odds right before the numbers are confirmed. I think her overall reaction is pretty rational.
Now, there are some small differences between the video and the previous examples. For instance, she already knows that there's a winner out there, which increases the prior odds. But on the other hand, the woman's belief is achieved with little effort on the man's part, taking only minutes of insistence. The man being her acquaintance, and the fact that he comes up to her during the filming of this video, also increases the chances for something like a practical joke - which is an additional factor that his personal claim has to overcome.
On the whole, you can see her mind being pulled through a Bayes factor of something like 1e6 within mere minutes, in good accord with rationality, in a situation pretty similar to what we described in the previous examples. So 1e8 for something like the disciple's testimony about the resurrection is quite reasonable.
Summary of the findings
We began by examining our gut feelings on how much credit we would give to someone who made extraordinary claims, like having won the lottery or been struck by lightning. From this initial calculation, using just some intuition, we got a variety of numbers for the Bayes factor of a human testimony, ranging around 1e7 to 1e9. The number we ended up using, 1e8, started from these calculations.
That's a good start, but we wanted empirical backing. The first natural experiment we used to verify this number was the case of the people who lied about winning the 1.6 billion dollar Powerball lottery. The result from this calculation was about as good as it could possibly be expected; 1e8 really turned out to be the correct order of magnitude for the Bayes factor, when someone claimed that they had they had won the lottery.
We then considered how often people lie to us. We found that even weak, off-hand statements making rather unremarkable claims have a Bayes factor of at least 1e5. Adding the "sincere, insistent, and enduring" condition on top of that could easily bring this to around 1e8.
We then investigated the case of someone missing an appointment due to a car accident. The claim of a car accident on a specific day turned out to have a Bayes factor of 1e5 as a lower bound, while its true value was estimated to be around 1e7.
We next investigated the tragic story of a young woman dying in a car accident, and her mother committing suicide when she heard the news. The testimony of the person who related this story was calculated to have a Bayes factor of 1e8 as a lower bound, while its true value was estimated to be around 1e11.
For the claims of being in Harvard's physics PhD program, the Bayes factor was found to have a lower bound of 1e7 - of course, the most likely value would be higher. And for the case of people claiming to have lost a close loved one in the 9/11 attacks, the Bayes factor turned out to be about 1e6, despite the fact that there was cold, hard cash to be won as a strong temptation to lie.
Furthermore, we saw that making a claim with a Bayes factor of 1e8 doesn't require some extraordinary honesty or accuracy. Since Littlewood's law says that one in a million events happen every month, and one in 1e8 events then happen around every decade, merely reporting such events with mediocre accuracy gives you a Bayes factor of 1e6 or 1e8 respectively, even if such reports are wrong half the time.
Lastly, we checked this value on a gut level. We saw a video of a woman whose degree of belief changed by around 1e6, in a matter of minutes, by a man who claimed to have won the lottery. In the video, she was clearly rational and displayed the appropriate amount of skepticism, but in the end her decision to give the lottery winner the benefit of doubt turned out to be the correct one.
And here's one more double check, which we'll cover fully a few chapters later: consider all the personal testimonies about a resurrection made throughout world history, which pass the "sincere, insistent, enduring, and life-changing" condition. How many such testimonies are there? And what does that imply about the Bayes factor of such a testimony?
As we'll see, there are essentially no non-Christian figures who have had such a resurrection claim about them. There are many claims at the "some people say..." level, but none of them reach the level of a sincere, insistent, enduring, and life-changing personal claims of the kind we're looking for. Given that billions of people have died with no resurrection claims about them, this means that such a resurrection testimony is at least an one-in-a billion (1e9) event - and so it must be accorded a correspondingly large Bayes factor.
The strength of a human testimony is firmly established and understood.
It is important to note that all of these examples were merely the first ones that came to my mind which I could also get good numbers for. There is not a set of other examples which I chose not to use because they didn't suit my purpose. There is no selection bias here. In fact, I encourage you to come up with your own examples through which you can compute the Bayes factor of a human testimony. Compare your answer with mine, and independently verify my values.
It is also important to acknowledge that there is variance in the Bayes factors. That 1e8 is a typical value, and it will naturally change when we put conditions on it. For example, the relatively low value of 1e6 for people claiming to have lost loved ones in the 9/11 attack can be attributed to the explicit possibility of dishonest gain through fraud. On the other hand, the high value of 1e11 was obtained for a friend telling me an unlikely story, and its greater Bayes factor can perhaps be attributed to the credit of that friendship. It seems that such considerations can shift the Bayes factor by a couple orders of magnitude.
But now, having examined these multiple examples, the Bayes factor for the kind of testimony in Jesus's resurrection really is about 1e8, at least. This is borne out by multiple lines of thought, and verified by multiple cases of empirical inquiry. In certain circumstances - like when there is cold, hard cash on the table and there's a real opportunity for fraud - it can get as low as 1e6. Still, 1e8 is better as a typical value.
But, even with that lowball value of 1e6, the evidence for the resurrection is amply sufficient. With 1e6 as the Bayes factor, Peter and Paul's independent testimonies already gives a Bayes' factor of 1e12, which overcomes the prior odds of 1e-11. Then the rest of the testimonies in 1 Corinthians 15 drives the posterior odds for the resurrection far beyond any reasonable doubt. Meaning, EVEN IF you KNOW that the disciples had a good reason to be deceptive or delusional, there's STILL enough evidence in their weakened testimonies to conclude that Jesus did really rise from the dead. That's how strong the case for the resurrection is.
Chapter 5:
A deeper understanding of human testimony
Questions about human testimony
So, the Bayes factor for the kind of human testimony involved in Jesus's resurrection is about 1e8. This estimate is now firmly established.
However, you may still have some niggling doubts - maybe not about this number exactly, but about some other surrounding issues on which you feel a cloud of uncertainty. You may feel, for example, that 1e8 still somehow gives too much credit to human honesty. Or that 1e8 is too much at odds with the Bayes factor for a chess game record, of 1e120. Since both are a form of human testimony, you may worry that such a large difference points to a flaw somewhere. Or perhaps you're disturbed by how much the prior probability for an event seems to influence the Bayes factor for the testimony about that event. Or maybe, you're not sure how to stack multiple testimonies together. The math is easiest if the testimonies are independent - you just multiply the Bayes factors - but of course, this almost never happens in reality. So how do we take the dependency factors into account?
Answering these questions involves going into more depth about what makes up a human testimony - which we will now do.
The first step in a testimony: the inception of the idea
I'm thinking of a false statement right now. Can you guess what it is?
You almost certainly cannot. There are so many possible false statements out there - a functionally infinite number - that to be able to guess the specific one that I'm thinking of is basically impossible. For the record, I was thinking that I once had lunch on a planet that was 2.192 312 times older than the earth, which was orbiting the 82 234 344 436th most massive star in the Andromeda Galaxy. To be able to guess that specific statement would involve at least getting all the numbers right, meaning that the odds against just that numerical part of the guess are at the best 1e-18. There was essentially no way for that specific false statement to get into your head.
This illustrates an important point in determining the Bayes factor of a human claim. Whether it's a truth or a lie, the thought for the claim first has to somehow get into the human's head. Then afterwards, they may choose to make the claim or not. Each of these two steps are conditioned on whether the claim is true or false, and the overall Bayes factor for the claim will depend the combination of both steps.
Let's go through an example, of a chess game. Say that you watch the game and record it, and present the following as the game record:
1. e4 e5 2. f4 exf4 3. Bc4 Qh4+ 4. Kf1 b5 5. Bxb5 Nf6 6. Nf3 Qh6 7. d3 Nh5 8. Nh4 Qg5 9. Nf5 c6 10. g4 Nf6 11. Rg1 cxb5 12. h4 Qg6 13. h5 Qg5 14. Qf3 Ng8 15. Bxf4 Qf6 16. Nc3 Bc5 17. Nd5 Qxb2 18. Bd6 Bxg1 19. e5 Qxa1+ 20. Ke2 1-0
Now, if this record is in fact the truth, then how did it get into your head? Well, that's easy - it's the truth, you watched it happen, and you recorded it as it was happening. The probability this game record entering your head, if it really did happen this way, is near certainty.
But what if the game did not in fact happen this way? Well then - it's something of a mystery how you even thought to record this other, incorrect game. Why this specific game record, out of more than 1e120 possible untruthful chess games? The chances of this specific game record even entering into your head in the first place is at most 1e-120, if it were chosen at random.
Then the Bayes factor for the truthfulness of this game record is at least the ratio of the two numbers above - "near certainty" at 1, and 1e-120 - resulting in 1e120. This is just based on the fact that the record even entered into your mind at at all, and before you make any actual claims about whether the record is in fact true.
The "human honesty" step, and dependence factors in multiple testimonies
The next step in the process, after the game has first come into your mind, is to make the actual claim based on what's in your head. Because people are mostly honest, you are more likely to make the claim if it's the actual truth, and less likely to make the claim if it's false. This adds an additional Bayes factor for the truthfulness of the game record, but this factor is generally small - much smaller than 1e120. The exact value varies by individual of course, but something like 1e2 may serve as a guess here. In other words, people tell the truth about 99% of the time (as a guess), when the truth and the falsehoods are both present in their minds.
This explains why some may feel that numbers like 1e8 or 1e120 are somehow too large to be the Bayes factors for a human testimony. They're intrinsically thinking of something like 1e2 as the proper number, as the Bayes factor for a scenario where someone has both a truth and a lie as fully present alternatives in their minds. This may be the proper Bayes factor if someone merely gave a reply under direct questioning - as in something like "did you, or did you not, see the suspect at the scene of the crime?" It is the proper Bayes factor if someone flips a coin and tells you that it landed heads. But it is incorrect for the kind of voluntary, declarative claims made by the earliest Christians announcing Jesus's resurrection.
This also illustrates the effect of dependency factors in how multiple testimonies stack together. The first testimony, presenting new information, should be given a large Bayes factor like 1e8. A second testimony should be given something much smaller, like 1e2, if it's only answering the question of "Is the first testimony correct?" Further testimony offering only confirmation would get ever smaller Bayes factors, but a new, independent testimony would get the full value again.
The "stretchiness" of human testimony
Now, let's throw a twist into the example. Your game record above turns out to be identical to that of the Immortal Game - arguably the most famous chess game in history. What are we to make of that? How does it change the Bayes factor for that game record?
It drastically reduces it, of course. Recall that the enormous Bayes factor exceeding 1e120 came mostly from the first step, where we assumed that a specific incorrect game record had less than a 1e-120 chance of being selected randomly for getting into your head in the first place. Well, as a very famous chess game, the Immortal Game would not have been selected randomly. Even if that wasn't the actual game that was played, it could have entered into your head in a number of different ways, all of which are much more plausible than 1e-120. This precipitously drops in the Bayes factor. So, there's essentially no chance that your game record is correct, right?
Actually, this has far less impact on the final, posterior probability than you might guess. One may think, "Oh, so you're saying that a random chess game someone played just happened to be the exact replication of the most famous chess game ever? Give me a break!" But this was unlikely to be a random chess game, from the beginning. As one of the most famous games, the Immortal Game has a much, much higher chance of being played than a random, 1 out of 1e120 game. The game you witnessed may have been an exhibition match from a series of famous historical matches. Or you may have simply gone to an online chess site which replays famous games. Or the two players may have planned out the game beforehand as a demonstration, stunt, or a joke.
So, the Bayes factor of your game record becomes much smaller than 1e120, but the prior odds of the game you recorded being actually played becomes much greater than 1e-120. In fact, the two effects will cancel out to a significant degree. A moment's reflection reveals why: the same mechanism is responsible for both effects. A famous game is more likely to be replicated in actual play than a random game, and it's also more likely to falsely enter into your mind than a random game. The first effect increases the prior odds, and the second effect decreases the Bayes factor. But the effects have the same origin, and their magnitudes are therefore comparable. The degree to which a game is likely to be replicated is also the degree to which it may falsely enter into your mind. The net effect is that the final, posterior odds of the game being truthfully recorded doesn't change as much as you'd think. If you handed me the above game record and claimed that it was an actual, recent game between two people, I may lean towards believing it was real. I'm likely say "huh, it looks like these guys replicated the Immortal Game in their match", rather than "You're lying. You expect me to believe that their random play just happened to exactly replicate the Immortal Game?"
This explains the large swings in the Bayes factor of a human testimony depending on the circumstances, and why it depends so much on the prior odds of the event in question. If the event is intrinsically unlikely, it has low prior odds, but it's also unlikely to enter into your head in the first place, so the Bayes factor correspondingly increases.
So human testimony has this somewhat strange property, in that it may "stretch" to cover a great deal of very unlikely priors, even up to numbers like 1e120. The less likely the prior, the more the Bayes factor of the testimony stretches, so that the final, posterior odds is not as affected as you'd think.
The maximally unlikely, worst case scenario: when the testimony can't stretch
Now, given all this, what kind of claim would be the least likely to be true? You can't just have low prior odds - that only causes the Bayes factor to "stretch" as detailed above. To get around this, we need to consider a claim that's not merely unlikely, but also interesting or remarkable to the human mind in some way. We thus freeze the "stretching" of the testimony about the claim, because there is now a special, alternative way for the claimed event to enter your mind. We still want to have low prior odds for the claim, of course: the goal is to maximize the difference between the prior odds, and the likelihood of the idea entering your head.
In other words, the claimed event should be unlikely intrinsically, but likely to enter your head due to its remarkable, interesting nature. In the chess game example, we achieve this by claiming that completely random play by both players resulted in the replication of the Immortal Game. That is the claim with the minimal posterior odds. That is how we achieve maximum skepticism.
Here are some more examples of maximally unlikely claims, on which you would have maximal cause for skepticism: If the claim is about the lottery, you claim that you won the jackpot, rather than saying that your numbers came up in the drawing 3 months later. If the claim is about birthdays, you claim that you have the same birthday as your listener, rather than saying that it's some random date like October 23rd. If the claim is about being struck by lightning, you claim that you got struck, rather than saying that your uncle once had a close call. If the claim is about a car accident, you claim that you were in that accident today, rather than saying that an accident happened at the same place a month ago. And if the claim is about your school and major, you say that you're in the physics PhD program at Harvard, rather than saying that you're going for your masters in civil engineering from Ohio State.
You will recognize these as the examples that we've studied earlier, from which we obtained the value of 1e8 as the Bayes factor of a human testimony. In other words, that 1e8 value was calculated precisely for these "maximally skeptical" scenarios, where someone makes an extraordinary claim about an unlikely event, and the Bayes factor is used mostly to overcome the small prior. And even under these conditions, the resurrection was found to be highly likely.
So there is no escaping that value: the Bayes factor for an extraordinary claim about an unlikely event really is about 1e8. This was calculated from the same kind of "maximally skeptical" scenarios as the resurrection. All of our previous calculations have been for precisely claims of this type. The value from such calculations is therefore fully applicable to the testimonies concerning Jesus's resurrection.
The resurrection story revisited, with dependence factors
We now have all necessary components to understand a scenario involving multiple pieces of evidence.
Let's say that someone testifies to a rather unlikely event - say, Peter testifies that "Christ is risen". That testimony has a Bayes factor of 1e8, against a prior of 1e-11. That brings the posterior odds to 1e-3. You should not yet assent to Peter's claim.
So, being skeptical, you turn to John, who is Peter's friend and compatriot. You ask him, "hey, is Peter telling the truth?" and he answers "yes". Now, because John's testimony here is not independent of Peter's, it should not get the full 1e8 as its Bayes factor. Something like 1e2 is more appropriate. That brings the posterior to 1e-1 - still not quite enough for you to assent to the resurrection, but certainly close enough that the remaining testimonies in 1 Corinthians 15 would easily and amply make up for the difference.
But now, let's go back to the scenario as we previously covered it: while you're considering Peter and John's testimonies, Paul - nearly the last person you'd expect to agree with the other two - randomly bursts into the room and says, "Hey guys! Christ is risen!" What is the Bayes factor for that testimony? Because of the large degree of independence, Paul's testimony should get a large portion of the full 1e8 - easily overpowering the remaining 1e-1 odds, and fully shifting the posterior odds to be much greater than 1.
Paul's testimony, with full dependence factor
Do you doubt that Paul's testimony is enough? Then consider the following: Taking the full dependence factor into account, the Bayes' factor of Paul's testimony is, by definition, given by the following:
P(Paul|John, Peter, Resurrection) / P(Paul|John, Peter, ~Resurrection)
Where "Paul", "John", and "Peter" stand for each of their respective testimonies, and "Resurrection" or "~Resurrection" is our hypothesis in question, whether the resurrection happened or not.
Now, as ever, let us approach this empirically. P(Paul|John, Peter, Resurrection) is not all that unlikely. This is the probability of an opponent of Christianity giving a miraculous conversion testimony. Even apart from Apostle Paul himself, stories like this are old hat. You can't be a Christian for very long without tripping across a load of them.
Then what about P(Paul|John, Peter, ~Resurrection)? This is like the probability of a Paul-like miraculous conversion to your opponent's religion, DESPITE the fact that the religion is false. To get at this number, we only need to pick a religion that both you and I agree is false. Islam or Hinduism will do nicely, as they're mutually exclusive world religions: everyone can pick at least one of those as their "false" example. So, how many Paul-like miraculous conversion stories are there to that religion?
I have not heard of a single case. But it's not just me - I don't think even GOOGLE has heard of a single case. Google search will return different results for different people at different places and time, but my experiences on this front are still telling.
When I searched for "conversion stories to Islam", I got many cases of exactly what I searched for - conversion stories to Islam. So it's not as if Google has some anti-Muslim or pro-western agenda which prevents them from showing Islam-positive search results, nor is there a shortage of conversion stories to Islam on the internet. This is not surprising - the internet is a big place and Google is good at what it does.
But, when I searched for "miraculous conversion stories to Islam", the majority of the results, including the top result, was for Muslims converting TO CHRISTIANITY. And of the few results which actually described conversions to Islam, I could not find one which actually claimed to be miraculous in nature. Most of them are only of the "Islam made sense after I studied it" type. And none of them involved anyone who was as rabidly anti-Islam as Paul was anti-Christian.
Do you understand how remarkable this is? Even Google couldn't find me a single example of a miraculous, Paul-like conversion story to Islam, and when asked to do so it actually returned mostly conversion stories FROM Islam TO Christianity, despite "Christianity" not being in the query at all. That should give you an idea for the relative prevalence of such stories. It's reflective of the absolute dominance that P(Paul|John, Peter, Resurrection) has over P(Paul|John, Peter, ~Resurrection).
In fact, from this experiment, we can see that the magnitude of this dominance - that is, the Bayes factor of Paul's testimony - is in the same ballpark as the Bayes factor of a Google search itself, which is worth many orders of magnitude. It easily and greatly outpaces the numbers like 1e1.
Searching for "miraculous conversion stories to Hinduism" gave me mostly similar results. Nearly the entire first page is about Hindus converting to Christianity.
So, here is the challenge: do you believe that Paul's testimony is not enough? That it can't cover the remaining 1e-1 odds? Then you need to be able to back that up empirically. You need to give me one instance of a miraculous conversion to Hinduism or Islam by a former opponent, for every 10 instances of a miraculous conversion to Christianity that I could cite. Good luck with that, given that even explicit Google searches for such conversion stories return far more cases supporting the Christian position.
Back to the resurrection story
So, after hearing Paul's testimony, and given its large degree of independence, you assign it a Bayes factor fairly close to the full 1e8 value. This fully overcomes the remaining prior, and pushes the posterior odds firmly to the "for the resurrection" position. You should now firmly believe that Jesus did rise from the dead.
And as if that wasn't enough, you then encounter a flood of people all claiming that Jesus rose from the dead - the remaining members of the twelve apostles and other disciples, James, and a crowd of more than 500 people, just to name the remaining witnesses in 1 Corinthians 15. After considering all of their testimony, there claim is now beyond the shadow of any doubt: Jesus Christ almost certainly rose from the dead.
Human testimonies stretch to cover the rest of the Bible
Ah - but what about the other miracles in Christianity? Sure, the resurrection might be well-attested, but what about the numerous other miracles in the Bible which has barely any evidence behind it? For example, only Matthew mentions the resurrection of other people at the time of Jesus's death. He only mentions it briefly, in passing. Many of the remarkable miracles during the Exodus are also mentioned only in that book. The Bayes factor of a single testimony can't possibly cover all of these other stories. How could a Christian believe in such things, if such evidence is inadequate according to our methodology?
This is where the "stretchiness" of human testimony comes into play. Recall that a human testimony can have absurdly high Bayes factors exceeding 1e120, like when you choose to believe a particular chess game record. Once we're freed from the constraint that we must only consider the maximally unlikely scenario, where the most extraordinary claim is made about the most unlikely event, the Bayes factor is no longer restricted to just 1e8. It can "stretch" to cover nearly any prior, as long as it doesn't have some special, exceptional way of entering into your head in the first place that allows it to outpace the prior.
So, could Jesus's resurrection have been accompanied by the resurrection of other people, as Matthew testifies? Well, sure - the prior is low, but there is no special reason for Matthew to have thought up that story. You may argue that it is an interesting enough story that Matthew may have made it up after learning that Jesus rose from the dead, but the prior for something like that is also increased given that Jesus rose from the dead. In fact, "given that Jesus rose from the dead" is the common factor in both increasing the prior and making the story interesting, so they cancel out to a large extent, the "stretchiness" of human testimony kicks in, and the posterior odds remain reasonably high. This is exactly analogous to the mechanism through which a chess record of the Immortal Game has a reasonable odds of actually having been played.
So then, once you accept the resurrection, all sorts of ancillary claims get a Bayes factor which stretches far beyond 1e8 to meet their prior. It makes sense that if Jesus really rose from the dead, he'd also be able to heal the sick. It makes sense that his resurrection would be accompanied by other remarkable events. And such a person is probably trustworthy when they vouch for the miraculous stories in Exodus. That is how all other other miracles in the Bible can be believed, once you accept Christ.
Going back to the chess analogy, this is like being given a second game record after the Immortal Game record, where the two players supposedly played through the Game of the Century this time. If you have sufficient evidence to believe that the two players really played through the Immortal game in their first game, you have no real reason to doubt this second game record.
A fuller understanding of human testimonies validates our previous calculations
So, what have we achieved in this deeper dive into human testimonies?
We've seen that, while there is in fact high variability in the strength of a human testimony, our value of 1e8 for their Bayes factor was already calculated for the maximally pro-skeptical scenario - where the most remarkable, noteworthy claim is being made about a highly unlikely event. This was most fitting scenario for the resurrection testimonies, which gave the lowest posterior odds. And yet, even under these conditions the resurrection proved overwhelmingly likely.
Along the way, we've explained why this 1e8 might mistakenly feel too large, if you ignore the priors that human testimonies have to overcome. For instance, a human testimony can have a small Bayes factor like 1e2 - if it's about an event which is already quite probable, like a coin flip or a yes/no question. Conversely, since the resurrection is a highly improbable event, testimonies concerning it should be given a higher value than our other examples.
We've also explained how human testimonies may "stretch" to cover absolutely minuscule priors (as in the record of a chess game), as long as there was no special way for a particular falsehood to enter into your mind. Incidentally, this gave us a nice bonus, in that it justifies our belief in the other, non-resurrection miracles in the Bible. Yes, these miracles have low priors on their own. But once we accept Jesus, the testimonies about them can easily "stretch" to cover their priors, as such miracles cease to be the most remarkable, special thing that can happen in a world where Jesus rose from the dead.
Putting all this together, we repeated our earlier calculation for the odds of the resurrection, this time taking the dependence factor between Peter and Paul fully into account. We saw that the strength of just their two testimonies, even with full dependence factors, was easily enough to put the odds firmly in the "likely" side. The rest of the testimonies in 1 Corinthians 15 then puts the resurrection beyond any reasonable doubt.
So, we now have a good idea of how a human testimony comes together. We understand its "anatomy" and its behavior. And this deeper dive into human testimonies made sense of our intuitions, verified our earlier thinking, and validated our previous conclusion.
Chapter 5:
A deeper understanding of human testimony
Can naturalistic explanations account for the resurrection testimonies?
So, the previous Bayesian analysis compels us to believe that Jesus really rose from the dead. But, as an additional layer of verification, let's approach the problem from a different angle, and see if we come to the same conclusion.
In our analysis, the odds for Jesus's resurrection went from a prior value of 1e-11 to a posterior value far greater than 1 - meaning, the Bayes factor for the testimonies in 1 Corinthians 15 was well in excess of 1e11. Another way of stating that is to say that the evidence of those testimonies is, at the very least, 1e11 times better explained by an actual resurrection than by naturalistic alternatives.
Now, if you want to cling to a naturalistic alternative, you must believe that this Bayes factor value is incorrect. That it is not really that large. That the true value is insufficient to overcome the small prior odds. That a naturalistic alternative can sufficiently explain the evidence, so as to make an actual resurrection unnecessary.
Well, can you demonstrate that empirically?
If naturalism can sufficiently explain the evidence for Jesus's resurrection, I expect there to be some non-resurrection cases where the same level of evidence was achieved through ordinary means - through naturalistic chance, as it were. It would be a strange naturalistic explanation indeed that works only once for the specific case that we're trying to explain, and never works again.
Here's what I mean: let's say that you think the resurrection testimonies are totally worthless and changes nothing about the probability that Jesus rose from the dead. This would correspond to a Bayes factor of 1 (or 1e0), meaning that a non-resurrection is equally likely to produce these testimonies as a genuine resurrection.
As a conservative estimate, let us say that there have been 1e9 reportable, non-Christian, naturalistic deaths throughout world history. Then, a Bayes factor of 1 would correspond to saying that all 1e9 of those people were as likely as Jesus to produce a resurrection story, each as well-evidenced as Christ's resurrection. Then there ought to be literally a billion of such cases. Well, where are they? Can you produce these resurrection reports?
Say that you're willing to be slightly more reasonable: you think the Bayes factor for the Jesus's resurrection testimonies is 1e6 - far smaller than 1e11, but still significantly greater than 1. Effectively, you believe that the testimonies clearly do count as evidence, but that it's just not enough to overcome the prior. Well, a Bayes factor of 1e6 corresponds to saying that a non-resurrection still has one millionth the chance of producing New-Testament level of testimonies compared to a genuine resurrection. Again, with those odds, given that there have been at least 1e9 people whose deaths were reported throughout human history, this means that you should still be able to produce more than a thousand of accounts of someone rising from the dead, each with as much evidence as the New Testament has for Jesus's resurrection.
You can easily do the same calculation for a Bayes factor of 1e9. Following the examples above, if you think that the Bayes factor is only that large, then you should still be able to find at least one other case where a non-Christian, natural death produced the same, New Testament level of evidence as Jesus's resurrection.
Validating even larger Bayes factors with historical records
Ah, but what if you believe, as I do, that the Bayes factor is far larger? That Peter's testimony by itself already has a Bayes factor of at least 1e8, and that Peter and Paul's testimonies together has a Bayes factor well over 1e11?
Well, Peter's testimony by itself is pretty clear. If its Bayes factor really is 1e8, I should be able to find something like 10 resurrection stories throughout history which have a level of evidence matching Peter's testimony. If the Bayes factor is actually smaller, I'll be able to find more such stories. If the Bayes factor is greater, then I'll be able to find less.
For Peter and Paul's testimonies together, if the Bayes factor there is really well over 1e11, then I won't be able to find a single resurrection story with enough evidence to match their testimony. In fact, since 1e11 is 100 times larger than 1e9, this would require observing less than one of something. How does that work out?
While we can't observe less than one instance of something, we can observe how close the closest instance comes. Let's work through our numbers: 1e11 is 1e9 raised to the 11/9th power. So, if 1e11 is the Bayes factor for Peter and Paul's testimonies, You'd expect the nearest case of a non-Christian resurrection story to have about 9/11th of the evidence of Peter and Paul's testimonies combined. In other words, it would come pretty close to matching Peter and Paul's combined testimonies. So this hypothetical non-Christian resurrection story would perhaps have the evidence of two fully committed personal testimonies, but the two might only be strangers instead of mortal enemies.
If you assume that Peter and Paul's testimonies are almost completely independent, as I have in my calculation at the beginning, their combined testimonies gives a Bayes factor of 1e16. How could we verify that? Again, we look to the historical record. If that 1e16 is correct, then throughout the 1e9 reportable deaths in history we would expect a resurrection report with a maximum evidence reaching 9/16th of the level of Peter and Paul's combined testimonies. 9/16 is a little more than 50%, so that might correspond to one exceptionally well-documented individual testifying to the resurrection. If that is what we find, our value of 1e16 is validated. If we find less than that, that means Peter and Paul's combined testimonies may have an even larger Bayes factor.
Think of the process in this way: say that there's a record of a million coin flips. While examining that record, I come across a sequence of 10 heads in a row, and say "Wow, that's amazing! These coin flips couldn't have been random!" Now, if you wanted to debunk me by showing that random chance can easily produce such sequences, you can say "Actually, the chances of getting 10 heads in a row randomly is only 1 / 2^10, or about 1e-3. The Bayes factor of your sequence for your hypothesis is therefore only 1e3. In a million coin flips, you'd expect to see something like this about a thousand times". You can then proceed to point out those thousand other "10-heads-in-a-row" sequences in the coin flip record, and that would validate your Bayes factor estimation.
However, let's say that I then come across a sequence of 60 heads in a row. I say again, "Wow, that's amazing! These coin flips are clearly non-random! I think the chances of a sequence like this is 1 in 1e18". How could I empirically prove that my estimate is correct, when the probability is so small? Wouldn't I naturally expect zero such "60-heads-in-a-row" sequences from a million flips?
It's simple. Just find the sequence with the longest chain of heads in the coin flip record. In a million flips, you'll probably see a maximum sequence with about 20 heads in a row, which has about a one in a million chance to occur. This means that a 40-head sequence will happen once in a million-squared coin flips, and a 60-heads-in-a-row will happen once in a million-cubed (or 1e18) coin flips. Thus, by verifying that the longest sequence of heads has about 20 head in it, I also verify that the chances of 60 heads in a row is about 1e18. So even when the Bayes factor is extremely large for a very strong piece of evidence, you can still get an estimate for that Bayes factor by seeing what fraction of that evidence is duplicated by chance in the population at large.
But what about dependence factors?
Dependence factors complicate this example, but changes nothing about the fundamental idea. Let's say that each heads in the sequence increases the chance of the next coin being heads. This simulates how one person's testimony increases the chance of another person giving an agreeing testimony, due to dependence factors. Let's furthermore say that the exact functional form of this increase depends on myriads of factors in a complicated way. What now? Do these complications make our previous plan for validation intractable?
Not really. Our overall program still works. The Bayes factor for a testimony can still be measured from the frequency of such testimonies in history. That's the beauty of working from empirical, historical records: you can skip all the math and theorycrafting, and just read off the answer that reality computed for you.
Even when the testimony in question is so strong that its suspected Bayes factor far exceeds the number of available historical records, we can still follow our program and get an estimate. Just compare the testimony with the closest thing that happened in history, and note the difference. This is enough to validate qualitative statements like "far exceeds 1e11". If we're willing to do a little more math and actually model the distribution of the empirical historical testimonies, we can even get a quantitative answer. In fact we will do exactly that, later in this work.
So, it basically comes down to this: you think that the evidence for the resurrection isn't good enough? Well then, start citing other, non-Christian examples in history where someone comes back from the dead. We'll see how the best of these measure up against the evidence for Christ's resurrection, and then see how the Bayes factor calculated this way compares to our previously calculated value.
The conditions: the requirements for "matching" a testimony.
Before we begin diving into specific examples of other resurrection stories in history, let us establish the comparison criteria: what would it take to match the level of evidence in Christ's resurrection story?
Recall that we're using the testimonies enumerated in 1 Corinthians 15. This passage identifies six specific individuals or groups who personally testified to Christ's resurrection. They are Peter, James, Paul, "the twelve", "the other apostles", and "the 500".
The plan is to compare these testimonies against the level of evidence we find in world history through a naturalistic process and outlook. We want to see if any such naturalistically generated resurrection stories can match or even approach the level of evidence in Christ's resurrection.
What would it take to "match" one of the 1 Corinthians 15 testimonies? Well, the idea here is that the overall quality of the evidence should be on par with the evidence we have from the testimonies mentioned in 1 Corinthians 15. So:
To match Peter, James, or Paul's testimonies, we will require a sincere, insistent, and enduring personal testimony by a single named individual. They must have been a public figure whose entire life (choice of profession, place of residence, etc.) was lived in complete alignment with that testimony. History must be able to locate this person with great precision, and have a good amount of information available about them.
To match the testimonies of "the twelve", we will require a sincere, insistent, and enduring personal testimony by a group of about a dozen named individuals. They must have been public figures whose entire lives were lived in complete alignment with that testimony. History must be able to locate these people with good precision, and have a good amount of information on at least some of them.
To match the testimonies of "the other apostles", we will require a sincere and enduring personal testimony by a group of individuals. At least some of them must be named. At least some of them must have been public figures on the matter of their testimony. History must be able to locate these people with good precision, but not a lot of historical data is required of them.
To match the testimonies of "the 500", we will require a sincere personal testimony by a large group of people. They need not be named, or be public figures, or endure in their testimony, or have any additional information known about them. But history must be able to locate these people precisely enough, so that at least some of them could be theoretically pointed out by a well-known figure like Apostle Paul.
We can, of course, extrapolate from this set of matching testimonies. The above list should be comprehensive enough that it can serve as a metric for measuring most of the personal testimonies in history.
So, here's the program now: we will search through world history, and examine the best non-Christian reports of a resurrection. We will examine the level of evidence behind each of them, and measure them up against one of the matching categories in the list above. We will also measure their evidence against the total evidence for Jesus's resurrection. Doing so will allow us to validate our earlier estimation of an individual's Bayes' factor, and the total amount of evidence for Jesus's resurrection.
What are we expecting? Which results would vindicate which hypothesis?
As previously stated, let us conservatively say that there have been 1e9 reportable, naturalistic deaths throughout world history. So a resurrection report with a Bayes factor of 1e8 would have 10 similar, matching cases in world history. A better resurrection report, with a higher Bayes factor of 1e9, would be rarer and therefore be the only case of its kind. A weak resurrection report with a Bayes factor of 1e6 would have 1000 similar cases, and so on and so forth.
I evaluated Peter's testimony by itself at a Bayes factor of 1e8, as a conservative estimate. Therefore, I expect there to be less than ten resurrection reports with a level of evidence matching Peter's (or Paul's or James's) testimony. This will validate our earlier evaluation of an individual's testimony, as having a Bayes factor in excess of 1e8.
I evaluated Peter and Paul's testimonies together (including their anti-dependent nature) to have a Bayes factor safely above 1e11. Therefore, I expect that no case in naturalistic world history will match their testimonies. Even the nearest approach would fall distinctively short.
Recall that adding more evidence multiplies the Bayes factors: therefore doubling the evidence squares the Bayes factors. So, it doesn't take much evidence for the Bayes factor to go from 1e9 to 1e11. A little bit of evidence goes a long way. In terms of Peter and Paul, this means that the nearest approach by a resurrection report in naturalistic world history would amount to something like half of their amount of evidence, and that would be sufficient to put Peter and Paul's Bayes factor safely above 1e11.
As for the total evidence for Christ's resurrection, I evaluated that total Bayes factor to far exceed 1e11. The net amount of evidence summarized in 1 Corinthians 15 is multiple times that of just Peter and Paul's. Therefore, we would expect that there is not a single other case which comes even remotely close to matching the level of evidence for Christ's resurrection. The nearest approach by a historical, naturalistic death would fall far short of that level - not just by 30% or 50%, but by a sizable multiplicative factor.
Ah - but when we evaluate against the total evidence for Christ's resurrection, how should we weigh the six different testimonies in 1 Corinthians 15? What type of testimony is worth more? How should we evaluate a non-Christian resurrection report, if it has an individual testimony like Peter's, and a group testimony like "the 500"'s?
No matter - I will allow matching any of the six testimonies as counting for 1/6th of the total evidence. Essentially, I'm pretending that each of the six testimonies in 1 Corinthians 15 have equal value. This is wrong, but it's wrong in a direction that handicaps against the Christian position. What will happen is that the easiest of the six testimonies to match - which is worth less than 1/6th - would be more likely to show up in the historical record, and yet they will be given the full weight of 1/6th. This is an important point: any errors in the evidence-level assignment due to our metric can only be favorable to the skeptic's case, so the pro-Christian conclusions we come to will have an extra margin of assurance.
So now we know what observations would validate our previous calculations. Making those observations will show that an individual testimony like Peter, James, or Paul's is worth a Bayes factor of at least 1e8, that Peter and Pauls testimonies together (including their anti-dependence factor) is worth well above 1e11, and that the sum total of evidence for Christ's resurrection is far in excess of 1e11.
Now we're finally ready to look at the non-Christian historical records. Let's start.
The other historical records:
Fortunately, skeptics of Christ's resurrection have done some of the early leg work for us, in that they compile lists of purported people who have been said to be like Christ for one reason or another. These include a number of people who are said to have been raised from the dead. Let us look at a representative sample from such lists.
Apollonius of Tyana:
Apollonius of Tyana is sometimes compared to Christ because they were both philosopher/preachers in first century Rome, to whom miraculous powers are attributed. Wikipedia has a list of similarities between Jesus and Apollonius, which includes a wondrous birth, the ability to heal the sick and raise the dead, a condemnation by Rome, and an ascension into heaven. That sounds pretty similar, no? So how does the evidence for Apollonius's "resurrection" hold up?
Pathetically. Most of the information on Apollonius comes from Philostratus, who was paid to write a biography of Apollonius well over a hundred years after Apollonius's death, and after Christianity was already a thing. This biography only "implies" that Apollonius underwent heavenly assumption. Furthermore, the chief primary source for this biography is one Damis, a disciple of Apollonius, who is unknown outside of this biography. And to top it off, Philostratus specifically writes that Damis had not recorded anything about Apollonius's death. The stories of his death and supposed heavenly assumption are in a part of the biography that are filled with 'some say this, some say that' stories, which, by the author's own admission, he wrote because he felt that his story needed to have a natural ending.
So, the evidence for Apollonius's "resurrection" comes down to one author, who wrote more than a hundred years after the event, who says that he's getting his information second-hand from a Damis that nobody else has heard of, who then explicitly says that the "resurrection" bit - which is only implied - doesn't even come from Damis.
Compare that to the evidence for Christ's resurrection, in the form of the testimony of his disciples. 1 Corinthians 15 was written within a couple decades of the event, and it contains a creed that was formulated mere years after the resurrection. We have the personal, first-hand testimonies of the people who have seen the risen Christ. They clearly say that it really happened, and that it transformed their own lives. Each of these disciples appear in multiple other sources, and bear the same witness about Christ's resurrection in those sources.
So... now we're suppose to compare the strength of the evidence between these two? Well, let's see. Remember our previous criteria, about what it would take to "match" 1/6th of the evidence in 1 Corinthians 15. Can we say that maybe Damis's testimony about Apollonius's resurrection matches the testimony of Peter, James, or Paul? Well, no. Damis never even made that testimony, nor is he anything like those three individuals on the quality of historical information we have on him. So then, all that's left as evidence is "some say that Apollonius rose from the dead", stated more than a hundred years after the fact?
That is essentially no evidence. But since I have to give a numerical estimate, I would be generous and say that Damis's "testimony" counts as an order of magnitude less than that of Peter, James, or Paul. I will also generously grant the "some say..." part of the story as being a order of magnitude less than that of the 500 witnesses that Paul mentions. So, that comes to:
1/6 (matching a single element in 1 Corinthians 15)
× 1/10 (an order of magnitude less)
× 2 (two such instances),
= about 1/30th of the evidence that we have for Christ's resurrection.
Zalmoxis:
Next, let us consider Zalmoxis, whom Herodotus writes about in his "Histories" as a divinity in the religion of the Getae. Herodotus wrote that Zalmoxis's followers believed they have a form of immortality in him, and performed a kind of human sacrifice to communicate with him through death.
According to Herodotus, he was told by certain non-Getae peoples that Zalmoxis was really a man - that he was teaching his countrymen some philosophy, but then hid himself in a secret underground housing for three years while people thought he was dead. He then came back out and showed himself alive, and this caused the people to believe his teachings.
And... that's it. That's the substance of this Zalmoxis and his "resurrection". I'm not even summarizing that much - the text in Herodotus is hardly longer than the above paragraph. Apparently this is one of the best examples that the world can come up with when asked about non-Christian resurrection stories. And yes, some people really have tried to link this "resurrection" to Jesus's resurrection, in an attempt at discrediting Christianity. This, in spite of the record having no witnesses testimonies of any kind, nor even a group of people who can clearly be said to believe that someone came back from the dead.
Again, using the metric derived from 1 Corinthians 15, how does this measure up against the evidence for Christ's resurrection? Is anything about Zalmoxis's "resurrection" comparable with the testimonies of Peter, James, or Paul? Well, no. Zalmoxis has no witness testimonies, period - let alone any named witnesses among historically known persons. This means that nothing about Zalmoxis is comparable to the testimony of the apostles as a group, either. At the end of the day, all the evidence for Zalmoxis's "resurrection" comes down to "some people might have said that a god, who might have been a real person, might have come back from the dead". Note that all the "might have"s in that sentence are part of the historical evidence. It is not an external skeptic injecting doubt into the story, it's actually how the story is handed down to us through history.
So... I would again say this is pretty much no evidence. But again, because we need a quantitative value, I will be generous and say that this is an order of magnitude less than the evidence of the 500 witnesses in 1 Corinthians 15. That gives Zalmoxis 1/6 * 1/10 = 1/60th of the evidence that we have for Christ's resurrection.
Aristeas:
Let's next look at Aristeas, who is another character in Herodotus's "Histories". He is said to have been a poet. The "Histories" relate how Aristeas "suddenly dropt down dead" one day (in front of just one witness), but then his body could not be found and he was seen alive - once close to the time of his death, and then seven years later, when he appeared in another town and wrote a poem.
Here's the thing about this story: it was already at least 240 years old when Herodotus was telling it. Then, Herodotus says that some people say that Aristeas appeared again (as a "ghost" or an "apparition") after those 240 years, and instructed these people to build an altar to Apollo and a statue of Aristeas himself.
Again, that's about it. The whole story only takes up a couple of paragraphs in Herodotus's "Histories". Now, it's not quite clear that a "resurrection" had taken place - the first part of the story sounds more like a fainting or a disappearance, and the second one is called a "ghost" or an "apparition" by the people who were suppose to have seen it, who presumably had no means of personally identifying Aristeas. But let's ignore that for now. What kind of evidence - what kind of witness testimony - do we have for this story, and how does it compare to the story of Christ's resurrection?
Well, once again we have no named witnesses. The first part of the story is at least 240 years old at the time of the telling - so no witnesses, of any kind, are even possible. The second part, where a ghost or an apparition instructs people to build an altar and a statue, may be a bit more credible. We seem to at least have a group of people who were instructed to build a specific altar and statue, and Herodotus might have conceivably met the individuals who claimed to have personally received these instructions. On the other hand, they are never identified more specifically than "the people of Metapontion", and it's unclear whether this is simply a story that the Metapontines told about their altar and statue. Furthermore, it's not even clear how long ago this was supposed to have happened - the story about the apparition might as well have happened another 240 years ago from the time that Herodotus relates the story, judging from the scant details.
So, once again the testimony evidence here only turns out to be of the "some people say..." kind. The closest thing we can relate this to is the testimony of the 500 witnesses in 1 Corinthians 15. Given the rather shadowy nature of the "apparition", and the uncertainty about whether Herodotus has any specific primary witnesses in mind, I would generously say that this counts for maybe a fourth of the evidence of the 500 witnesses. So, Aristeas's "resurrection" has about 1/6 * 1/4 = 1/24th of the evidence we have for Christ's resurrection.
Mithra:
How about we look at some ancient gods? Jesus is often compared to the gods in other religions, but can any of them actually serve in our comparison of historical evidence for a resurrection?
Mithra, for instance, is a god in the Persian religion of Zoroastrianism, who then inspired a Roman mystery religion. He often appears on lists of gods that Jesus was supposed to have been copied from. But... um... it seems that he was never actually said to have been a human, or any kind of a historical figure, in either the Persian or the Roman variants. He doesn't even die, let alone rise from the dead, even in his mythologies. Furthermore, any specific details or even general plot points is notoriously difficult to extract from any Mithra mythology. The Roman version of Mithra was worshiped in a mystery religion, and none of their written narratives or theology survive - we only have some iconography to glean what we can of this Mithra. In the Persian version, Mithra is mentioned in some hymns (Yashts), which are again very short on details, mythology, or narrative. In all cases, he is always presented as a mythic entity, and the scant stories about him are always framed in that context.
So, on his comparison with Jesus, Mithra fails on this crucial point of historical existence. For our purposes, this also means that we can safely say that there is no evidence for Mithra's resurrection. Indeed such a claim is never even made, or even dreamt of - someone would first have to claim that Mithra was a historical figure.
Horus and Osiris:
Horus, an ancient Egyptian god, along with his father Osiris, are some more gods who are sometimes compared to Jesus - and they, too, fail the "historical existence" test. As with Mithra, all of the stories concerning these gods take place on a purely mythological level, and there are no claims to them having been a real, historical figure. For our purposes, it's clear that their story presents no evidence for a historical resurrection. But at least Osiris has a mythological story where he comes back after being murdered, and there is a story where Horus, as a child, recovers from a fatal scorpion sting. Of course, it's not even clear that there was ever a group of people who might have claimed to have been historical witnesses to these events - all ancient sources (Pyramid Texts, Palermo Stone, Metternich Stela, etc.) which mention this story always present it something that took place a long time ago, in an mythic age.
So, in assigning a level of evidence to this, we'll be extremely generous and again count this as an order of magnitude less than the evidence of the 500 witnesses in 1 Corinthians 15. Recall that this comes to 1/6 * 1/10 = 1/60th of the evidence that we have for Christ's resurrection.
Dionysus:
Dionysus is another god, this time from the Greek pantheon, who is superficially compared to Jesus but fails the "historical existence" test. Yes, there is a mythological story where he is killed as an infant then re-incubated in Zeus's thigh - but none of the sources that mention this mythology pretends to be history. Dionysus's situation with regards to his "resurrection" is therefore similar to that of Osiris or Horus - there is virtually no historical evidence for his "resurrection".
As with Osiris, we'll again be extremely generous and rate him as having 1/60th of the evidence for Christ's resurrection.
Krishna:
We now come to Hinduism's Krishna, who's another god that's sometimes compared with Jesus. He's said to be have been the incarnation of Vishnu, who is either the supreme god, or one of three or five most important gods, depending on the specific tradition in Hinduism.
Krishna has perhaps a greater claim to a real, historical substance compared to the other gods we've covered. For starters, he is at least said to have been born as a human. He is said to have gotten married and ruled kingdoms and fought battles. There is a great deal that is said about Krishna - but we are, of course, primarily interested in the story of his death and "resurrection".
The main literary sources we have on this part of Krishna's life are the Mahabharata and the Srimad Bhagavatam. They tell the story of how Krishna, at the end of a long and eventful life, intended to leave the world. He was then shot by a hunter named Jara, with an arrow through the foot. This marked the end of Krishna's life, for thereafter he immediately ascended to go to his own abode, leaving earth.
So, what are we to make of this "resurrection" story? What kind of evidence is there for it? Let us first try to establish the setting. These stories take place in ancient India, and Krishna is proposed to have lived some time between 3200 and 3100 BC, although there are some wildly differing estimates. These are quite large uncertainties, from a very long time ago - right at the edge of pre-history. These issues, by themselves, might not cause too much concern - until we attempt to date the writing of the Mahabharata, which contains these stories.
Dating the Mahabharata is tricky - it is a massive work, composed of multiple layers. Current scholarship estimates that the oldest layers are from around 400 BC, and the origin of the stories within it can perhaps be extended back to 1000 BC. In other words, the stories of Krishna were, at best, already thousands of years old at the time that they were recorded. Therefore, no personal, firsthand testimony to Krishna's death and ascension are possible in this work.
Okay - but what if we ignore the scholarship, and and go with the Hindu tradition which says that the Mahabharata was authored by the legendary sage Vyasa? Unfortunately, this doesn't help things at all. We know little about a historical Vyasa. When did he live? When did he write? We can no more anchor him in history than we can Krishna.
Complicating matters further is the story structure of the Mahabharata. You see, the death and ascension of Krishna is not just told as a story; it is framed as a story being told by Vaisampayana (a student of Vyasa) to the king Janamejaya (supposedly a great-grandson of a character in the Mahabharata), many years after the fact. But that's not the end of it - this story is further framed as a story being told by Ugrasrava Sauti, even more years later. So, the story of Krishna's ascension is a story (about Krishna), within a story (being told by Vaisampayana), within a story (being told by Ugrasrava Sauti), within a work (the Mahabharata itself, which was presumably written down some time afterwards). All this "story-within-a-story" structure sounds like a device for saying "once upon a time...", and makes the story sound like something told about "a friend of a friend". But let us ignore that for now. Even if we were to take the Mahabharata entirely at face value - an outlandishly generous acquiescence - we would still be forced to conclude that this story was already incredibly old at the time of the recording, and its content disqualifies itself from being considered a primary account, due to its story-within-a-story structure. Again, no personal testimonies are possible.
But - what if the dates for Krishna's life are mistaken? What if he lived more recently than in the 4rd millennium BC, and the portion of the Mahabharata which contains his ascension were written closer to the actual event, and the rest of the Mahabharata, including the story-within-story structure, was built up later? Well, that's a lot of "what-if's" - and while that does get the text closer to the event, it's still of no help in solidly placing Krishna in history, or producing personal testimonies from any witnesses to his ascension.
Going to the Srimad Bhagavatam instead of the Mahabharata doesn't help here - for the Srimad Bhagavatam was written even more recently than the Mahabharata. Modern scholarship places its composition as some time between 500 to 1000 AD, and it references parts of the Mahabharata. In fact, its other name - Bhagavata Purana - means "Ancient Tales of Followers of the Lord". The work itself acknowledges that these are "ancient tales", right there in the title. It cannot possibly produce the kind of testimonies we're looking for.
Let's compare all this to the evidence for Jesus's resurrection. Even if we only consider those modern scholars that are skeptical and unbelieving, the New Testament was mostly completed within decades of Christ's death and resurrection. 1 Corinthians, from which we got the summary of the evidence for Christ's resurrection, was written a mere 20 years after the event. The creed within it comes within several years of the event itself. Furthermore, we have numerous records within the New Testament of people claiming to have personally seen the risen Christ. Multiple such claims are in fact made in the first person within the New Testament text itself.
These are stark differences compared to the ascension of Krishna. We have time gaps of years compared to millennia, and personal, firsthand testimonies instead of a story about a story about a story about an ascension. It may be that Krishna was a real person who once lived a remarkable life. It may be that the Kurukshetra War actually took place. But in judging the amount of evidence for Krishna's ascension, there can be no real comparison to the evidence for Christ's resurrection.
But in the end, we still need a numerical value for the level of evidence for Krishna's ascension. Well, we can certainly say that some people say that Krishna "rose from the dead". But we cannot historically locate any group of people who first personally testified to this fact, like we can with the 500 witnesses in 1 Corinthians 15. Nor can we find any group of witnesses corresponding to the apostles, or to the specific named witnesses in 1 Corinthians 15. In the end, we just seem to have the story in the Mahabharata, with the version of the story in Srimad Bhagavatam being a later telling of the same story. Previously, I've assigned such "some people say" stories 1/10th of the level of evidence of the 500 witnesses. But given the sheer size of the works about Krishna, I'll increase this to 1/4th of the level of evidence of the 500 witnesses. That means that the evidence for Krishna's ascension amounts to 1/4 * 1/6 = 1/24th of the evidence for Jesus's resurrection.
Bodhidharma:
Let us now turn to some figures from Buddhism who are said to have appeared after their deaths.
Bodhidharma is the Buddhist monk credited with bringing Chan Buddhism to China, some time around the 5th century AD. Here is Wikipedia's summary of the legend surrounding his death:
Three years after Bodhidharma's death, Ambassador Sòngyún of northern Wei is said to have seen him walking while holding a shoe at the Pamir Heights. Sòngyún asked Bodhidharma where he was going, to which Bodhidharma replied "I am going home". When asked why he was holding his shoe, Bodhidharma answered "You will know when you reach Shaolin monastery. Don't mention that you saw me or you will meet with disaster". After arriving at the palace, Sòngyún told the emperor that he met Bodhidharma on the way. The emperor said Bodhidharma was already dead and buried and had Sòngyún arrested for lying. At Shaolin Monastery, the monks informed them that Bodhidharma was dead and had been buried in a hill behind the temple. The grave was exhumed and was found to contain a single shoe. The monks then said "Master has gone back home" and prostrated three times: "For nine years he had remained and nobody knew him; Carrying a shoe in hand he went home quietly, without ceremony."
So, that's something. We not only have the usual "group of people who believe" that Bodhidharma rose from the dead, but also a named figure, one "Ambassador Sòngyún of northern Wei", who at least sound like a historical person. So, how should we evaluate this story?
As before, we first ask where this story comes from. It turns out that the source for this story is the Anthology of the Patriarchal Hall, which was compiled in 952 - about 400 years after Bodhidharma is supposed to have died. Again, this is far outside a human lifetime, and that makes it impossible to find the kind of personal testimonies of historical individuals that we're looking for.
As for "Ambassador Sòngyún of northern Wei" - well, it turns out that he really is a historical person - a Buddhist monk who was sent into India to acquire some Buddhist texts, some time around 520. But this does not really help the case for Bodhidharma's "resurrection", because none of the texts that mention Song Yun or his journey mentions this "resurrection". The event therefore seems to be a later, legendary addition.
The other sources on Bodhidharma, many of which are earlier than the Anthology of the Patriarchal Hall, also force us to draw the same conclusion. None of them mention this story of Bodhidharma "going home". It is clearly a later, legendary addition, and Wikipedia has no qualms about labeling it as such.
Let's now assign a numerical value to the level of evidence in this story. There's the usual "some people say this happened" dimension to the story, which again gets counted for 1/10th of the 500 witnesses in 1 Corinthians 15 - that is, as 1/10th of 1/6th of the evidence for Jesus's resurrection. As for "Ambassador Sòngyún of northern Wei", having a named, real, historical witness would count as a full 1/6th of the evidence, except that this witness is named 400 years after the fact. This would still count as some non-negligible fraction of that 1/6th, and we'd have to add that in.
But nearly all of this gets wiped out by the strong evidence against the story from the lack of mention in the earlier sources, indicating that this whole story is a later, legendary addition. In the end, the level of evidence for Bodhidharma's "resurrection" can't amount to more than the "some people say" level - the usual 1/60th of the amount of evidence for Christ's resurrection.
Puhua:
Puhua (known as Fuke in Japan) was a Chinese Buddhist monk, who supposedly lived around 800AD. He, too, is said to have not really died. He may or may not have been a real individual. If real, he was a student of Linji (known as Rinzai in Japan), who was another Chinese Buddhist monk, who founded the Linji school of Chan Buddhism.
Here's the story of Puhua/Fuke's death and "resurrection" as told in the Record of Linji, quoted by Wikipedia:
"One day at the street market Fuke was begging all and sundry to give him a robe. Everybody offered him one, but he did not want any of them. The master [Linji] made the superior buy a coffin, and when Fuke returned, said to him: "There, I had this robe made for you." Fuke shouldered the coffin, and went back to the street market, calling loudly: "Rinzai had this robe made for me! I am off to the East Gate to enter transformation" (to die)." The people of the market crowded after him, eager to look. Fuke said: "No, not today. Tomorrow, I shall go to the South Gate to enter transformation." And so for three days. Nobody believed it any longer. On the fourth day, and now without any spectators, Fuke went alone outside the city walls, and laid himself into the coffin. He asked a traveler who chanced by to nail down the lid. The news spread at once, and the people of the market rushed there. On opening the coffin, they found that the body had vanished, but from high up in the sky they heard the ring of his hand bell."
As before, we want to evaluate the evidence for this story, and begin by inquiring about the source of the story.
We've said that this story comes to us through the Record of Linji - a work that was not consolidated until more than 250 years after Linji's death in 866. Puhua, if he was real, died before Linji - as the story itself makes clear. Therefore, this story about Puhua's death and "resurrection" was recorded more than 250 years after the event itself. Again, the large gap, which far exceeds a human lifetime, makes it impossible for us to find anything like the personal testimonies of historical individuals.
More damning still is the other, earlier account of Puhua's death, in the Anthology of the Patriarchal Hall - the same Anthology that recorded Bodhidharma's "resurrection". This text is also known as the Zutang ji, and it contains the first mention of Linji as well as telling the following story of Puhua's death (look on p.312. "ZJ" refers to Zutang ji):
One day Puhua, carrying an armload of coffin-planks, went about town bidding farewell to the townspeople, saying, “I’m leaving this life.” People gathered in crowds and followed him out of the east gate. He then said, “No, not today!” The second day he went to the south gate and the third day to the west gate. By that time fewer people were following him, and not many believed him. On the fourth day he went out of the north gate, but no one followed him. He dug a tunnel, lined it with bricks, and died therein.
This is, of course, essentially the same story as the one found in the Record of Linji - except there is no resurrection. So, Puhua died, supposedly in 840 or 860. We then have the Anthology of the Patriarchal Hall, written in 952, which mentions Puhua's death but says nothing about a vanished body or a resurrection. We then finally come to the Records of Linji, which was consolidated after 1100, where a resurrection shows up attached to the end of the same story as the one in the Anthology of the Patriarchal Hall. We furthermore know that the Anthology of the Patriarchal Hall is not shy about putting in resurrection stories, since it included one for Bodhidharma. So, why does it not include Puhua's resurrection story? Because the story did not exist yet. The obvious conclusion is that Puhua's "resurrection" is a legend developed after 952.
Again, it's difficult to compare something like this to the evidence for Jesus's resurrection in the New Testament. None of the New Testament makes any sense without Jesus having risen from the dead. The whole corpus, from beginning to end, testifies to Christ's resurrection, without ever wavering from that truth. But, we're suppose to assign a comparative numerical value to the level of evidence for Puhua's resurrection - so the only thing we can do is to generously give it the "some people say" value of 1/60th of the evidence for Christ's resurrection.
Our previous calculations are fully validated
So, let us summarized these non-Christian accounts of a resurrection. For each supposedly "resurrected" person, the following table shows the level of evidence associated with their resurrection account, expressed as a fraction of the evidence we have for Christ's resurrection:
| Name of the person | The level of evidence |
| Apollonius of Tyana | 1/30th |
| Zalmoxis | 1/60th |
| Aristeas | 1/24th |
| Mithra | 0 |
| Osiris | 1/60th |
| Dionysus | 1/60th |
| Krishna | 1/24th |
| Bodhidharma | 1/60th |
| Puhua | 1/60th |
Here's how this looks like in a histogram:
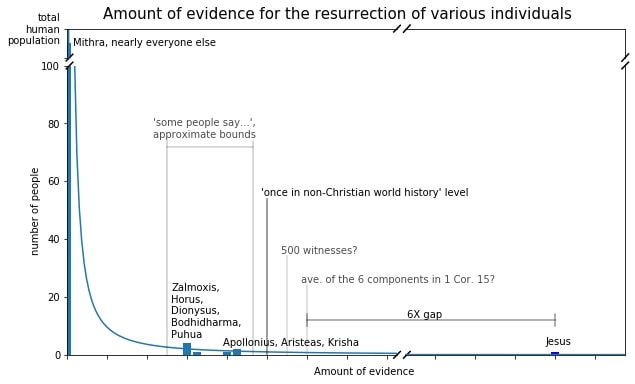
What does all this tell us? Quite a bit.
Let us recall our purpose in collecting these non-Christian stories about a "resurrection": we wanted to verify our Bayes factors for the evidence involved in Christ's resurrection.
The first part of our plan was to find other non-Christian resurrection testimonies matching any one of Peter, James, or Paul's testimonies, to validate our estimate of 1e8 as the Bayes factor of a single human testimony. As we saw, there was not a single instance of such testimony in any non-Christian resurrection stories among the ones we investigated. There may perhaps be one such testimony if we exhaustively investigate the entire historical record, but even that's doubtful, as we've already looked at the likeliest candidates, and we're already very much scraping the bottom of the barrel.
So, it turns out that if anything, 1e8 is an underestimate for Peter, Paul, and James's testimonies. The full-blown set of conditions associations with their testimony strengthens them significantly beyond just a sincere human testimony. Our best guess is that there may be a single non-Christian testimony that matches one of theirs, but it's unlikely. Given our earlier estimate of 1e9 reportable deaths, that puts Peter, Paul, and James's testimonies, individually, at a Bayes factor of greater than 1e9.
This also validates our estimate that Peter and Pauls testimonies, taken together with their anti-dependence considerations, have a Bayes factor safely above 1e11. We could not find even a single testimony matching either Peter or Paul's testimony individually, let alone two such testimonies, or two such testimonies where the individuals started out as enemies. As if that wasn't enough, we could not find even a single case where an initial skeptic of a resurrection changed his mind, even in a mythical story. No matter how one slices it, Peter and Paul's testimonies together are far above what might have appeared naturalistically in world history. There is nothing that matches them and nothing that can even come close to them. This validates my earlier claim that just these two testimonies together are enough to make Jesus's resurrection quite probable.
Lastly, we wanted to compare the total evidence summarized in 1 Corinthians 15 with the "nearest approach" by a non-Christian resurrection story in history. As it turned out, the "resurrection"s of Krishna and Aristeas had the most evidence behind them, amounting to roughly 1/24th of the evidence for Christ's resurrection. According to our program, this must be assigned a Bayes factor of roughly 1e9. Then, if we assumed independence, 24 times that amount of evidence would correspond to a Bayes factor of 1e216. This is such an absurdly large number that it's essentially impossible for it to be reduced below 1e11, even if you factor in dependence, even when crackpot theories are in play. We will later demonstrate this with a full calculation. But for now, we can qualitatively say that our earlier claim is validated: the total amount of evidence for Jesus's resurrection is not just greater than anything that was naturalistically generated in history. It is not even just distinctly greater than that by a significant margin. No, it is greater by a large multiplicative factor, to the point where the two are hardly comparable. Beyond any reasonable doubt, Jesus Christ rose from the dead.
PART III:
Answering simplistic objections
Chapter 7:
The usual barrage of objections
What, if anything, is wrong with the previous argument?
It is natural and proper to critique an argument. Some critiques are weak, brought on by those who are looking to provide the thinnest veneer of intellectual justification for their unbelief. Others may actually succeed in countering the argument. Therefore, critiques must be critiqued in turn, and the original argument's mettle can be thereby ascertained.
Is the prior too large, especially for a supernatural event?
One possible class of objections would try to argue that the prior probability for the resurrection wasn't small enough. One may say:
"It's not just that people don't rise from the dead. NO supernatural claim of ANY KIND has EVER been validated in a controlled setting. Therefore the prior probability for the resurrection must be smaller than the value used in the calculation."
Well, let's just give away everything this objection asks for. So, take every human to have ever existed (1e11), and say that every single person has made 100 supernatural claims, all of which we've tested in a "controlled setting" and have proven false. We will just ignore the fact that this level of testing simply hasn't been actually done. If we were to grant all that, the upper bound on the prior probability of the resurrection would drop from 1e-11 to 1e-13 - woefully inadequate for materially changing any of our previous conclusions.
Recall that the argument for the resurrection currently has overwhelming evidence for it. Just 1 Corinthians 15 has multiple times the evidence of just Peter and Paul, whose testimonies were already enough to safely and significantly overcome the prior of 1e-11. On the other hand, a change in the prior from 1e-11 to 1e-13 only requires 2/11, or 18% more independent evidence.
Furthermore, the argument for the resurrection is backed up by the historical record: There is no non-Christian resurrection report which approaches anything like even a single one of the many pieces of evidence in 1 Corinthians 15. This suggests a new way to evaluate this objection: does the skeptic fare any better if we expand our examination of the historical record from "non-Christian resurrection reports" to "non-Christian miracle reports"?
As we will later see, they do not. Even with this expansion to any kind of miraculous reports, there are hardly any that come close to matching, say, Peter's testimony by itself. This means that the rest of the argument follows through unchanged: Peter's testimony by itself is enough to negate nearly all of the prior, Peter and Paul's together is enough to safely and significantly overcome it, and all of 1 Corinthians 15 completely overwhelms it.
"But Science!"
Here is another objection along the "prior is too big" line.
"But science says that miracles can't happen; so whatever prior probability value you've set for the resurrection must have been too big to start with. If the conclusion to the calculation is that the resurrection actually happened, we must reduce the prior probability, so that we can arrive at a rational, scientific answer."
One wonders at how anyone can invoke "science" after abandoning empiricism and ignoring mathematical reasoning. This kind of statement betrays a willingness to pay lip service to math, reasoning, and science, while ignoring the conclusions that these fields actually lead to - all for the purpose of clinging to a bankrupt preconceived notion.
For instance, I have seen numerous skeptical arguments about miracles that mention Bayes' theorem and their prior probabilities. I have not seen a single one of these put an actual, numerical value to this prior probability. Among all the ones that I've seen, the argument has ALWAYS been "and since this number is going to be so small, it might as well be zero, although the value isn't actually, absolutely zero". So they claim to acknowledge that the prior probability can't be zero, while the argument functions as if it were zero in all circumstances. Thus they pay lip service to probability theory, while ignoring it in practice, to reach their preconceived conclusions.
You must actually do the math. Use Bayes' rule. At the very least, don't just bring it up only to have your biases negate the whole point of using Bayesian reasoning. Try to assign actual values to the various probabilities and likelihoods, even if they're just order of magnitude estimates. Base these values on some kind of empirical data. And most importantly, don't just reject the conclusion because it didn't agree with your preconceived notions, or fiddle with the numbers to arrive at the conclusion you were looking for.
Can human testimonies be trusted?
"The Bible is something that some people just wrote down, right? In general, you can't trust people. Human testimonies are worthless", says the naive skeptic. Now, I should be charitable, and assume that such a person is consistent in their view. Then they ought to distrust every expression of human thought they have ever read or heard in any medium. Their entire knowledge base ought to have been established via personally conducted direct scientific experimentation. One wonders how their visits to the doctors go, or what it was like for them to learn to dress themselves.
Perhaps this objection could be tempered by saying that human testimonies aren't entirely worthless, or that testimonies of certain types can sometimes be trusted. If you're in this camp, I certainly hope that you keep your mind open when someone comes to you with ample, empirical evidence from multiple sources using multiple, independent lines of thought about exactly how to quantify the strength of a human testimony.
So, human testimonies cannot be dismissed out of hand simply because they're human testimonies. But this detour does raise the issue where testimonies of a certain type, made under certain conditions, may be less reliable. Let's see if there's any tenable objections along this line of thought.
Could the disciples have been genuinely mistaken?
One such objection might go like this:
"1e8 is a ridiculously large Bayes factor for people's testimonies. People make mistakes all the time. Do you not know, for instance, how inaccurate eyewitness testimonies are? It is far more likely that the reports of Jesus's resurrections are mistakes of this type, rather than an accurate depiction of the events."
First, let's go over a few things before we tackle the specific issue on the reliability of eyewitnesses. The value for Bayes factor that I used - 1e8 - is derived from the strength of a human testimony in general, with relatively few conditions attached to it. It is the typical value to be assigned for someone saying "yes, this really happened", in circumstances similar to the disciples after the resurrection. Of course, if you start adding conditions to it, these will change the value of the Bayes factor, as we have already seen. So I have no problem acknowledging that eyewitness testimonies can often be mistaken, and that it's in human nature to give flawed testimonies under certain conditions. In such conditions the Bayes factor for a testimony must rightfully be severely discounted. However, one must also acknowledge that there are also conditions that dramatically enhance the value of human testimony - note the previous example of a chess game record, with a Bayes factor exceeding 1e120.
There is therefore bound to be a number of objections which effectively say "see how unreliable humans are (in these specific circumstances)!" What we must do, then, is to compare the circumstances in these objections to the actual circumstances surrounding the testimonies about the resurrection. We will see that, upon actually making this comparison, the testimonies for the resurrection are actually strengthened, rather than weakened, at nearly every turn by the specific circumstances surrounding them.
So, let's tackle the issue of eyewitness testimonies. The question of unreliable eyewitness testimonies typically come up in a courtroom setting, where a bystander is identifying someone they saw during an incident under investigation. A common example may have a policeman asking a witness, "now ma'am, can you point out which one of the fellows in that lineup was the one that pointed the gun at the cashier?"
Now, let's identify some of the common circumstances surrounding these events, about which such testimonies are made:
The witness is nearly always a bystander - a stranger who was previously not familiar with any of the actors in the crime.
The event in question often takes place in a matter of minutes, if not seconds. Witnesses are often caught by surprise - the crime takes place at its own pace, with no regard for making things easy for the witnesses. Indeed criminals often rely on the shock and the quick pace of the events to hinder possible identification and later prosecution.
There is often extreme stress placed upon the witnesses, who are fearing for their immediate personal safety. This may especially be the case if a weapon is present, which draws the focus of the victims or witnesses to it, and away from the proper identification of the perpetrator.
Related to the above, witnesses in such testimonies are often not primarily concerned with the identity of the perpetrator. In the moment, they are often simply shocked by the event, or mainly concerned about their bodily safety.
Now compare these to the testimonies about Jesus's resurrection:
Jesus was the most important person in the disciples' lives. He was explicitly more important to them than their family members or hometown friends. They had been around each other constantly for the last several years, and were familiar with one another as much as anyone can be.
Jesus's post-resurrection appearances occur multiple times, often in extended scenes where he converses with the disciples at length about what this all means. He eats with them, talks with them, and teaches them. Jesus furthermore specifically has these discussions for the benefit of the disciples, so that they can better understand his resurrection.
The pervasive mood during these post-resurrection appearances must have been awe and excitement. There is an optimal amount of stress for peak human performance, at a level which is neither too little (with accompanying boredom and lethargy) nor too much (with accompanying nervousness and panic). Speaking with the risen Christ must have put the disciples near this optimum peak, with an exhilarating atmosphere pervading every moment of their discussion.
The chief thought in the disciple's mind in each of these meetings must have been primarily about Jesus. 'Wow, it really is the Lord! He is risen from the dead! What could this all mean?' He commanded their wholehearted attention at each of these post-resurrection meetings.
So upon making this comparison, the result is clear. For each of the factors which causes courtroom eyewitness testimonies to be unreliable, the disciples' testimonies about Jesus are found to have the exact opposite property: they're testifying about someone they know very well (instead of a stranger), about events which happened repeatedly over an extended period of time (instead of being over in a flash), under the optimal amount of stimulation (rather than under crippling fear), with the person of Jesus as the chief object of their focus (rather than being shocked or focused on their immediate bodily safety). Insofar as the circumstances surrounding a typical courtroom eyewitness testimony cause them to unreliable, the same reasoning requires that the disciples' testimonies would then be especially reliable.
To put it simply, the example of unreliable courtroom witnesses only demonstrate how different the disciples' testimonies about the resurrection are. The disciples were not doing anything like saying "yes, that man with the red hair there is the man who pointed the gun at the cashier", with its accompanying uncertainty. No, their statement is rather more like a woman saying "yes, my husband really is the man I married at my wedding". Good luck finding many women who are mistaken about that.
Therefore, the Bayes factor associated with the resurrection testimonies must be greater than they were in the unconditioned case. 1e8 may have seemed like an overestimate upon a superficial comparison, but a more careful consideration reveals that it is actually an underestimate: none of the factors that weaken a courtroom testimony are present, while all of their opposite qualities infuse the disciples' testimonies and correspondingly strengthen them.
Or actively deceptive?
Yet another class of objections may argue for 1e8 being too large, on the basis of people being intentionally deceptive rather than being mistaken. It may go like this:
"1e8 is a ridiculously large Bayes factor for people's testimonies. People lie all the time. Do you really think that only 1 out of 1e8 things that people say are lies? There are conspiracies, con artists, and fame seekers everywhere, at all times. What makes you think that the disciples reporting on the resurrection were not just one of these people?"
The objection here, and its answer, is much the same as before. Yes, people lie, or are otherwise unreliable, in some circumstances. These circumstances rightly require us to adjust the Bayes factor downwards. But the comparison of such circumstances with with what the disciples actually faced will only reveal their vast differences. If you think that people are likely to lie under certain circumstances, you must then therefore think that the disciples were highly likely to be truthful about the resurrection, due to the absence of these circumstances.
So, taking lottery winners again as an example: if someone claims to have won the lottery, their claim should be given about a 1e8 Bayes factor. But what if they then go on to say that they've left their winning ticket with a Nigerian prince, and that they would share their winnings with you if you would only give them $5,000 to cover their travel expenses to retrieve the ticket? Well, now the Bayes factor drops precipitously, down towards zero.
However, what if the supposed lottery winner instead gives lavish gifts to their friends and family, buys a new house, then hires a financial adviser to discuss the tax implications of their sudden windfall? Then the Bayes factor would dramatically increase, towards values like 1e120.
So then, what are the circumstances under which people are likely to lie? And in contrast, what are the circumstances that the disciples faced?
Well, people often lie for material gain, as in the above example of a con artist. The disciples, however, did not accrue wealth by claiming that Jesus had risen from the dead; in fact the very nature of their claim made this outcome highly unlikely, with the emphasis on serving the poor and a general disdain for worldly gain. If money was their goal, this was certainly the wrong way to go about it.
People may also lie under social, psychological, or physical pressure, as in the cases of false confessions obtained under harsh interrogation or torture. The disciples, however, resisted such pressure, and held on to their testimony under immense opposition of all kinds. The imminent possibility of persecution is a constant theme throughout the entire New Testament. In fact, many of the early Christian leaders underwent torture and martyrdom, including all three of the named witnesses I used in my calculation (James, Peter, Paul). We know how effective such treatment can be in eliciting false confessions even from their modern victims. We must therefore consider anyone who resisted the far harsher ancient versions of these treatments to be exceptionally trustworthy.
One may argue that at least the negative social pressure from society at large may be made up for by the approval from the close-knit Christian community. But this simply does not apply. Again, among the three named witnesses I used in my calculation, only one (Peter) was originally one of Jesus's disciples. James and the rest of Jesus's family are considered to have been in a somewhat disharmonious relationship with Jesus before the resurrection, and Paul was a complete outsider - an early persecutor of the church, whose personal and social identity was very much set in opposition to Christianity. So in a majority of these cases, the close-knit approval would have gone the other way: they would have ample reasons to reject the resurrection. Their testimonies in spite of this, therefore, must be counted as being much more reliable than the average.
People may also lie for fame - they claim to have achieved something remarkable or to be someone special. But as we have just seen, the fame that came with proclaiming the resurrection would have been exactly the wrong kind of fame; the witnesses would have been shunned both by the Roman and Jewish society at large, and in many cases by their immediate social circle. Furthermore, it is the nature of fame to be fleeting; few would continue to lie for fame, in the face of intense opposition, for decades at a time, long after the shock of the initial claim wore off, to the point of death. Indeed, if the witnesses were fame-seekers of this type they would have done quite well by recanting the resurrection at the last minute and becoming a kind of whistle-blower for this deception that Christians pulled over the world. And yet, the witnesses did no such thing; they all died as martyrs.
People also sometimes lie for a cause. If they believe that some agenda is good and important, that may cause them to be deceptive "for the greater good", to advance that agenda. But this is impossible given the theology of the early church. Jesus was the greatest good; his resurrection was the most important event in the whole world. There was nothing greater which would be worth lying about the resurrection.
In all this, the actions of the witnesses were in perfect accord with their genuine belief in the resurrection. They had no reason to lie and every reason to tell the truth. We, also, have no reason to believe they were liars and every reason to believe that they were truthful.
So, it is true that men often lie. But this is a shallow observation. Upon considering the actual, specific circumstances surrounding the resurrection testimonies, we find that they are diametrically opposed to the circumstances conducive to lying. Therefore, the observation that "men often lie" only serves to enhance the trustworthiness of the witnesses to the resurrection, by pointing out how different these witnesses are from typical liars.
We ought to have reduced the Bayes factor for the resurrection testimonies down from 1e8 had we found the surrounding circumstances conducive to lying. But since the opposite has happened, we must therefore increase the Bayes factor. 1e8 is a distinct underestimate of its true value.
Or actually crazy?
Another class of objections would just argue that the witnesses to the resurrection were crazy:
"Obviously anyone who claims that they saw someone coming back from the dead is crazy. How can we take their stories about these outlandish miracles seriously? Clearly there was something mentally wrong with these people, and we ought to dismiss their 'testimonies' as the ramblings of the insane or the schizophrenic."
By now, it ought to be obvious that I'm going to handle this objection like all the others. Did the witnesses to the resurrection act like they were crazy? Did they exhibit the typical behaviors of the insane or the schizophrenic? If they did, we should rightly lower the Bayes factor for their testimonies from the relatively unconditioned value of 1e8. But if they did not, then by the same logic we must increase the Bayes factor.
This investigation is straightforward enough: read the New Testament, and look for symptoms of mental illness in areas that are not directly related to supernatural claims (one must be careful about circular reasoning). So, does the New Testament read like the work of a schizophrenic? Does it seem to describe people who were afflicted by mental illness? Would you say, for instance, that Peter's sermon at Pentecost exhibits problems with attention or memory, or that Paul's letter to the Romans demonstrate disorganized thinking?
In fact, apart from the supernatural components, I have not heard of anyone citing any part of the disciple's work in the New Testament as being characteristic of mental illness. If there is such a passage, I'd love to know about it. Can anyone point to a verse and say, "here is where Paul shows clear signs of psychosis", or "this is where Peter displays the classic symptoms of schizophrenia"? It says a great deal about the "insanity" accusation that the only evidence they can find for it are the very parts that make up the question at hand, the very parts they object to. In short, the objection effectively only amounts to saying "I disagree with these people on these points, so they must be crazy!"
On the other hand, there are plenty of reasons to think that the witnesses to the resurrection were of sound mind. Remember, they were the organizers and leaders in the early Christian church - a movement that spanned their known world. Furthermore, recall that they were successful beyond any naturally possible expectations: Christianity has lasted thousands of years until the present day, multiplied wildly, and now spans the whole globe. Can anyone give any example of an organization run by insane people that was even a millionth as successful?
In particular, the ideas behind this organization - that is, the theology of the early Church - are readily available to us as the text of the New Testament. They are the most read, discussed, studied, and applied texts to have ever been written. If you're reading this you're also free to go and read the New Testament. Does it seem like the work of the insane? What work by any mentally ill persons has ever reached a fraction of its stature?
So the conclusion is clear enough. Once again, upon actually considering the facts surrounding the resurrection witnesses, we find that they do not correspond at all to the scenario in the objection. The disciples display no sign of insanity, instead demonstrating many characteristics of sound and acute minds. So, according to the very logic embedded in the objection itself, this must again increase the Bayes factor of their testimonies. As we have repeatedly said, 1e8 is an underestimate. The true value must be higher - likely in excess of 1e9 - and this is also borne out through the empirical, historical record.
Or some combination of the above, or something else entirely?
Here is another typical attempt to deny Christ's resurrection:
"It may be that some of the disciples were crazy or especially grief-stricken after Jesus's crucifixion. This lead them to see some vivid visions of Jesus, which they related to the other disciples. Some of these other disciples, who had not seen the visions themselves, then spread the story about the 'resurrection' based on the vision of these few crazy people. Then, a few other disciples, who were dissatisfied with Judaism, formed an opportunistic conspiracy to start a new religion based on these budding stories about this 'resurrection', and that's how Christianity started.
Or, it could have gone another way. A few disciples wanted to start a new religion and formed a conspiracy. After Jesus's death, they suggested to some of the other, more gullible and mentally unstable people that Jesus rose from the dead. With a little faking of evidence, social pressure, and the power of suggestion, they eventually got enough of the other disciples to say that they saw the resurrected Jesus themselves. From there, the resurrection became part of their faith narrative, and that's how Christianity might have started.
There are dozens of other possibilities like these - it doesn't have to be that everyone was lying or crazy. We just need the right combination of lies, mistakes, and insanity at the right times and situations for Christianity to start. Surely, it is more likely that one of the many possibilities represented here lead to the belief in the resurrection rather than for Jesus to have really come back from the dead."
We can, of course, answer this objection like we answered the previous ones: there is less than no reason to give credence to any of these scenarios, so the Bayes factor for the resurrection testimonies must therefore correspondingly increase. As the current objection is merely combinations of already discredited previous objections, this is quite adequate.
But there is a more pressing concern: anyone willing to entertain objections of this type has a fundamental misunderstanding of how Bayesian reasoning works. This has likely led them to vastly underestimate the strength, resilience, and robustness of the argument for the resurrection. Much of the above sections, about how a failed objection actually strengthens the case for the resurrection, was probably lost on them.
For these reasons, we now need to go over the nature and strengths of a Bayesian argument, and how it handles all such simplistic objections.
Chapter 8:
The strength of a Bayesian argument: why none of these objections work
The nature of Bayesian arguments.
After hearing many objections in succession as we just have, it's easy to lose sight of the big picture. For instance, one may fall into the trap of thinking that if even one of these objections has even the slightest chance of being true, the argument would fall apart. But is that really the case? If the disciples had even the slightest chance of being crazy or mistaken or deceptive about the resurrection, would that cause the whole chain of reasoning to break and the case for the resurrection to collapse?
Bayesian arguments are not deductive arguments.
This is where it's useful to remember the big picture. You see, a standard deductive argument does work like that - A and B together lead to C, which lead to D, which then leads to the conclusion. For such an argument, all of its premises must be entirely true and each step of its reasoning must be completely correct. Anything else invalidates the whole argument. That is why a barrage of objections can sometimes succeed against such an argument, or at least cast doubt on its soundness.
But my argument for the resurrection is not a deductive argument. It is an order-of-magnitude probability estimation argument using Bayesian reasoning. The objections against it can only take two forms: you must either claim that I'm misusing the mathematical apparatus (that is, Bayes' rule), or disagree with my estimated probability values.
If you think that I've made a mistake in applying Bayes' rule, then by all means point it out. Otherwise, the objections against it come down to wrangling over the probability values, which were empirically derived and double-checked using multiple, disparate lines of evidence. Those two things - mathematical laws, and empirically derived and double checked probability values - are the foundations of the argument. They are what an objection must fight against. They are what needs to be taken down in order to tear down the argument.
It is easy to fall back to thinking in terms of a deductive argument when you hear many frivolous objections in succession - that one of them must eventually get through a chink in the armor and deliver the fatal blow. If only someone could just be clever enough to think of that one silver bullet! But it simply does not apply to our case. Bayesian arguments are naturally and fundamentally immune to this type of attack.
Bayes factors do not require certainty.
The point here is that in the wrangling over probabilities need not produce absolute certainty. The argument does not depend on it. I do not need to claim, for example, that there is absolutely no chance that the disciples were lying. Having demonstrated that the Bayes factor for a typical, relatively unconditioned human testimony is around 1e8, I only need to demonstrate that the disciples are not more likely to be liars than such a "typical" person. In fact, anything which suggests that the disciples' honesty exceeded that of the "typical" person actually strengthens the argument beyond its original form, by increasing the Bayes factor. This is what has actually happened upon the examination of every objection thus far.
Bayesian arguments compel belief.
(new material) You do not get to choose to be convinced. (add?)
Bayesian arguments are robust.
But let us imagine that one of these objections somehow actually succeeded. Say, for instance, we found actual evidence where the disciples were offered a monetary compensation from an external source for spreading stories about a resurrection. What would it do to the resurrection argument?
This would still not be a fatal blow. A single objection - even a completely real, legitimate, successful one - doesn't simply invalidate a Bayesian argument. We would merely have to re-calculate the final odds. In the above example, the scenario would correspond closely to the 9/11 fraudsters lying about their victimization, so the Bayes factor of the disciple's testimony would likely drop from about 1e8 to 1e6, according to our previous calculations.
But even with this theoretical coup for the skeptics, this would not materially change the outcome. As we have previously calculated, a Bayes factor of 1e6 still gives Peter and Paul's largely independent testimonies enough strength in combination to overcome the prior of 1e-11, and the remaining testimonies in 1 Corinthians still provide overwhelming evidence for the resurrection.
That is the robustness of a Bayesian argument, and the argument for the resurrection in particular. A few objections - even completely real, legitimate, and successful ones - are unlikely to do more than put small dents in it, while unsuccessful objections actually strengthen it.
Of course, this assumes that the objections are successful in the first place. And here, we get to the main problem with these previous simplistic objections, and the reason why all of them can be simply dismissed out of hand.
Only evidence moves the odds. Speculations do nothing.
Here is the simple, fundamental fact that all of these objections are ignoring: once you have a Bayes factor calculated from empirical evidence, the only thing which can change or correct it is more evidence. Mere speculation does nothing. All possible speculations - 'they were crazy', 'they were lying', 'they were mistaken', etc. - are already taken into account in the calculated Bayes factor of 1e8. Merely enumerating some of the ways the speculation could have played out does not change anything.
Imagine, for instance, that your friend claims to have been struck by lightning. You've taken stock of this claim, and based on the empirical evidence of other similar claims, decided to assign it a Bayes factor of 1e8. But then someone says, "well, your friend may be just a little crazy. And he might have had a nightmare about a thunderstorm last night. Then he might have gone to a hypnotist who had him recall that nightmare, which he's now confusing with reality. Or maybe it was the hypnotist who planted the suggestion in his mind first and that caused his nightmare. Really, it might have been any of these things - and isn't it more likely that at least one of these possibilities is true, rather than for him to have been actually struck by lightning?"
Should you or your friend then discount the previously assigned Bayes factor in light of these new possibilities? Absolutely not. Again, the Bayes factor ALREADY includes all of the ways that this claim may be wrong. It is the numerical estimation of the weight of evidence for a human testimony, and as such already inherently includes the possibility that the evidence may be misleading.
Having established its value, it is simply incorrect to further modify it with no evidence, based on enumerating possibilities that were already included in its evaluation. Your friend's proper reply to this wild speculation would be to say, "what makes you think that I had visited a hypnotist or had a nightmare? Of course, anyone might be wrong about anything in any number of ways - but my friends already know how much trust to put in my testimony. How does a list of ways that I might be wrong, with no evidence behind any of it, make them trust me less?"
Now, is there any evidence that your friend did really visit a hypnotist recently? Then it is proper to include that evidence to re-calculate the probability of the lightning strike. Similarly, is there any evidence that a crazy group of Jesus's disciples reported on the resurrection, which then got hijacked by a conspiratorial group of disciples? Then it would be proper to include that evidence to re-calculate the probability of Christ's resurrection. However, in the absence of such evidence, the mere existence of that possibility cannot change our calculations. Such possibilities are already included in the initial calculation.
Let me give an even simpler example. Suppose you flip a coin, then cover it up so that you don't know the outcome. Not having investigated the coin all that carefully, you assume that the probability of it turning up 'heads' is 0.5, based on the empirical evidence of coin flips in general. Now, someone comes up to you and says, "but consider all the ways that it may turn out to be tails. It might have hit the tabletop, flipped three times after the bounce, landed on its edge, then fallen over to show tails. Or it may have flipped fifteen times before the first bounce then landed flat with the tails side up. In fact, if the coin's leading edge strikes the table at 15 degrees with an angular velocity of 12 rev/s and a downward linear velocity of 2 m/s, it's guaranteed to end up tails. And this is only a small sample of the innumerable ways for you to get tails. Given all these different ways, shouldn't you decrease your 'heads' probability?"
Of course not. In modifying your empirically calculated probability, you must only consider the evidence that you actually have. You must disregard any evidence that you could have had, or wish you had. So, in the absence of any evidence, the probability for 'heads' is still 0.5, and the innumerable ways that the coin might turn out to be 'tails' does nothing to change it. Now, it may be that you recorded the first part of the coin flip in slow motion, and it turns out that the coin did indeed strike the table at an angle of 15 degrees for its leading edge, with an angular velocity of 12 rev/s and a downward linear velocity of 2 m/s. That would be evidence. That would cause the probabilities to change. But the mere possibility of this happening, in the absence of the actual evidence, does not change the probability.
Here is the evidence that we actually have: numerous witnesses gave their earnest, personal testimonies, saying that they personally saw the risen Christ. We know how to numerically evaluate such evidence. We have already numerically taken into account the many ways that they may have been wrong, whether through honest mistakes, deception, or insanity. All such possibilities are already included in our Bayes factor of 1e8. We have less than no evidence that anything like the speculative scenarios in the objections have taken place, and the mere possibilities for these speculations cannot change the empirically derived probabilities. Therefore the odds for the resurrection remains undiminished by the objections: Jesus almost certainly rose from the dead.
The lack of evidence against the resurrection
In answering these objections, we've touched on the lack of evidence for giving them any consideration. A skeptical reader may wonder whether I've ignored any evidence against the resurrection, or how I would answer this or that argument by this or that person. A large part of my reply would be that there is no significant evidence against the resurrection.
Let me reiterate and clarify that, because it's important. There is an utter lack of evidence for disbelieving the resurrection: literally every single document we have by the people who were actually connected to the event to any reasonable degree ALL portray the resurrection as something that actually happened.
If you believe in the resurrection, you have the unanimous support of every author who were actually close to the event and would know for certain. If you disbelieve the resurrection, literally all such evidence - every single testimony of every single individual who ever wrote personally about the actual event - is against you.
So, I'm not being selective about the evidence. There is nothing to be selective about, because there is essentially no evidence for the opposing argument. This is why I'm fundamentally unconcerned about any arguments against the resurrection: because they have no evidence. The only thing I've done in choosing my evidence was to handicap my own argument, by only using a small fraction of the total evidence available.
If there were any evidence against the resurrection, I'd be glad to incorporate it into the calculation. I've already said elsewhere that a sufficiently strong evidence against the resurrection can falsify the whole hypothesis for me - if, you know, such things actually existed.
So, does anyone know of a cave in Israel that houses Jesus's mummified corpse? By all means, tell me about it. Is there an ancient manuscript that exposes the disciples' conspiracy to fake the resurrection? Let me know. Is there a record of a Roman interrogation where an apostle confesses to having made up the whole resurrection thing? Is there an epistle where a disgruntled disciple warns the others about staking the faith on a schizophrenic woman and her crazy resurrection story? Is there any record of a psychoactive plant in first century Jerusalem that causes vivid mass hallucinations about the recently deceased? Is there a complaint from Jesus's family about how his message has been hijacked by a bunch of lunatics and their crazy resurrection story?
You see, nothing remotely like any of the above actually exists. There is zero evidence for disbelieving the resurrection.
This is why every single skeptical attempt at explaining the resurrection relies entirely on ignoring the existing evidence, and making stuff up instead. They have no other options, because they have no evidence on their side. That's why the only thing they can do is to ignore the existing evidence, and make stuff up.
So, when they say that Jesus's resurrection was a myth that grew over time to be accepted as fact, they're ignoring the existing evidence that says that the resurrection was at the very core of Christianity from its inception, and making stuff up instead about how a myth might have eventually gained enough traction to be accepted as dogma.
When they say that Paul might have converted because he already had second thoughts about Judaism before encountering Jesus on the road to Damascus, they are ignoring the existing evidence in Paul's own testimony, and making stuff up instead about what they think went on inside Paul's head.
When they say that the early Christians didn't believe in a real, physical resurrection, they are ignoring the existing evidence that unanimously say that Jesus's body was missing from the tomb, and are instead making stuff up about what they think the early Christians really thought.
When they say that Jesus might not have really died, but only swooned, they're ignoring the existing evidence that clearly presents Jesus's death, and making stuff up instead about the combination of circumstances that might have allowed Jesus to survive a crucifixion.
When they say that the post-resurrection appearances were only visions or hallucinations, they're ignoring the existing evidence that unequivocally states the physical nature of Jesus's new body, and making stuff up instead about the disciples' mental conditions.
When they say that the gospel writers were only interested in the theological and literary dimensions of their story, and showed no concern for the truth, they're ignoring the existing evidence from these writers themselves that directly contradicts them, and making stuff up about the writer's "true" motivations instead.
So, let's not be distracted by such made-up speculations. Remember the outline of the argument at hand. We are using Bayesian reasoning. We start with a prior probability for the resurrection, and modify it according to the Bayes factor of the evidence. Only evidence moves the probabilities; speculations do nothing. So we only consider the the evidence that we actually have, and disregard any speculative "evidence" which we might have had or wish we had. The numbers in this calculation already account for any point the skeptic may want to make: the inherently unlikely nature of the resurrection is already included in the prior, and the possibilities for the disciples being wrong are included in the Bayes factors. Upon carrying out this calculation, using very conservative values, we find that the evidence completely overwhelms the prior. Jesus almost certainly rose from the dead.
PART IV:
Addressing all possible alternatives
Chapter 9:
Time to address the crackpot theories
The next steps
We've just discussed how there is no evidence against the resurrection: literally every single written record we have by the people who were actually connected to the event to any reasonable degree all portray the resurrection as something that actually happened.
However, it's worth asking - what's the closest thing to an 'evidence against the resurrection'? Are there any historical record from around the time of the event which suggests that the resurrection didn't happen?
Ironically, the closest thing I know is actually in the Gospel of Matthew, where the author mentions the guards to Jesus's tomb being paid off to spread a rumor about the disciples stealing the body. So, I suppose at least some people at the time were saying that Jesus didn't really rise from the dead.
Now, this hardly count for anything in terms an opposing evidence - we don't have an actual personal testimony, our source Matthew clearly presents it as a lie,and if anything it confirms that the tomb was guarded and yet turned out empty.
However, it is instructive to see the nature of this lie. Why did they choose this lie? What do people do when they're faced against a mountain of evidence against their position, yet must find ways to ignore that evidence? What do they do when they know they're wrong? They turn to a crackpot theories, of course. They do things like make up a conspiracy theory about the disciples stealing the body.
You see, we can now be very confident that Jesus rose from the dead. The calculation which first gave us this confidence has now been verified in multiple ways, using completely different methodologies, with everything solidly grounded in empirical, historical data. Everything checks out, and all the numbers are in harmony.
But all this has been computed under the assumption that there isn't any extreme dependence in the disciple's testimonies. We have not yet accounted for the possibility that the entire set of testimony about Jesus's resurrection might have been been engineered to be in agreement by some unknown force. That is to say, we've been discounting crackpot theories - like a conspiracy by the disciples to steal Jesus's body, or an alien mind-controlling all the witnesses to the resurrection.
Note that this is beyond any kind of "normal" dependence, like ordinary social pressure or group conformity. The case for the resurrection is so strong that even when such mechanisms are included the conclusion is beyond doubt: recall that even just Peter and Paul's testimonies, with full dependence factors, was enough to safely conclude that the resurrection happened. No, the kind of crackpot theory we're talking about would involve, at a minimum, a conspiracy involving the disciples and their enemy Paul, and a plan to persecute their fellow Christians, offend their fellow Jews, and invite the ire of Rome, all for no discernible gain.
Ignoring such theories is fine and good, as long as both sides of the debate are agreed in dismissing them. Most doubters of the resurrection do not subscribe to these extreme theories, so carrying out our calculations in this way up to this point was still productive. However, they're now facing an overwhelming amount of evidence. Even a small fraction of it possesses a Bayes factor well in excess of 1e11. The total Bayes factor, without crackpot theories, turned out to be something like 1e48, even with some 'ordinary' dependence factors included. The final odds turned out to be something like 1e37. All the key steps in the argument have been double-checked and re-verified in multiple ways. The posterior probability against the resurrection has now become so tiny, that the small prior assigned to crackpot theories now seem much larger in comparison. Someone set on disbelief can no longer ignore these theories. Indeed they have no other choice: they must fully embrace these crackpot theories.
Examining crackpot theories, in general
Let us examine this general class of theories, that postulate a near-total interdependence in the evidence against them. What kind of theories are they? What are their properties? Is it fair to characterize them as "crackpot" theories?
Now, note that such theories generally require a conspiracy of some kind, almost by definition. Near-total interdependence means that what appeared to be many pieces of evidence was really just controlled by a singular false entity, which manufactured all the other pieces of evidence. Whether this source was a group of disciples or an elite Roman secret society or some space aliens or whatnot doesn't particularly matter - All such theories share the following traits.
The first thing to note about such theories is that they have very low priors probabilities to begin with. Indeed, among those skeptical of Christ's resurrection, a theory of this type is almost never their first choice. Few people want to be labeled a conspiracy theorist, after all. The skeptics want the resurrection testimonies to have been produced "naturally". They'll invoke known social phenomena such as myth generation over a long time, or religious fervor or delusion. They want such ordinary explanations to be a plausible way to generate the resurrection testimonies. Of course, what we've demonstrated thus far is that such explanations are in fact not plausible.
Maybe some people will say that they'd rather be a conspiracy theorist than believe in the resurrection. But even so, such people only say this as a backup, while still trying to argue for a more ordinary explanation.
So, conspiracy theories and other similar hypothesis have low prior probabilities, even in the mind of skeptics. This is appropriate, as conspiracies are in fact very rare.
Secondly, these 'near-total interdependence of evidence' theories are designed to ignore the evidence. They are chosen precisely because they allow their adherents to say "but that's exactly what they want you to think!" to any evidence you bring against them. It's important to note that this is not an accidental, fortuitous property of these theories. 'Near-total interdependence of evidence' is the defining feature of such theories, and it's precisely that feature which allows them to dismiss all the evidence which would weigh against more likely theories.
In combination, the above two facts mean that such theories cannot really hope to win the day. Since they start with a low prior, and are designed for ignoring the evidence, they cannot really hope to prevail - they need evidence to increase that low prior probability, but they're designed mostly to ignore evidence.
Note that, when a conspiracy theorist ignores evidence by saying "that's exactly what they want you to think!", this doesn't actually help the theory. It merely turns a piece of evidence against the theory into no evidence. Yes, the conspiracy theory has "explained" the evidence, but only about as well as the rival theory. The Bayes factor therefore stays around 1, meaning nothing has changed on that front, and the probability for the conspiracy theory remains at its low prior value.
But, such evidence does still hurt they conspiracy theory, because the prior probability itself is now a lower value. A greater conspiracy that explains more - one that is more vast and has planted more evidence and covered it up better - is a priori less likely to have come about than a lesser conspiracy. So a piece of evidence that the conspiracy has to dismiss does still hurt the theory. The hope of the conspiracy theorist is that this harm in the prior probability will be less than the exponential rate of harm that a fully independent piece of evidence would normally cause.
So the most such a theory can realistically hope for is a kind of non-total loss, where they lose less quickly and hope to say "at least it's not impossible!" at the end.
Now, there are very particular kinds of evidence that does help them - the ones that specifically demonstrates a conspiracy. Something like a document from a secret meeting that lays out the nefarious master plan would work. But, of course, for a vast majority of these theories, such evidence does not exist.
So, given all these traits - given that they are highly unlikely theories that are designed to ignore the evidence, with little chance at any positive evidence for them - I think it's fair to call them crackpot theories.
Yet some people will bring up even such theories. So we, too, must now consider them, and show that our conclusion holds firm even then.
Chapter 10:
The "skeptic's distribution" approach
Using the historical data to construct the skeptic's distribution
How can we quantitatively tackle things like conspiracy theories? What do we do about the interdependency of evidence? One can already imagine the objections to any such attempt. Every assumption would be questioned, and every ridiculous possibility brought up demanding a full numerical treatment. Even if a traditional conspiracy were to be fully debunked in a numerical argument, a skeptic would just weasel the argument to be about a "groupthink induced by religious fervor" instead, and when that got debunked, they would just say "but what about aliens?" Indeed, such weaseling is often the point of bringing up things like conspiracy theories in the first place: not to actually advocate for them, but to make the calculation appear intractable.
But I did title this part "addressing all possible alternatives" - and that's exactly what I'm going to do. My argument will take EVERYTHING into account - government conspiracies, religious groupthink, practical jokes by aliens, everything. Furthermore, my approach will be fully quantitative. Every single possibility for every conceivable degree of evidence dependence will be fully considered.
In addition, empirical evidence will be the foundation of my whole argument. That is, in fact, the key that makes it totally comprehensive. Do you remember the following graph?

That is the level of empirical evidence that history has actually recorded for the resurrection of various individuals. It's a partial histogram - note the differing number of people with different amounts of evidence for their resurrection. This suggests a probability distribution.
Of course, the graph above isn't the complete record of everyone - it's a small sampling of some people who have the most evidence for their resurrection. But if we had a complete record, we could get a very accurate model for their underlying probability distribution. What would that probability distribution represent?
If we exclude Jesus and the other Christian resurrection reports, the probability distribution we get would be the EXACT model that an empirical skeptic of Christianity MUST use, in predicting the likelihood of a resurrection report. Essentially, the idea is that we can calculate the probability of getting a certain level of evidence for a resurrection, based on how frequently similar reports have come up in history.
Note that, because the raw data is gathered from empirical reports collected in history, this automatically accounts for things like conspiracy theories. The possible interdependency of the evidence is fully included in this model. So you think that a great deal of evidence can be built up through a conspiracy, because the evidence doesn't have to be independent? The distribution includes all such evidence-manufacturing conspiracies that actually existed in history. You want to switch your argument to a religious mass delusion instead? The result of all such mass delusions are also included, at the level of evidence that they actually generated in history.
How about something that has probably never happened in history at all, like some aliens faking a resurrection as a joke? Even these possibilities are included, through at least two mechanisms. For one, there are a great many multitude of such unlikely scenarios - and at least one of them might have actually occurred in history, even if a specific one of them was unlikely. So we would have a record of such evidence in aggregate. And secondly, even if such unlikely scenarios never occurred, they can still be accounted for in the modeling of the probability distribution from the samples we actually have. As an analogy, if you were to model people's heights by sampling a thousand people, you can still deduce that human heights follow a roughly normal distribution, and can thereby figure out that there would be someone out there who's at least 7 feet tall, even if such a person was not in your sample.
So you see, this method does in fact take everything into account. It does generate the exact model that an empirical skeptic of Christianity must use. That's the great thing about arguing from empirical, historical records. You can bypass all the difficult and controversial calculations about the probabilities of conspiracies, or the precise degree of dependence among the evidence. All of that automatically gets incorporated into the historical data at their actually correct historical values, and all we have to do is to read off the final result. So a skeptic cannot reject this probability distribution without rejecting history or empiricism.
Once we have this "skeptic's distribution", the rest of the calculation is fairly straightforward. We can calculate the probability of generating a Jesus-level of resurrection evidence, from the point of view of the skeptic's hypothesis. From a Christian perspective, this probability is within an order of unity, so the skeptic's probability then essentially becomes our Bayes factor. We then simply see if this Bayes factor is enough to overcome the low prior probability for a resurrection.
What should be the form of this "skeptic's distribution"?
How about we fit the "skeptic's distribution" to a normal distribution? Well, that would be plainly ridiculous. Even with very conservative estimates, the data we have so far gives Jesus 24 times more evidence for his resurrection than anyone else in history. Our goal is to get the probability of something like this happening.
But if we used a normal distribution for the "skeptic's distribution", this could essentially never happen. Recall that human heights roughly follow a normal distribution. Then, our problem would be analogous to looking for someone 24 times taller than anyone else in history - that is, someone well over 200 feet tall. The probability for something like that is essentially zero. So if we chose the normal distribution, we'd essentially be dooming the skeptic's case from the start.
The same is true for an exponential distribution. An exponential distribution decreases in its probability value by a constant factor for each unit of increase in its domain. As the domain for our problem is "level of evidence", this means that each piece of evidence would multiply the probability values. That is to say, we'd be treating each piece of evidence independently. And we already saw that, even with a reasonable degree of dependence factored in, the probability values reached numbers like 1e-48, again dooming the skeptic's case.
This is a testament to how quickly these distributions decay as they extend to the right. Their right tails are so "stubby" that the maximum values of their samples are strongly restricted, and getting something 24 times greater than that maximum is essentially impossible. Picking any such distribution would not be taking into account the dependence of the evidence, and would unfairly doom the skeptic's case from the start.
Rather, we need a distribution with a "long tail" - something that has a chance for a new high record to beat the previous record by factors like 24. Something that decays slowly enough that its probability values remain non-negligible as we move further to the right. The distribution should still be realistic and have some justification for being selected, but we want to give the skeptic the best chance.
Taking all that into account, I have chosen a power law function for the "skeptic's distribution". This should not be a surprise - indeed anyone familiar with the statistics of human behavior might have guessed it from just the previous graph of the histogram we're trying to fit.
What makes a power law particularly appropriate? Well, for one, power laws are the quintessential long-tailed distribution. They have one of the longest possible tails, and are fully "capable of black swan behavior", according to Wikipedia. They can easily have tails so long that the overall distribution has an undefined (that is, infinite) mean. In fact, power laws, as mathematical functions, can decay so slowly that it's not allowed to be a probability density function, because the area under their curve can diverge. One can hardly ask for a more slowly decaying function than that. So this gives the skeptic the best chance at naturally generating a Jesus-level of resurrection evidence.
There exists distributions that decay even more slowly than a power law, but they're rare, obscure, and have no relation to what we're doing. By contrast, power law distributions are ubiquitous in human behavior. They form the basis for the well-known Pareto principle, and they capture the "dependency of evidence" factor we're currently trying to model.
For example, the distribution of income among people follows a power law. A few people, out at the long tail, have a great deal of wealth, because rich get richer - that is, because how rich you get depends on how rich you already are.
The size of cities also follows a power law. There are a few very large cities out at the long tail, because your chances of moving to a city depends on the number of people who already live there.
The number of links to a website follows a power law. There are a few, very popular websites out at the long tail, which have a lot of links to them. This is because a site's chances of getting a link depends on its popularity - that is, on the number of links it already has.
Don't let the specificity of these examples fool you. There are many, many more. Power law distributions are, as I said, ubiquitous in human behavior. They will frequently come up when one human behavior depends on the same kind of behavior, either by others or by the same person.
So it is entirely appropriate that we use a power law to model the level of evidence for a resurrection report. There will be relatively few reports out at the long tail, like the "resurrection" of Apollonius or Krishna. In the context of things like conspiracy theories, this is because the chances of generating an additional piece of evidence depends on how much evidence it already has.
So there are excellent external reasons and examples to expect that the "skeptic's distribution" will follow a power law. Furthermore, power laws give one of the best possible chances for the skeptic's case, having a very "long tail" and allowing for a "black swan" event like the level of evidence in Jesus's resurrection event.
Details of the distribution: generalized Pareto distribution and its parameters
So we've decided on a power law as the general form of the "skeptic's distribution".
The details of the distribution near zero will not particularly matter. We're more concerned about how rapidly it decays at very large values. This allows us quite a bit of leeway in choosing the specific form of the power law distribution, as they all decay similarly as we move along the tail off to the right.
For this reason, I've chosen the generalized Pareto distribution as the specific form of the "skeptic's distribution", guided chiefly by the straightforward interpretation of its parameters. But the choice here will not affect any conclusions. Any other power law distribution would give the same results.
The generalized Pareto distribution is characterized by three parameters: location, scale, and shape. The location parameter determines where the distribution starts. It's where the probability density of the distribution is the largest. As the vast majority of humans have zero evidence suggesting that they rose from the dead, the location parameter should obviously be set at zero.
The scale parameter is irrelevant; it only controls how far the distribution should multiplicatively scale in the horizontal direction, and it can be arbitrary changed by changing the unit of evidence we use. As we'll consider all the evidence for our the resurrection reports relative to one another (for example, as a fraction of the amount of evidence for Christ's resurrection), the value for the amount of evidence in some specific units never enters the picture. So we'll just set this parameter to 1, or to whatever is convenient for visualization, and forget about it.
The shape parameter is the interesting one. It's what we really care about. It effectively determines the power in the power law, and controls how quickly the function decays as the amount of evidence increases.
For example, this is what the tail end of the distribution looks like with various shape parameters:
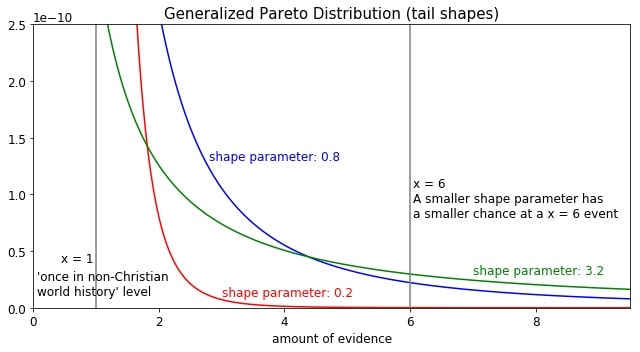
In each case, the distribution has been scaled so that the total probability to the right of the grey line at x = 1 is 1e-9. Essentially, x = 1 is where you would expect the maximum value out of 1e9 samples to appear, corresponding to the level of evidence for the resurrection of a figure like Apollonius or Aristeas.
Note the different rates decay. With the shape parameter at 0.2 (red curve), the probability density drops to practically zero as we move to larger x values. There is essentially nothing left by the time we've moved to x = 24, even if we integrate out to infinity. Therefore, if this were the final form of the "skeptic's distribution", the probability of generating a Jesus-level of evidence for a resurrection would be essentially zero.
However, with the shape parameter at 2 (blue curve), we see that the decay rate is much slower, and there is a good amount of probability even out at x = 24 and beyond. If this were the "skeptic's distribution", it would have a good chance of generating a Jesus-level of evidence for a resurrection, even if that level were 24 times higher than the runner-up.
A shape parameter of 20 (green curve) decays more slowly still. It's hardly decaying at all by the time it reaches x = 24 - it's nearly flat out there. In fact, it decays so slowly that the blue curve with the shape parameter of 2 will eventually move below it. If this were the "skeptic's distribution", it would have a non-negligible chance of generating an event at x values of much higher than 24.
But how should we determine the value of the shape parameter?
Well, what kind of data do we have to determine the shape parameter?
We have the historical data, of course. We have some number of people who are said to have been resurrected in some sense, and each of these people have some amount of evidence associated with their resurrection claim.
We essentially want to "fit" these evidence data into a generalized Pareto distribution, and read off the shape parameter. However, this will be somewhat tricky. We do not have the complete data for all 1e9 reportable deaths throughout human history. We can reasonably assume that the vast majority of them would have essentially zero evidence for a resurrection, but the complete data set would be pretty much impossible to obtain. We don't even have the complete data set just for the "outliers" - cases like Apollonius or Zalmoxis, where there is a distinctly non-zero level of evidence for a resurrection. Furthermore, the precision on determining the level of evidence is rather poor. All this means that the usual "fit a curve through some kind of x-y scatterplot" approach would not work very well.
However, given that we already know we'll be fitting a generalized Pareto distribution, this is not necessary. We're just looking for the shape parameter, and for that, we merely need to count the number of "outliers" near the maximum value. Consider the following graph:
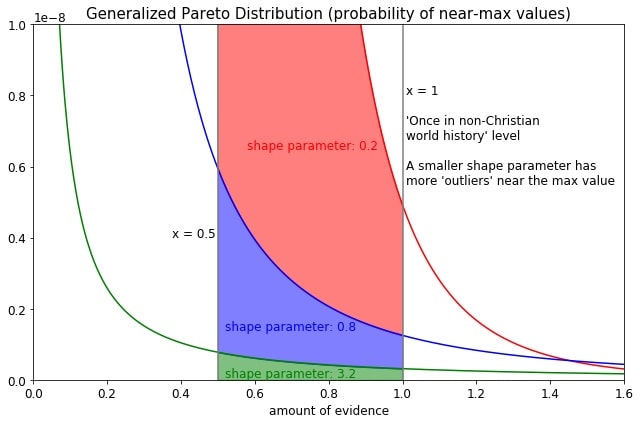
This is the same graph as before, in the sense that it just shows the generalized Pareto distribution, scaled so that the probability of x > 1 is 1e-9. Once again, this means that the maximum evidence from 1e9 reportable deaths is likely to appear around x = 1.
However, we now want to focus on how to fit the data. And since the data will have x values less than the maximum, this graph is scaled so that we're focusing to the left of the x = 1 line, instead of the tail to the right.
In particular, note the vast differences in the area under the curve for different shape parameters. The shaded regions represent the probability of finding an "outlier" - which we'll define as a non-Christian resurrection report with at least 20% of the evidence of the maximum report. For instance, the reports of the resurrection of Puhua or Apollonius would be considered an "outlier".
So, let's look at the green curve, with a shape parameter of 20, and a tiny area under the curve. If this were the skeptic's distribution, you'd expect essentially no other outliers. The area under the curve is too small. The maximum value would stand by itself, with no other outliers coming anywhere near its value.
Similarly, if the shape parameter is 2, the area under the curve is larger, and you'd expect more outliers. In fact, the area under the blue curve is roughly 1e-9, so you'd expect perhaps one outlier out of 1e9 samples.
Lastly, if the shape parameter is 0.2, you'd expect many, many outliers. The probability distribution grows very rapidly as it goes backward from x = 1, and therefore you expect to find many other resurrection reports with a similar level of evidence as the maximum.
So by counting the number of outliers, we can make a determination about the shape parameters.
But... wait a minute. Having more outliers is associated with smaller shape parameters? But didn't smaller shape parameters correspond to a faster-decaying function, and therefore a lower probability for the "skeptic's distribution" generating a Jesus-level of evidence? Wouldn't this lead to the "skeptic's distribution" being less able to explain the evidence for Jesus's resurrection, and therefore make the resurrection more likely?
Are we saying that having MORE non-Christian resurrections reports make Jesus's resurrection MORE likely?
That is precisely what we are saying.
More non-Christian resurrections reports make Jesus's resurrection more likely
One way to see that is just from the above graph - the more probability there is with x < 1, the less there must be at higher values of x, like x = 24. This means that the "skeptic's distribution" is less able to explain an event with x = 24, so Christ's resurrection becomes more likely.
The following analogy may help understand this in a more intuitive way.
Alice accuses Bob of theft. Bob is known to have come into a sudden possession of $100,000. He is also known to be a gambler. He claims that his sudden fortune came from a lucky night at the card table, but Alice believes that he stole the money - she claims that $100,000 is far too large a sum for Bob to have naturally won through gambling.
Carol takes on this investigation. She looks into Bob's past gambling history, to see if it's realistic for him to have won $100,000 in a single night. She finds that, among Bob's past verifiable winnings, there were two nights where he won $5,000 and $3,000. These are his most remarkable winnings on record, and Carol cannot find any other instances where he won more than $1,000 on a single night.
Carol concludes that she does not really have enough information. It could be that Bob plays a card game with an erratic payout scheme, where winning 20 or 30 times more money is not that unusual. Maybe it has some kind of "let it ride" or "double or nothing" mechanism which makes such returns plausible. Or maybe Bob himself is an erratic gambler, and decided to bet a lot more money than usual on the night when he supposedly won $100,000. Based on all this, Carol decides to be skeptical of Alice's claim that Bob stole the money. Her own "skeptic's distribution" for how much money Bob can win does not decay quickly enough. There are relatively few outliers near his maximum winnings of $5,000, and this suggests that it decays very slowly - meaning that the $5,000 cannot be established as a limit to what Bob can win. His theoretical winnings might possibly stretch out quite far into the higher values, making it impossible to rule out a $100,000 winning.
But then, Carol has a breakthrough in her investigation. She finds extensive, previously undiscovered records of Bob's gambling winnings, and it shows that Bob has won more than a $1,000 on dozens of nights. The maximum that he's won is still $5,000, but he's also regularly won thousands of dollars in a single night.
Carol takes this new information into account, and adjust her "skeptic's distribution" for how much Bob can win in a single night. Clearly, Bob's winnings are not erratic; he regularly wins up to about $5,000. But this also establishes, with the weight of those repeated winnings, that this is close to the likely upper limit for what he can win in one night.
Carol therefore decides to believe Alice. Her "skeptic's distribution" cannot explain how Bob would naturally win $100,000 in a single night, because it goes against his established pattern of regularly winning up to $5,000. She pursues the case further, and eventually convicts Bob of theft.
This is not just a story; it can be mathematically established, and we will do just that very shortly. But for now, this story just provides the intuitive backing for the mathematical results to come.
So, having more non-Christian reports of a resurrection, with their pathetically low levels of evidence behind them, only make Jesus's resurrection more likely. When skeptics say "don't you know there are numerous other Jesus-like stories of someone dying and resurrecting?", they are only kicking against the goads. The more such cases they come up with, the more firmly it establishes that Jesus really did rise from the dead.
Chapter 11:
Calculation and confirmation using the "skeptic's distribution"
The calculation plan: obtaining and using the "skeptic's distribution"
Let us bring together everything we've said thus far about the "skeptic's distribution", and lay out how we'll approach this calculation.
We assume that the "skeptic's distribution" will take the form of a linear combination of generalized Pareto distributions, distributed over different possible shape parameters.
We will then determine that distribution over the shape parameters by looking at how many "outliers" exist in history.
A person's resurrection report is considered an "outlier" if it has at least 20% of the maximum evidence among the non-Christian resurrection reports.
The "maximum evidence among the non-Christian resurrection reports" is taken to be that of Krishna or Aristeas, with Apollonius not too far behind. These are taken as having 1/24 of the evidence for the resurrection of Jesus.
Recall that the "some people say... " level of evidence - as per Puhua, Osiris, Zalmoxis, etc. - corresponded to 1/60th of the evidence for Christ's resurrection. This is 40% of 1/24, meaning that anyone with the "some people say..." level of evidence for their resurrection would comfortably pass the 20% threshold for an "outlier".
So then, the number of outliers determines the distribution over shape parameters, which determines the "skeptic's distribution", which determines the probability of it naturally generating a Jesus-level of evidence for a resurrection.
We will write a computer program to do all this. Here are the specs for the program.
We will consider shape parameters from 0.02 to 2.1, in increasing intervals of 0.02. That is to say, we will consider shape parameters 0.02, 0.04, 0.06, etc. all the way up to 2.1. Our region of interest will lie in this range.
We will create a generalized Pareto distribution with that shape parameter, then simulate drawing the maximum value of 1e9 samples from that distribution. We will then estimate the number of outliers in that distribution, and the probability of that distribution generating a sample more than 24 times larger than the maximum.
We will do this 10000 times for each value of the shape parameter. This gives us a table with a little more than a million rows, with each row containing the shape parameter, the number of outliers, and the probability of generating a Jesus-level of evidence.
If we assume equal prior weights for each of the shape parameters, we can consider the final, posterior distribution of the shape parameters to be just its distribution from the subset of the table where the number of outliers is equal to the actual, historical value. That is to say, we just have to look at where the theory fits the data, and consider only those theories. This satisfies Bayes' theorem, as we're effectively just using a hierarchical Bayes model.
Likewise, the probability of the "skeptic's distribution" achieving a Jesus-level of evidence for a resurrection will be just the mean value of that probability from the same subset.
The final output of the program will be the probability of the "skeptic's distribution" generating a Jesus-level of evidence for a resurrection, given the number of historical "outliers" as an input.
All of the assumptions and choices made above favors the skeptic's case. Therefore, the probability obtained at the end will be a maximum probability; the skeptic cannot hope for more than that in explaining Jesus's resurrection "naturally".
Simulation and code: The number of "outliers" decides the case.
This is a jupyter notebook. It contains the python code which generates the relationship between the number of "outliers" (as previously defined) and the probability of naturalistically generating a Jesus-level resurrection report.
%matplotlib inline import numpy as np import pandas as pd from scipy.stats import genpareto
def max_out_of_n_from_dist(dist, out_of_n=1e9):
manageable_n = 100000
if out_of_n <= manageable_n:
return dist.rvs(out_of_n).max()
else:
top_percentiles =
np.random.rand(manageable_n) * manageable_n / out_of_n
return dist.isf(top_percentiles).max()
We then simulate getting the maximum value out of 1e9 samples drawn from these distributions. We next calculate how many "outliers" would exist given that maximum value and that distribution. Lastly, we calculate the probability of drawing a sample whose value is 24 times greater than the maximum value. This is the probability of "naturally" generating a Jesus-level resurrection report for that distribution.
We repeat this 10000 times for each of the 105 shape parameters between 0.02 and 2.1, and put it all in a table. The result is a table with 1050000 rows, whose columns are the shape parameter, the number of outliers, and the probability of drawing a sample 24 times greater than the maximum.
The following code gives us this results table.
sample_size = int(1e9)
genpareto_shapes = np.linspace(0.02, 2.1, 105)
shape_params = []
prob_24max = []
n_outliers_estimation = []
for shape_param in genpareto_shapes:
dist = genpareto(shape_param, scale=1, loc=0)
for i in range(10000):
shape_params.append(shape_param)
max_val = max_out_of_n_from_dist(dist, sample_size)
prob_24max.append(dist.sf(max_val * 24))
p_outlier = (dist.sf(max_val * 0.2) - dist.sf(max_val))
/ dist.cdf(max_val)
n_outliers_estimation.append(
int(round(p_outlier * sample_size)))
genpareto_results_df = pd.DataFrame({
"shape_params":shape_params,
"prob_24max":prob_24max,
"n_outliers":n_outliers_estimation,
})
#save to .csv, as generating this takes a while
genpareto_results_df.to_csv(
"genpareto_results_df.csv", encoding="utf-8")
genpareto_results_df = pd.read_csv(
"genpareto_results_df.csv", encoding="utf-8"
).drop("Unnamed: 0", 1)
print genpareto_results_df.shape genpareto_results_df.head()
(1050000, 3)
| n_outliers | prob_24max | shape_params | |
|---|---|---|---|
| 0 | 6579077 | 1.547543e-57 | 0.02 |
| 1 | 7069940 | 3.123026e-57 | 0.02 |
| 2 | 6141769 | 7.974702e-58 | 0.02 |
| 3 | 6608791 | 1.616688e-57 | 0.02 |
| 4 | 3459940 | 4.204358e-60 | 0.02 |
How could we get this posterior distribution of the shape parameters? All we need to do is to take the subset of the results table where the number of outliers is exactly 10, and look at the shape parameters. This satisfies Bayes' theorem, and gives us a posterior distribution that looks like this:
genpareto_results_df[genpareto_results_df["n_outliers"] == 10]
["shape_params"].hist(bins=99).set_xlabel("shape parameters")
genpareto_results_df[genpareto_results_df["n_outliers"] == 10]
["prob_24max"].mean()
8.840657596227429e-11
Of course, there's almost certainly more than 10 "outliers" in world history. So this will not matter for our actual calculation. But it does show us that we have to be careful about bumping into the edges of our range of shape parameters. We also have to worry about the decay rate of the distribution over shape parameters, as it goes off to the right. Thankfully, it turns out that it decays quickly enough that we can simply ignore it after a certain point. The probability density beyond a shape parameter of 2.1 is negligible once we go a little bit beyond 10 "outliers". Demonstrating this is left as an exercise for the reader.
genpareto_results_df[genpareto_results_df["n_outliers"] == 50]
["shape_params"].hist(bins=100).set_xlabel("shape parameter")
genpareto_results_df[genpareto_results_df["n_outliers"] == 50]
["prob_24max"].mean()
4.428207171511614e-12
genpareto_results_df[genpareto_results_df["n_outliers"] == 250]
["shape_params"].hist(bins=100)
genpareto_results_df[genpareto_results_df["n_outliers"] == 250]
["prob_24max"].mean()
1.5911343547015349e-13
outliers_p24max = genpareto_results_df[
genpareto_results_df["n_outliers"] < 100
].groupby("n_outliers")["prob_24max"].mean()
outliers_p24max.reset_index().plot(
kind="scatter", x="n_outliers", y="prob_24max",
xlim=(0,100), ylim=(0, 2e-10)
)
Looking at the rest of the graph, we see that the probability of generating a Jesus-level resurrection report drops as the number of "outliers" increases. Having MORE non-Christian resurrection reports (that is, having more "outliers") makes the skeptic LESS able to explain Jesus's resurrection, and therefore makes it MORE likely - exactly as we said before.
So, the question now just comes down to this: how many "outliers" can we find in world history? Recall that anyone with a "some people say..." level of evidence for their resurrection counts as an outlier. The more such people we can find, the more firmly Christ's resurrection is established. Can we find enough such people to overcome the low prior probability against a resurrection?
The list of outliers. These put the chance of Christ's resurrection over the top.
Aristeas (ancient Greek Poet)
Apollonius of Tyana (ancient Greek philosopher)
Krishna (Hindu god)
Zalmoxis (ancient Getae god)
Osiris (ancient Egyptian god)
Dionysus (ancient Greek god)
Bodhidharma (Buddhist monk)
Puhua (Buddhist monk)
Horus (ancient Egyptian god)
Ba'al (Canaanite god)
Melqart (Phoenician god)
Adonis (ancient Greek god)
Eshmun (Phoenician god)
Tammuz (Sumerian god)
Ishtar (Sumerian goddess)
Attis (Phrygian god)
Quetzalcoatl (Aztec god)
Parashurama's mother (character in Hinduism)
Sisyphus (character in Greek mythology)
Pelops (character in Greek mythology)
Persephone (ancient Greek goddess)
Asclepius (ancient Greek god/healer)
Hippolytus, son of Theseus (character in Greek mythology)
Achilles (ancient Greek hero)
Memnon (ancient Greek hero)
Castor (character in Roman mythology)
Alcmene (character in Greek mythology)
Heracles (ancient Greek hero)
Melicertes (character in Greek mythology)
Romulus (mythic founder of Rome)
Cleitus (character in Greek mythology)
Cycnus, king of Kolonai (character in Greek mythology)
Cycnus, friend of Phaethon (character in Greek mythology)
Odin (Norse god)
Augustus (Roman emperor)
Peregrinus Proteus (ancient Greek philosopher)
Rabbit Boy (character in native American mythology)
Arrow Boy (character in native American mythology)
Man-eagle (character in native American mythology)
Judah the Prince (Jewish rabbi)
Sabbatai Zevi (Jewish rabbi, messiah claimant)
Kabir (Indian mystic poet)
Calybrid and Calyphony (characters in Celtic myth)
Muisa (character in a Nyanga epic)
People of Tubondo (characters in a Nyanga epic)
Hebo (Chinese god)
Li Tieguai (Chinese immortal)
Zhang Guolao (Chinese immortal)
People resurrected by Zhongli Quan (associates of a Chinese immortal)
Ye Fashan (Chinese immortal)
I'm going to stop here - not because I've exhausted such "outliers", but because this is quite enough. The above list contains 50 people (or groups of people) who are claimed to have been "resurrected" in some form, with about a "some people say..." level of evidence behind them. And as we saw previously in the jupyter notebook, 50 outliers is enough to reduce the probability for a naturalistic explanation to around 4e-12.
Recall that the Christian explanation for the level of evidence for Christ's resurrection is of order unity. The ratio between that and the above probability of 4e-12 is the Bayes factor for the Jesus-level of evidence, which in this case turns out to be above 1e11. Combining that with the prior of 1e-11 against the resurrection gives a number bigger than 1e0. That is, Jesus's resurrection has better than even odds of having occurred.
Notice that the procedure up to this point outlines the worst case scenario for the resurrection. For example, I stopped the above list of "outliers" at 50 only because it was getting tedious to write more. Each member of the above list was obtained with just a little bit of effort, mostly from stories that are readily available online, accessible to a culturally western, English-speaking audience. If you enjoy studying mythologies, you'll often just run into such stories without even trying. How many such "outliers" are there in total, throughout all of world history? How many stories where "some people say..." that someone rose from the dead? I would easily imagine it to be in the hundreds, if not thousands.
So, "better than even odds" is the absolute minimum that can be said for Christ's resurrection. Next, we will go back over the procedure that got us to this point, and demonstrate that the worst case scenario for the resurrection had in fact been assumed at every point.
We were far too generous for the "skeptic's distribution"
We have established that the resurrection has, at a minimum, even odds of having taken place. Let us retrace our steps and demonstrate that this is, in fact, the minimum.
The power law distribution
Looking back, we see that our first decision was to choose a power law as the form of the "skeptic's distribution". As we mentioned when we made the choice, this is the most pro-skeptical choice we can make which still fits the facts. Power law distributions have one of the longest possible tails, which can decay very slowly. They're fully capable of a "black swan" event. Furthermore, they're ubiquitous in human behavior, in that they're naturally generated when an increase in a value depends on the value itself. For this reason, the distributions of personal wealth, city sizes, and website popularity all follow a power law distribution. It's therefore appropriate to use it to model the buildup of evidence through possibilities like conspiracy theories or religious mass delusions.
However, there are excellent reasons to believe that the true "skeptic's distribution" will die off more quickly than a power law distribution, especially when we extend it to 24 times the maximum observed value. You see, few real-life power law distributions can actually extend off to infinity. Some external factor will intervene to cut off the distribution at very large values.
Consider city sizes, which we just mentioned. The population of cities follows a power law, and this holds up pretty well as long as we consider populations up to tens of millions of people. However, if we try to extend this out to infinity, the distribution no longer holds. We run into external factors which limit city sizes, such as the total population of humanity or the logistics of city growth in a given geography. For example, the largest city in South Korea is Seoul, with about 10 million people. A city 24 times larger than that would have over 200 million people - much larger than the total population of South Korea, which is only 50 million. Such a South Korean city cannot exist - not because its probability would be too small according to the power law distribution, but because it runs into external factors, like the fact that a city cannot be larger than the country to which it belongs. That is to say, the power law distribution for city sizes is limited, or cut off, at the long tail.
You can imagine similar arguments for personal wealth and website popularity. An individual cannot actually have "all the money in the world", and a website cannot be linked from more websites than the number that actually exist. And even far before such limits, sociological effects will likely truncate the distribution's long tail. Likewise, naturalistically generated evidence for resurrection stories cannot follow a power law distribution out to infinity. Other, external factors will cut off or strongly attenuate the probability as such resurrection stories gains more momentum.
For this reason, the true "skeptic's distribution" is almost certainly something that looks like a power law over the actually existing samples, but decays more quickly thereafter. A number of distributions - like a log-normal distribution or a power law with an exponential cutoff - follow this behavior, and are also very common in human behavior. In each case, these other distributions with their "shorter" tails would help the case for Christ's resurrection. So adopting a generalized Pareto distribution, which is a genuine power law all the way out to infinity, was therefore the most pro-skeptical choice we could have made.
The uniform distribution over shape parameters
Next, we considered evenly-spaced shape parameters, in intervals of 0.02, for our distribution. That is to say, we chose a uniform distribution over the shape parameter as our prior. Again, this almost certainly unduly favors skepticism. Consider what such a prior distribution means: the true shape parameter would be 1000 times more likely to be between 1000 and 2000 than between 0 and 1. It would be infinitely more likely to be greater than 1 than to be less than 1. Remember that a larger shape parameter favors the skeptic's case, and we have chosen a prior that favors these larger values. It is only through the weight of the evidence that this prior distribution gets reigned in, but choosing such a biased prior still biases the end results.
A more common and reasonable choice of prior in such circumstances is to consider shape parameters which increase linearly in their logarithms. For example, we may consider shape parameters like 0.01, 0.1, 1, 10, 100, and so on. The idea is that we don't know what the order of magnitude of the shape parameter would be, and therefore consider each order of magnitude equally. Of course, such a prior favors the smaller shape parameters compared to the uniform distribution that we actually used, meaning that it helps the case for the resurrection. So once again, our choice of evenly-spaced shape parameters was the most pro-skeptical choice we could have made.
The sample size, in the number of reportable deaths
Next, we considered the maximum value of 1e9 samples drawn from our "skeptic's distribution". That value of 1e9 was chosen as the number of "reportable deaths" in world history. That is, this is the number of deaths that had a chance to be witnessed, documented, or told about in a story. It excludes those deaths where nobody could have made a statement about that death, even if a genuine resurrection took place.
But a moment's reflection shows that this number is too small. Only 1e9 - one billion - "reportable deaths" in world history? More people than that have died just in the last century, and virtually all of these deaths have been "reportable" according to the definition above. Surely a more realistic figure would easily be above 1e10.
This is important, because this value effectively sets an upper bound on the probability of generating a Jesus-level resurrection report. A report with the most evidence out of 1e9 samples has a probability of about 1e-9 of being generated. The most evidence out of 1e10 samples would correspondingly have a probability around 1e-10. We are then calculating the chances of generating a report with 24 times more evidence.
It's clear that the larger the number of samples, the smaller the probability of generating a report with a level of evidence comparable to the maximal sample. The probability of beating that by a factor of 24 is smaller still. So, the more samples we use, the smaller the probability for the "skeptic's distribution" generating a Jesus-level resurrection report. In other words, using 1e9 as the number of "reportable deaths" was a pro-skeptical choice. The true value is definitely much larger - easily above 1e10. And using this true value would only strengthen the case for the resurrection.
The quality of the other resurrection reports
Next, consider the factor of 24 that we used, as the ratio between the level of evidence for Jesus's resurrection, and that of the runner-up. This, too, was a very conservative estimate, which favors the skeptic's case.
You'll recall that the runners-up were Aristeas and Krishna, with Apollonius falling not too far behind. In our previous review of each of these cases, I noted that I was being quite generous in granting them their level of evidence. I felt that there was essentially no evidence, but wanted to express the fact that at least someone had said something - so I somewhat arbitrarily assigned the "some people say..." level of evidence as 1/10th of a single, solid, historic person's sincere testimony. Then, the three people named above got a multiplier on top of that to account for some additional details.
Of course, this is an overestimate of the "some people say..." level of evidence. Would you believe ten people with "some people say..." stories, over a single person who's giving a sincere, personal testimony? Let's consider some thought experiments. What would you think of ten people who say, "a friend of a friend whose uncle works for the US government tells me that some people say that the president has been contacted by aliens"? How would that compare with a single witness who sincerely and consistently says "I was there when the aliens contacted the president. It really happened"? If you were a journalist, which source would you cite in your article? If you needed more information, who would you talk to?
Another way to see that the "some people say..." level of evidence is relatively worthless is to observe how common it is. Indeed, this is tied in with the fact that the great number of such evidence throughout history works as evidence FOR the resurrection. We have seen that there is at least 50, and likely hundreds or thousands of such "some people say..." reports for a resurrection. In contrast, none of these non-Christian accounts has even a single, historical person claiming that they personally witnessed the resurrection. This speaks to the relative worth of a single personal testimony over hundreds or thousands of "some people say..." reports.
All told, the level of evidence for Jesus's resurrection is far greater than 24 times that of the runner up. Using 24 as the factor is a very conservative, pro-skeptical choice.
The number of non-Christian outliers
I've also touched upon the number of "outliers" - the number of resurrection reports with a "some people say..." level of evidence. I've cited 50 such reports, and have used 50 in the calculation as the number of outliers. But as I mentioned, this is a vast underestimate. It comes from a very limited subsample of all the stories in world, mostly reachable by just a few minutes of online research in English. The true number of such outliers could easily be in the hundreds or thousands. So this, too, was chosen to favor the skeptical case.
The region of integration
There's still more. Note that, for the "skeptic's distribution", we've integrated out to infinity to get the probability of it explaining the level of evidence for Jesus's resurrection. Strictly speaking, this is an improper way to calculate the Bayesian likelihood. The correct way would be to calculate the probability for the "skeptic's distribution" getting the ACTUAL level of evidence for Jesus's resurrection, rather than calculating the probability of it EXCEEDING that level of evidence. Of course, doing it correctly makes the likelihood smaller, because you're giving up all of that probability out to infinity, in the long tail.
The Christian hypothesis must face the same treatment, of course. So its Bayesian likelihood would also drop. But this will not as detrimental as it is for the "skeptic's distribution", because the Christian hypothesis more narrowly focuses the distribution of evidence around the actual value. That is to say, if Jesus did really rise from the dead, it's quite likely for that to have left the amount of evidence that we actually find, while there's good reasons for it to be not that much greater. On the other hand, a long-tailed "skeptic's distribution" would extend on out to infinity - with the consequence that it must pay when the band narrows to the actual amount of evidence we have.
You can argue that the "skeptic's distribution" need not extend on out to infinity - but that just means you're arguing that it doesn't follow a power law, instead following another distribution with a stubbier tail. So either the "skeptic's distribution" does worse because it's actually a "stubbier" distribution than a power law, or because it loses its probability mass when we force it to focus around the amount of evidence we actually have in history. In any case, our previous calculation was one that favored the skeptic's case.
A better estimate of the probability
So we see that "even odds" for Jesus's resurrection is not really even the minimum - it's an impossibility. It's a value derived by severely discounting and ignoring huge realms of evidence for the resurrection, while granting the skeptic's case all reasonable (and some unreasonable) allowances. The actual odds would be far more favorable towards Christianity.
Let us run some the calculations to get an estimate of these "actual odds".
The simulation and code, revisited with more likely values
This is another Jupyter notebook. It contains python code that generates the probabilities of a "skeptic's distribution" generating a Jesus-level resurrection report.
import numpy as np import pandas as pd from scipy.stats import lognorm, genpareto
def max_out_of_n_from_dist(dist, out_of_n=1e9):
manageable_n = 100000
if out_of_n <= manageable_n:
return dist.rvs(out_of_n).max()
else:
top_percentiles =
np.random.rand(manageable_n) * manageable_n / out_of_n
return dist.isf(top_percentiles.min())
def calculate_p_skeptic(
dist_type, #genpareto or lognorm
shape_params_dist, #np.logspace or np.linspace
sample_size, #1e9 or 1e10
greater_by, #24 or 50
n_outliers, #50 or 250
n_max_draws=10000,
):
if dist_type == genpareto:
shape_limits = [0.02, 2,1]
elif dist_type == lognorm:
shape_limits = [0.2, 10.0]
min_shape = 0.2,
max_shape = 10.0
if shape_params_dist == np.logspace:
shape_limits = [np.log10(x) for x in shape_limits]
shape_params_list = shape_params_dist(
shape_limits[0], shape_limits[1], 105)
shape_params = []
p_max_greater_by = []
n_outliers_estimation = []
for shape_param in shape_params_list:
dist = dist_type(shape_param, scale=1, loc=0)
for i in range(n_max_draws):
shape_params.append(shape_param)
max_val = max_out_of_n_from_dist(dist, sample_size)
p_max_greater_by.append(dist.sf(max_val * greater_by))
p_outlier =
(dist.sf(max_val * 0.2) - dist.sf(max_val))
/ dist.cdf(max_val)
n_outliers_estimation.append(
int(round(p_outlier * sample_size)))
result_df = pd.DataFrame({
"shape_params":shape_params,
"p_max_greater_by":p_max_greater_by,
"n_outliers":n_outliers_estimation,
})
match_df = result_df[result_df["n_outliers"] == n_outliers]
if match_df.shape[0] < 50:
print "warning: match_df.shape = ", match_df.shape
if match_df["shape_params"].max() == shape_params_list.max():
print "warning: maxed out shape_param"
p_skeptic = match_df["p_max_greater_by"].mean()
return p_skeptic
calculate_p_skeptic(
dist_type=genpareto,
shape_params_dist=np.linspace,
sample_size=int(1e9),
greater_by=24,
n_outliers=50,
)
4.139635934580683e-12
The prior distribution of the shape parameters: from being uniform in linear space to uniform in logarithmic space.
The sample size (that is, the number of reportable deaths in world history): from 1e9 to 1e10.
The number of "outliers" (That is, the number of reports of a "resurrection", with at least a "some people say..." level of evidence): from 50 to 200.
All of these changes are almostly certainly true to at least that extent. The actual truth is probably even more extreme - for example, the number of outliers may actually be in the thousands.
This gives a very conservative answer for how small the "skeptic's probability" may be.
calculate_p_skeptic(
dist_type=genpareto,
shape_params_dist=np.logspace,
sample_size=int(1e10),
greater_by=24,
n_outliers=200,
)
1.866911316237698e-14
calculate_p_skeptic(
dist_type=lognorm,
shape_params_dist=np.logspace,
sample_size=int(1e10),
greater_by=50,
n_outliers=300,
)
3.855529808259304e-16
calculate_p_skeptic(
dist_type=lognorm,
shape_params_dist=np.logspace,
sample_size=int(3e10),
greater_by=70,
n_outliers=200,
)
6.095046577090992e-17
So, those are some of the possible values for the "skeptic's probability". We see that the "skeptic's probability" of 4e-12 was an impossible best-case scenario for skepticism. A very conservative - but not fantastical - value would be more like 1e-14, and a likely scenario gives values like 1e-16.
So, is that it? A Bayes factor of 1e14 to 1e16 results in a probability of 99.9% (conservative) to 99.999% (likely) for the resurrection. Is that our final value?
Not at all. Notice that throughout this "skeptic's distribution" argument, I've never brought up Paul's independence from the rest of the disciples? This is a huge point in favor of the resurrection which has been simply ignored. Let us next address this point, and others like it.
Chapter 13:
Defenses against crackpot theories
The pro-resurrection arguments we have yet to consider
Remember that this whole time, we've only been considering the summary account mentioned in 1 Corinthians 15. This severely discounts the weight of evidence for many people (John, for example, should be counted more like Peter than just a member of the Twelve), and doesn't take some groups of people (like the women at the tomb) into account at all.
On top of that, our entire argument about the "skeptic's distribution" only takes the AMOUNT of evidence into account. It argues that no possible effect - not even the ones with a near-total dependence in the evidence (e.g. conspiracy theories) - could falsely generate the amount of evidence for Jesus's resurrection.
Of course, Jesus's resurrection has more than just the sheer AMOUNT of testimonies going for it. We now have to consider the specific properties of these testimonies which counters hypotheses like conspiracy theories. This will further strengthen the evidence for Christ's resurrection, beyond the evidence from the mere amount of testimonies.
Recall that nearly all of the remaining possibility for the skeptic was in crackpot theories like conspiracies. Without such theories, their probability values drop to ridiculous numbers like 1e-43. So these specifically anti-crackpot properties in the resurrection testimonies are a direct body blow against the skeptic's remaining case. They apply nearly their entire weight against the remaining probability for the skeptic.
So we expect these anti-crackpot properties to significantly shift the final odds. Let us examine them one by one.
Defenses against crackpot theories built in to Christianity
Could the resurrection testimonies really have a near-total dependency among them? Could they have been generated by a conspiracy of some sort? There are a multitude of reasons to think they were not.
Apostle Paul
First, there is the story of apostle Paul, whose independent nature we've already discussed up in "the basic argument" section. He's one of the named witnesses in 1 Corinthians 15, and someone who first started out as a zealous persecutor of Christianity. He is then supernaturally converted by literally seeing the light on the road to Damascus, and becomes Christianity's most effective evangelist. How many conspiracies have something like that in their narrative?
Now, the conspiracy theorist can still say "that was obviously a part of the plan! You've been taken in by their trap!" After all, that's precisely what a conspiracy theory is designed to do. But as I said before, while this allows them to "explain" apostle Paul by keeping the Bayes factor for his testimony to around 1, it is still a significant blow against a conspiracy theory. For a conspiracy that has planned for such a conversion is a priori far less likely than one that has not. In postulating a deeper, vaster, and more comprehensive conspiracy, the theorist has postulated a less likely one.
In fact, Paul's conversion is so unlikely that it's probably enough by itself to debunk the most common types of crackpot theories. Ascribing Paul's actions to a conspiracy is like planning to punch a stranger in the streets in hopes of making money from the ensuing lawsuit, or asking a politician to concede an already won election to their bitter rival solely out of respect and goodwill. The odds of success for such schemes are long indeed. Humans just don't work that way.
Apostle James
But we're just getting started. Let's look at James - the biological brother of Jesus, and another of the named witnesses in 1 Corinthians 15. Consider his relationship with the rest of the early Christian movement. Earlier on in his ministry, there is good reason to think that there were some strained relationships between Jesus's family and his disciples. And yet, after the resurrection James is considered one of the chief disciples, and is named as a witness to Christ's resurrection. Could this have been the result of a conspiracy?
Unlikely. Family members and professional associates often form disparate circles, and it would be an additional obstacle for a conspiracy to grow to encompass that divide, especially if it involves an estranged family member. Of course, you can postulate that James was in on the plan from the beginning - but you're again just postulating a bigger, and therefore a less likely, conspiracy.
So there is already a great deal of independence for Paul and James from the rest of Jesus's disciples, which include Peter. So our three named witnesses in 1 Corinthians 15 are quite unlikely to be dependent, and therefore their testimonies are unlikely to be the product of a conspiracy.
The diversity among the 12 disciples
But the independence of the witnesses don't stop here. The twelve disciples may be thought of as a fairly interdependent group - after all, they were twelve Jewish males who all followed one leader. But looking into their background reveals a good amount of diversity. Some of them were fishermen - but their number also included, at a minimum, a tax collector (working for Rome) and a zealot (revolutionaries working against Rome). It's not easy to come up with three groups that would have gotten along less with each other than a tax collector, a zealot, and a regular Jewish worker, like a fisherman. Could a conspiracy rise from such a group? Again, it's not impossible, but it's also not likely.
The diversity among the earliest converts
The diversity is further magnified in the earliest converts to Christianity, at the Pentecost. According to Acts 2, these people were from all over the known world. Many of them did not even consider Hebrew, Aramaic, or Greek to be their native tongue. Again, could a conspiracy spread out so quickly to such a diverse group, as the very first people to be taken in? It must have been a very flexible and compelling conspiracy indeed - and therefore a very unlikely one.
The inclusion of women
Lastly on the point of diversity, there are of course the women. They go unmentioned in the public declarations of 1 Corinthians 15, because women were not considered reliable witnesses in the 1st century Jewish society. Yet they are featured prominently in the actual narrative in all of the gospels - as the group that did not abandon Jesus at the cross, and the first witnesses to the risen Christ. What kind of conspiracy does this? Why have the first witnesses to the resurrection be a class of people the society considers unreliable? Why include them in the story at all, if you're not going to publicly mention them among the chief witnesses?
If it's all true, then this all makes sense. But as a conspiracy theory, each one is a mystery. One can construct a conspiracy theory that fits all this, but such a conspiracy would be a rare one indeed, and highly unlikely a priori.
The divisions in early Christianity
So the diversity of the individuals involved in Christ's resurrection testimonies already make a high degree of interdependence unlikely. One could hardly find a less likely group of people to enter into a world-spanning conspiracy. You would expect disparate parts of such a group to be constantly at odds with each other, destroying the conspiracy almost immediately.
In fact, that's pretty much what happened: the disparate parts of the group were constantly at odds with each other - and yet, the "conspiracy" was preserved.
There were hints of confusion and division even before Jesus's crucifixion, in things like the disciples arguing about who will be the greatest, or who will be sitting by Jesus's side when he establishes his kingdom. Peter even berated Jesus for announcing his upcoming death, and there seems to have been a general confusion about the nature of the movement - are they going to lead an uprising against Rome? Do they need to be armed?
After Christ's ascension, very early in the book of Acts, there was a conflict between the Greek-speaking members and the other Jewish members of the Church, concerning the equitable distribution of food to the widows. This was a big enough deal that the Church instituted a whole new tier of leadership - the first deacons - to address the issue. And yet, the central tenant of the "conspiracy" - the resurrection - was unchanged.
Persecution and further division
Soon thereafter, an intense persecution befell the church. Several key members were killed, and the church was scattered across the known world. Gentiles were first evangelized around this time as well (Cornelius, Ethiopian eunuch) - which in itself caused no small amount of controversy. All of this further fragmented an already very diverse church. The problem was so bad that various evangelists regularly encountered people with very incomplete knowledge about Jesus. There was a group who did not know about the baptism of the Holy Spirit, and Apollos had to have his knowledge completed by Priscilla and Aquila. Still others were only attracted to the power associated with the name of Jesus and wanted to misusing it outright, like Simon Magus and the seven sons of Sceva. And despite all this persecution, fragmentation, and confusion, the "conspiracy" held together.
In the middle of all this, Paul - already mentioned as one of the early persecutors of the church - miraculously converted to Christianity, and became one of its foremost evangelists, to the point of becoming one of the named witnesses in 1 Corinthians 15. He then got embroiled in the central controversy of the early Christian Church: how to handle the new Gentile believers. This controversy got so heated that Paul once had to publicly rebuke Peter for his stance, and James wrote his epistle with a vastly different emphasis from Paul on what it means to truly be a "believer". In other words, this controversy set all three of the named witnesses in 1 Corinthians 15 against one another, to some degree. And yet, the "conspiracy" endured.
And that's not the end to the divisions of the early church - A number of outright heretical groups had to be condemned - Paul pronounced anathema to a group proclaiming "a different gospel", and John pointed out certain "antichrists" at large in the world, and also named the works of the Nicolaitans as the objects of Jesus's hate. And despite all this division, the "conspiracy" remained.
Again, what kind of conspiracy does this? What conspiracy kills off its leader, fragments itself into dozens of different factions, bitterly fights itself on internal controversies, condemns some parts of itself, and still survives? And all for what purpose? Persecution, controversy, poverty, and death? That is all that any insider might have hoped to receive by adhering to their conspiracy. As Paul himself says in 1 Corinthians 15: "If in Christ we have hope in this life only, we are of all people most to be pitied."
If the "conspiracy" is that Jesus really did rise from the dead, and that this was the central truth that held early Christianity together, despite all of its divisions - then all this makes sense. But if you want all this to be the result of some made-up story, then you have to postulate a completely ridiculous conspiracy - one where the leaders somehow concocted the greatest and most effective lie the world had ever seen, despite being an inept, fractious group of people with little control over their followers. Or, you can instead postulate a truly vast conspiracy, one which planned for all this persecution and division and infighting from the beginning. You can postulate whatever you'd like. That's the whole appeal of conspiracy theories. But at the end, the prior probability for any conspiracy you postulate will be absolutely minuscule.
The "final" odds for the resurrection
All this is difficult to fully quantify in terms of a Bayes factor. But using the arguments about Paul's testimony from the earlier parts of this work, we can say that his independence by itself is worth several orders of magnitude. Then the rest of the anti-crackpot properties can be used to simply firm up that value. Meaning, the overall Bayes factor of the entire anti-crackpot suite can be assigned, at a minimum, several orders of magnitude - let's say about 3 orders.
Recall that the "skeptic's distribution" approach resulted in a conservative estimate of 1e14, and a likely estimate of 1e16, as the Bayes factor for the testimonies in 1 Corinthians 15. Adding 3 more orders of magnitude results in 1e17 to 1e19. When this Bayes factor is set against a prior of 1e-11, it results in a final odds of 1e6 to 1e8, as the conservative and likely values, respectively.
I would characterize this as "safely above 1e6". The odds for Christ's resurrection is safely above a million-to-one, even after allowing the skeptic to consider every possible alternative explanations, up to and including all the crackpot theories.
Furthermore, recall that this is still using only the data summarized in 1 Corinthians 15. We are still ignoring other important witnesses like the women at the tomb, and vastly underestimating the testimony of people like John.
But with those restrictions and conditions, "safely above 1e6" is my final answer for the odds of Christ's resurrection. In other words, the probability that Jesus rose from the dead is safely above 99.9999%.
Conclusion: the resurrection is still certain, even after taking every possibility into account
Let us summarize the "skeptic's distribution" argument for Christ's resurrection.
We've already seen that any kind of reasonable investigation into Jesus's resurrection accounts would conclusively demonstrate that Jesus did rise from the dead. The only possibility left for the skeptic is to turn to unreasonable hypotheses - that is, to crackpot theories like conspiracies.
The distinguishing feature of these theories is that they postulate a near-total interdependence among the evidence, as if they were all manufactured by a single source - the conspiracy. This allows them to ignore the abundance of evidence for a certain position, and instead attribute it all to a rather unlikely prior.
So then, how could we quantitatively consider the interdependence of evidence, fully taking everything into account, up to and including all the different possible crackpot theories?
We constructed the "skeptic's distribution" - the probability distribution for achieving a certain level of evidence for a resurrection, assuming a skeptical, anti-supernatural worldview. This probability distribution is actually quite accessible, since every single non-Christian resurrection report in world history would be the result of a sample drawn from it. And because it's constructed from these historical, empirical samples, the final distribution is quite indisputable - one cannot reject the distribution without rejecting history or empiricism.
Furthermore, such a distribution fully takes into account the aggregate of all the different types of theories that actually could have happened in history, including all the crackpot theories. The results of all things like conspiracy theories or religious mass delusions would show up in the samples, and the samples can then be extrapolated for things beyond what actually happened in history.
Once we had the "skeptic's distribution", the rest of the calculation was easy. We calculated the "skeptic's probability", which is the probability for the "skeptic's distribution" to generate at least a Jesus-level resurrection report. Since the corresponding "Christian's probability" is of order unity, the Bayes factor for Jesus's resurrection is essentially the reciprocal of the "skeptic's probability".
We first constructed the "skeptic's distribution" using the most pro-skeptical assumptions possible. Even then, this gave "even odds" of Jesus's resurrection having taken place, under an impossibly favorable set of assumptions for skepticism.
Re-running the calculation with demonstrably more realistic - but still very conservative - assumptions, we saw that the Bayes factor for Jesus's resurrection was still at least around 1e14 or 1e16. Against a prior of 1e-11, this puts the odds for Jesus's resurrection at somewhere between 1e3 to 1e5.
However, all of this considered only the sheer amount of testimonies for Jesus's resurrection. It did not consider the high degree of independence among these testimonies, which would further favor the case for the resurrection. For instance, just the conversion of Apostle Paul would be enough to put any kind of conspiracy theory beyond any realistic possibility. And Paul's independence only scratches the surface of the many defense against crackpot theories built in to the resurrection testimonies.
After taking these into account as well, the final odds for the resurrection turned out to be safely above 1e6. In other words, the probability that Jesus rose from the dead is safely above 99.9999%, even after taking every alternative explanation - up to and including crackpot theories - into account.
All this was with only part of the evidence for Christ's resurrection (those summarized in 1 Corinthians 15). A more complete look at all the testimonies would drive the numbers higher still.
The conclusion is clear: Jesus almost certainly rose from the dead.
PART V:
More double checks
Chapter 14:
Double check: reports of miracles in other religions
The stance on non-Christian miracles
As before, we want to double check our methodology. We want to apply it to different situations and make sure that it gives the expected results.
A common argument from skeptics is that we cannot accept the miraculous stories about Jesus while simultaneously rejecting them for all non-Christian miracle-workers in world history. But that is nonsense. Of course we can discriminate between these stories. It just comes down to discerning which ones have enough evidence.
So, for instance, we've already shown that the stories about Jesus's resurrection have far more evidence behind them than any other resurrection stories in world history. We've done the math. And that math, with its self-consistent logical rigor, compels us to both accept Jesus's resurrection, and reject the other resurrection accounts. It merely comes down to their respective level of evidence.
But what about other, non-resurrection miraculous stories? Could any such stories of non-Christian origins be true? A Christian must answer "no" for the most part. There may be some allowances for God sending 'rain on the righteous and the unrighteous', but certainly any miracles that expressly support a non-Christian worldview must be false.
And here, both Christians and skeptics can find common ground. We both believe that a large majority of non-Christian miracle stories must be false. And if the Bayesian methodology that I've employed thus far is sound, it ought to be able to come to that conclusion. And by doing so, the methodology will demonstrate that soundness - in accordance to Bayes' rule, for both Christians and skeptics.
So let's begin this extension into non-Christian, non-resurrection miracles, and thereby double check our methodology.
Ichadon
We'll start out with a quick, easy case, where the conclusion is not difficult to reach. Many miraculous stories fall into this category. A full-blown analysis is not necessary in such stories, as none of them reach anywhere near the level of evidence in Jesus's resurrection. Just a quick comparison to with our previous analysis will do.
For example, there's the story of Ichadon, an ancient Korean Buddhist monk. It's said that the miraculous signs accompanying his death resulted in the adoption of Buddhism as the state religion. His story is recorded in the "Lives of Eminent Korean Monks" - about 700 years after the fact. As we've mentioned before, this kind of time gap makes any kind of personal testimony impossible, so the level of evidence here only reaches the "some people say..." level, which falls far short of overcoming the small prior against a genuine miracle.
Vespasian
Our first major case will be the stories of miraculous healing attributed to the Roman Emperor Vespasian. These are recorded in history through the following accounts:
Vespasian himself healed two persons, one having a withered hand, the other being blind, who had come to him because of a vision seen in dreams; he cured the one by stepping on his hand and the other by spitting upon his eyes.
- Cassius Dio, Roman History, 65.8
Vespasian as yet lacked prestige and a certain divinity, so to speak, since he was an unexpected and still new-made emperor; but these also were given him. A man of the people who was blind, and another who was lame, came to him together as he sat on the tribunal, begging for the help for their disorders which Serapis had promised in a dream; for the god declared that Vespasian would restore the eyes, if he would spit upon them, and give strength to the leg, if he would deign to touch it with his heel. Though he had hardly any faith that this could possibly succeed, and therefore shrank even from making the attempt, he was at last prevailed upon by his friends and tried both things in public before a large crowd; and with success. At this same time, by the direction of certain soothsayers, some vases of antique workmanship were dug up in a consecrated spot at Tegea in Arcadia and on them was an image very like Vespasian.
- Suetonius, The Lives of the Twelve Caesars: Divine Vespasian, 7.2
During the months while Vespasian was waiting at Alexandria for the regular season of the summer winds and a settled sea, many marvels continued to mark the favour of heaven and a certain partiality of the gods toward him. One of the common people of Alexandria, well known for his loss of sight, threw himself before Vespasian's knees, praying him with groans to cure his blindness, being so directed by the god Serapis, whom this most superstitious of nations worships before all others; and he besought the emperor to deign to moisten his cheeks and eyes with his spittle. Another, whose hand was useless, prompted by the same god, begged Caesar to step and trample on it. Vespasian at first ridiculed these appeals and treated them with scorn; then, when the men persisted, he began at one moment to fear the discredit of failure, at another to be inspired with hopes of success by the appeals of the suppliants and the flattery of his courtiers: finally, he directed the physicians to give their opinion as to whether such blindness and infirmity could be overcome by human aid. Their reply treated the two cases differently: they said that in the first the power of sight had not been completely eaten away and it would return if the obstacles were removed; in the other, the joints had slipped and become displaced, but they could be restored if a healing pressure were applied to them. Such perhaps was the wish of the gods, and it might be that the emperor had been chosen for this divine service; in any case, if a cure were obtained, the glory would be Caesar's, but in the event of failure, ridicule would fall only on the poor suppliants. So Vespasian, believing that his good fortune was capable of anything and that nothing was any longer incredible, with a smiling countenance, and amid intense excitement on the part of the bystanders, did as he was asked to do. The hand was instantly restored to use, and the day again shone for the blind man. Both facts are told by eye-witnesses even now when falsehood brings no reward.
- Tacitus, Histories, 4.81
So, what are we to make of these accounts?
We apply the methodology that we've been using all this time. How much evidence is there for these miracles? And is it enough to overcome the small prior?
As before, we first look at the people providing the testimony. Who claimed that this actually happened? We have three accounts by three well-known historians, but they're merely reporting what they heard from others in their research. Now, we didn't count every New Testament author as witnesses for merely writing about Christ's resurrection. We only counted those who actually gave personal testimonies. So we can no more count the three historians above as witnesses. Who were their sources? Who were the actual, original individuals that personally witnessed and reported Vespasian's miracles?
None of the accounts give specific names for such individuals. We have some vague characters, such as "people of Alexandria" or "[Vespasian's'] friends" - but there are no named characters, except perhaps emperor Vespasian himself. However, this group of people seem to be well specified: they're better than the "some people" level of evidence that we've seen so much of thus far. The witnesses are the crowd of people who gathered in Alexandria and saw Vespasian heal these two people. Tacitus mentions eye-witnesses, and presumably he could have gotten to these specific individuals if he had to. So, overall, I would say that this testimony is on par with the 500 disciples witnessing Christ's resurrection. The Bayes factor for such a testimony is in excess of 1e8, according to our previous calculations. It would be greater still if you counted Vespasian himself.
So, that's a pretty big Bayes factor, right? So this event actually happened?
But now we run into the problem of precisely defining what "this event" was. Did Vespasian "heal" two people in front of a large crowd? The prior odds for such an event is decently large - certainly much larger than someone coming back from the dead. Let's say, for the sake of argument, that it's around 1e-6. This is easily overpowered by the Bayes factor of the testimonies above, which exceeds 1e8. We can be decently confident that such an event happened.
But was the healing supernatural? Now we're talking about an entirely different kind of event, with different prior odds. A supernatural healing of this type would be almost as unlikely as a resurrection. Since a resurrection had prior odds of 1e-11, let's be generous and assign this a prior odds of 1e-8. But... isn't the Bayes factor still large enough to overcome that?
No, not at all. For the Bayes factor itself now changes. For one, we can no longer count on Vespasian's testimony at all. Apart from the massive conflict of interest (to be addressed shortly), Vespasian himself doesn't believe that he can actually heal these people in the beginning. Tacitus explicitly reports that everything was perfectly achievable through mundane means, and that Vespasian only attempted the healings when he was informed of this possibility.
Therefore, Vespasian himself is certainly not testifying to anything like a supernatural healing here. Neither, for that matter, is the crowd itself, if things went according to what's in Tacitus's account. In fact Tacitus goes to some lengths to provide naturalistic explanations for the "healing" - to such a degree that his account should actually be counted as evidence against a supernatural healing. He calls these people "most superstitious", and nearly explicitly says that anyone in the crowd who actually believed in a supernatural healing would have been deceived. And there is nothing in either of the other two accounts to contradict his.
Tacitus's account is the earliest, most detailed, and most explicit in mentioning witnesses. The other two are mostly just summaries of his. And yet, in this best account, the idea of a supernatural healing is almost explicitly refuted. So what happens to the evidence? Where is the testimony?
There is essentially none left. At best it's reduced to that familiar, unspecific "some people say..." level. This is nowhere enough to overcome a prior odds of 1e-8, and therefore we can be very confident that a supernatural healing did not take place here.
In summary: on the question of whether there was a public spectacle where Vespasian "healed" two people, a prior odds of something like 1e-6 is overcome by a Bayes factor exceeding 1e8 - therefore we can reasonably hold that this actually took place. But on the question of whether this "healing" was supernatural, a prior odds of 1e-8 is essentially unmoved against a "some people say..." level of evidence. We are therefore very certain that the "healing" was not supernatural.
And all this is without taking into account the enormous potential for deception, conspiracy, political shenanigans, or a publicity stunt. Vespasian was a newly crowned Roman Emperor, after all. Taking that into account would lower the final probabilities even further, for both the supernatural and the mundane versions of the event. In the end, I think our methodology brings us to the point where there's better than even odds for some kind of public spectacle taking place, but the nature of the event was almost certainly not a supernatural healing.
Do you agree with that assessment? Does it seem reasonable to you? Good - then you are compelled to correspondingly increase your faith in the methodology we used, and therefore increase your degree of belief in Christ's resurrection.
"Something happened" vs. "a miracle happened":
But wait! Can a similar type of reasoning be used against Christ's resurrection? Could it be argued that "something" probably happened with a man we now call Jesus, but that it was not anything supernatural?
No, it cannot. The reasons that existed for Vespasian that allowed for such an argument simply does not exist for Christ's resurrection.
For one, a resurrection is nearly impossible to fake. One can fake being healed of blindness, or of a defective limb, without much effort. You can do it right now - you just need a little bit of acting skills. Just a bit of placebo effect or the excited anticipation of the crowd can be enough to get someone to walk around for a few steps, or convince a man with poor vision that he sees better. That is all that is required to generate the above accounts of Vespasian's miracles. But can you imagine making such an argument for a resurrection? "Are you sure that it wasn't just the placebo effect that cured his death? Or sometimes, if everyone in the crowd anticipates it, a corpse can be encouraged enough to get up and walk."
We believe that nothing supernatural took place in Vespasian's case, partly because what he achieved in healing is not all that remarkable. There are many possible naturalistic explanations. But in Christ's case, you need a naturalistic explanation for a man who was confirmed dead multiple times, who then came back walking, talking, eating, teaching, converting skeptics, and giving missions. Good luck getting all that with common naturalistic explanations like "placebo effect" or "crowd anticipation".
But secondly, and far more importantly, the evidence itself points towards a naturalistic explanation for Vespasian, and a supernatural event for Jesus. We are, as ever, evaluating and following the evidence. The evidence itself - in the form of Tacitus's account - spells out that Vespasian's miracles likely had naturalistic causes. In Jesus's case, it's again the evidence itself - in the form of the text of the New Testament - that consistently and repeatedly tells us that Jesus's resurrection was a supernatural event.
In order to draw an equivalence, and say that "something happened, but nothing supernatural" in both cases, you would need the same kind of evidence. You would need something like the Gospel of John explicitly stating that Jesus's disciples stole the body, and spelling out exactly how they did it and why there was nothing supernatural required. If the Gospel of John actually said such things, then you could draw an equivalence between the "miracles" of Vespasian and the resurrection of Jesus.
But, of course, the Gospel of John does not in fact say that. It is no good making up evidence you don't have. We are to only follow the evidence we actually have. So, merely mentioning that "something happened, but probably nothing supernatural" is worthless. It's wishful thinking about evidence you don't have. Speculations - merely mentioning possibilities - do absolutely nothing against a Bayesian argument. It is evidence, not speculations, that move probabilities.
So in considering all of the above, you see that the methodology is perfectly consistent in concluding that nothing supernatural happened in Vespasian's stories, while also concluding that Jesus rose from the dead.
Splitting the Moon
In Islam, the Quran itself is said to be Muhammad's chief miracle - but it is difficult to evaluate this claim. We would need to dig into specific passages in the Quran and interpret it, which would easily end up leading down a rabbit hole. Otherwise, it's hard to say that the mere existence of the text is miraculous. Rather, we want a clear miracle - a miracle that can be recognized as such by anyone, like a resurrection. That is the point of a sign, after all. What good is a sign if it can't be clearly recognized?
The best known Islamic miracle of that kind - a miracle which is clearly a miracle - is Muhammad's splitting of the moon. But even this miracle is highly controversial. There are even certain interpretations - Islamic ones - which deny that this took place at all. They say that it is rather a prophecy that's suppose to take place at a future time.
The clearest case for the splitting of the moon being a literal miracle come from certain hadiths. Now, the sheer number of such citations and the authority they claim in going back to Muhammad's companions is indeed impressive - if one were to stop their investigation there.
But if you actually read these hadiths, you immediately notice how sparse they are. For example, one of the more detailed hadiths on the subject reads:
We were along with God's Messenger at Mina, that moon was split up into two. One of its parts was behind the mountain and the other one was on this side of the mountain. God's Messenger said to us: Bear witness to this.
And... that's it. Clearly, such a claim doesn't score particularly high on the "sincere and insistent" scale. Many of these hadiths are so short and free of context that it's hard to get a definitive narrative or interpretation out of them. For example, the above hadith is compatible with Muhammad (God's Messenger) causing the splitting of the moon, or with him merely pointing out some kind of a natural spectacle. In addition, many of these hadiths are highly interdependent, as their texts are often just a copy or a subset of one another. In fact, if you were to weave together all the independent text in all of the hadiths about this miracle, it would hardly amount to a decently sized paragraph.
Compare that to, say, the Holy Week narrative in the Gospel of John, which spans several chapters in length and leaves no ambiguity in relating Jesus's death and resurrection. The difference in narrative clarity and the level of detail is incomparable.
It is furthermore worth noting that these hadiths were generally written down over two hundred years after Muhammad's death. They claim a chain of transmission going back to some contemporaries of Muhammad, which is certainly something - this is what keeps the hadiths from degenerating to the "some people say..." level of evidence - but this chain often exceeds five or six people in length. These hadiths are therefore distinctly inferior to any of the testimonies in the New Testament in terms of their authenticity, just on this point alone.
Considering all this, it's hard to see how these sparse testimonies could add up to overcome the small prior odds for a miracle as remarkable as the moon splitting in two. Let's try a rough estimate: A full-blown, sincere personal claim of the type we've been studying has a Bayes factor of 1e8. But that's not what we have here. Instead, we have a set of short claims made with no context, relayed to us after more than 200 years, through a chain of transmission involving five or six people.
For such a testimony, I would assign a Bayes factor of ~ 1e1-1e4 for each of the 5 or so companions that were suppose to have originated some of the hadiths, giving an overall Bayes factor of around 1e13 - which would then be quickly reduced to below 1e10 when dependence factors are taken into account. At this point it's worth noting that the dependence factors are especially severe in this problem, with its highly interdependent texts.
Another way to think about this is to consider these hadiths to be parallel to the testimony of the group of apostles mentioned in 1 Corinthians 15, if we only had very sparse records of the apostles from over two hundred years after the fact. This again brings us to numbers far less than 1e10.
On the other hand, a miracle like splitting the moon is indeed highly remarkable - so it should at least be given a prior odds on par with the resurrection, of 1e-11. So the math doesn't work out. The evidence just doesn't add up. A Bayes factor of less than 1e10 doesn't overcome a prior odds of 1e-11. In addition, we must consider that there are no credible non-Islamic records of this highly visible and remarkable astronomical event, and the fact that there are good Islamic reasons to disbelieve that this ever happened. At the end of it all, we can be fairly confident that this did not actually happen as a miracle.
So, overall, we can say that our methodology does correctly reject non-Christian miracles. This validates the methodology for our test cases, and therefore compels us to accept the results when the same methodology says that Jesus definitively rose from the dead.
Accounts in Josephus
Let us now consider some miraculous stories from the works of Josephus.
Josephus was a Jewish historian who was active in the latter half of the first century. His works include The Jewish War and Antiquities of the Jews. They deal with the contemporary and the ancient history of the Jews, from the perspective of the a Jew living after the Siege of Jerusalem and the destruction of the Second Temple in 70 AD.
As such, he is a valuable resource in understanding the background of early Christianity, and his works are quite compatible with the New Testament. The miracles in his works that we're about study are likewise Jewish in origin, and compatible with Christianity. It would do Christianity no harm if these miracles really took place. They do not meet our earlier criteria of "miracles that expressly support a non-Christian worldview". In fact, Christianity could quite happily accommodate Josephus's stories about the signs surrounding the sacking of Jerusalem (Jewish War, 6.5.3).
After all, Christianity started out as a Jewish sect, and acknowledges the fundamental truth in Judaism. Jesus himself was Jewish, and famously predicted the sacking of Jerusalem. The New Testament acknowledges the existence of miracle workers and exorcists outside the immediate circle of New Testament Christians, of varying degrees of legitimacy, including one that Jesus himself was okay with.
However, even all that doesn't mean that we ought to uncritically accept the miraculous stories in Josephus. We will evaluate some of them, according to the methodology we've developed, and thereby also evaluate the methodology itself.
Honi the Circle-drawer
First, Let us consider the story of Honi the Circle-drawer, also known as Onias. Josephus tells his story in the Antiquities of the Jews, 14.2.1-2, briefly mentioning that he once called on God to bring down rain.
Now there was one, whose name was Onias, a righteous man he was, and beloved of God, who, in a certain drought, had prayed to God to put an end to the intense heat, and whose prayers God had heard, and had sent them rain.
The rest of the story is about how a warring faction attempted to get him to use his miraculous powers against their enemies - so apparently his feat was publically known. He is also mentioned in some other Jewish works. But at the end of the day, there just isn't much written about him.
Now, what are we to make of this story? Unfortunately Josephus doesn't mention any specific sources in relating this story. He simply tells it as a story, more than a hundred years after the fact. That means we don't have anything like any of the direct witnesses we have for Christ's resurrection. The best we can do here is infer that Josephus and others must have heard the story from someone, presumably from a group of people. But given that we don't know anything else, again the most we can do is credit this account with the "some people say..." level of evidence. This is nowhere near enough evidence to overcome the small prior against a supernatural event, and we can be fairly certain that this event did not actually occur as a miracle.
Eleazar the exorcist
Next, let us consider the story of Eleazar the exorcist, as told in the Antiquities of the Jews, 8.2.5:
I have seen a certain man of my own country, whose name was Eleazar, releasing people that were demoniacal in the presence of Vespasian, and his sons, and his captains, and the whole multitude of his soldiers. The manner of the cure was this: He put a ring that had a Foot of one of those sorts mentioned by Solomon to the nostrils of the demoniac, after which he drew out the demon through his nostrils; and when the man fell down immediately, he abjured him to return into him no more, making still mention of Solomon, and reciting the incantations which he composed. And when Eleazar would persuade and demonstrate to the spectators that he had such a power, he set a little way off a cup or basin full of water, and commanded the demon, as he went out of the man, to overturn it, and thereby to let the spectators know that he had left the man; and when this was done, the skill and wisdom of Solomon was shown very manifestly: for which reason it is, that all men may know the vastness of Solomon's abilities, and how he was beloved of God, and that the extraordinary virtues of every kind with which this king was endowed may not be unknown to any people under the sun for this reason, I say, it is that we have proceeded to speak so largely of these matters.
Well, now this sounds pretty impressive! Josephus names himself as an eyewitness! And also his Roman patron, the emperor Vespasian, whom Josephus would not invoke lightly! And a great number of Vespasian's associates! Let's see what we can make of this.
First, Josephus and Vespasian are both very well known historical characters, easily on par with the named individual witnesses in 1 Corinthian 15. Josephus furthermore mentions a crowd of other people - Vespasian's sons, captains, and soldiers. This crowd is specific enough to match the crowd of 500 in 1 Corinthians 15. The only thing missing from the roster in 1 Corinthians 15 is the group of apostles, and a third major historical character. So that's nearly as much evidence as there is for Christ's resurrection, right?
But we have not yet considered the full qualifications to match the 1 Corinthian 15 witnesses. First, Josephus's testimony here is nowhere as earnest or insistent as the testimonies of someone like apostle Paul. The above passage is the only thing that's known about this Eleazar. Josephus mentions him this one time, and that's it. Compare that to the twelve disciples, who completely oriented their entire lives around the resurrection of Jesus Christ. Compare also to the apostle Paul, whose every writing can be traced back to his testimony that Jesus rose from the dead. There can really be no comparison.
Furthermore, it's worth noting that Josephus mentions this story in a larger narrative about king Solomon. Yes, that Solomon - the son of king David, builder of the first temple, well known for his wisdom. What's a story involving Vespasian doing in a narrative about king Solomon, who lived a thousand years earlier? Well, it turns out that this whole story is actually an aside, an anecdote that Josephus tells to demonstrate how wise Solomon was. He was saying that Solomon was so wise that his wisdom was used to exorcise demons even after all this time. In other words, the whole story is a parenthetical remark to the main point he was trying to make. In fact, for Josephus's main point, it doesn't even matter if this exorcist was genuinely supernatural. A fake exorcist invoking Solomon is still evidence for Solomon's renown. This erodes the testimony further on the "earnest" and "insistent" scales, for everyone involved.
In addition, there's an overwhelming amount of dependency factors at work here. Josephus's main motive, as plainly written out in the text itself, is to impress his Roman audience with the wisdom of Solomon. And this motive drives - and therefore serves as the source of dependency for - his choice to name the other witnesses in his account, and describe the exorcism as a success. Given that he was writing to a Roman audience about Solomon, one of the most famous Jewish kings, it makes sense that he would invoke Vespasian and his associates.
But it's important to note that we're not told what Vespasian and his associates thought of the whole affair. Indeed it's not even clear that they can be said to have given any testimony. Josephus mentions that they were in the audience, but he does not record their personal reactions. Clearly they cannot count as being more impressed than Josephus, as there are no records of any testimony that they, or anyone else, gave concerning this event.
And this complete lack of any other mention of this story makes the dependency factors far worse. The testimonies in 1 Corinthians 15 are of course all attested to in multiple other places in the New Testament, and corroborated by multiple non-biblical sources. We have no doubt that their testimonies are accurately summarized in 1 Corinthians 15. Even Vespasian's healing miracles had multiple attestations. But with this story, it's Josephus and only Josephus, who is himself one of the named witnesses. Everything depends on his testimony, on that short passage he wrote - including his claim that there were other testimonies. This dramatically increases the chance of near-complete dependency among the witnesses.
Lastly, it's again worth noting that a resurrection is nearly impossible to fake, whereas in Josephus's story the text itself suggests that nothing remarkable happened. He specifies very simple, physical things involved in the exorcism: a ring was put up to the demoniac's nostril. The man fell down. There were incantations mentioning Solomon. A container of water was knocked over. All these are simple, ordinary, non-supernatural events. There is no mention of whether the man was actually restored, in the sense of being in his right mind, free of demonic influence. Taken altogether, it again looks like nothing much happened, and this is far less remarkable than the resurrection of a man who was confirmed to be dead multiple times, who then came back walking and talking.
So we see that while the roster of witnesses is pretty impressive for Eleazar's exorcism, their testimony is actually very weak in comparison to their parallels in 1 Corinthian 15, according to the previously established rules for matching testimonies.
"Something happened" vs. "a miracle happened", again
So, let's consider two separate versions of what happened, as we did for Vespasian's healing miracles. First, did something remarkable happen with Eleazar performing in front of a crowd? And second, was it an actual, supernatural exorcism of an actual demon?
As with Vespasian's healing, let's give the first, non-supernatural "something happened" version of the event a prior odds of 1e-6. On the evidence side, after taking everything above into account, I'd give Josephus's testimony for himself a Bayes factor of 1e5 - about half as strong as a full-blown, earnest, insistent testimony. Vespasian's testimony must be significantly less than this, because of the very strong dependency factors involved. It is entirely dependent on Josephus's testimony, and Josephus has a strong motivation to mention Vespasian in appealing to a Roman audience in telling a Jewish story. I'd give Vespasian a Bayes factor of around 1e2, and his associates 1e1. That all comes to a combined Bayes factor of 1e8.
Or, another way of thinking about this is to say that we really only have Josephus's testimony, but the fact that he's willing to involve Vespasian shows that he's really serious, and that upgrades his testimony to make it earnest, giving it a Bayes factor of 1e8. In the end, this very rough calculation comes out to about a final odds of about 1e2. We can be fairly certain that "something happened" here.
As for the "actually supernatural exorcism" case, we'll again start with a prior of 1e-8, as in Vespasian's healing miracles. As for the Bayes factors, Josephus himself gets 1e4. He clearly tells the story as an exorcism which he himself witnessed, but his focus on the physical aspects of the story sounds like he has some doubts himself as to whether there was actually anything spiritual going on - so he loses an order of magnitude compared to the "something happened" case above. Vespasian and his associates get 1e1 together. The drop here for them is due to the lack of any testimony concerning what they thought about the event. Overall, the combined Bayes factor is 1e5.
Again, we can think of this as being entirely up to the testimony of Josephus - he starts with a Bayes factor of 1e8 as in the "something happened" case, but loses 3 orders of magnitude because of the relative ease of faking this kind of exorcism, and his lack of any mention of how Vespasian and his associates reacted. So then, this Bayes factor of 1e5 is set against a generous prior of 1e-8, resulting in a final odds of 1e-3. Therefore, we can disbelieve that anything actually supernatural happened in the story, with some confidence.
The calculations here are quite rough, but I'm fairly certain that the results here are good to a couple orders of magnitude. Something probably happened, but nothing supernatural. Again, does that sound reasonable? Is that what you would have concluded upon reading this passage from Josephus? Good - then the methodology we used is further validated, and the resurrection of Christ is therefore made more certain.
Chapter 15:
Double checks of the "skeptic's distribution" approach
More double checks
(new material)
(youtube views, wealth distributions, crashes in the stock market, ufo sightings, living in a simulation, a case that works out?)
(for miracles: even with just 10x the level of evidence, with no increase in outliers, it's enough)
Double checks: conclusion
So, all that covers the numerous ways to test our methodology. It has passed them all. In everything there is perfect logical consistency and harmony. We believe all the things that ought to be believed, and reject all the things that ought to be rejected. And this methodology, which passes all the tests of the skeptics and the other religions, clearly concludes that Jesus Christ almost certainly rose from the dead.
PART VI:
Challenge and Conclusion
Chapter 16:
The final challenge: replicate the results
The rationale for this challenge
Is anyone still skeptical of the fact that Jesus rose from the dead? Well then, here is one more test, straight from a hallmark of the scientific method:
If you think that the evidence for Christ's resurrection was naturalistically produced, then replicate the result.
We have seen that history, in its natural course thus far, has utterly failed to reproduce a Jesus-level of evidence for a resurrection. It has not even come remotely close. And this has not been for a lack of trying, either - we've cited multiple cases where people tell a resurrection story, but their level of evidence always fell incomparably short of Christ's resurrection.
But perhaps you might succeed! And really, there isn't any fundamental reason why you can't, if Christianity started naturalistically. In fact, if you are not convinced by the arguments in this work, a scientific mindset demands that you give it a try.
So, do you think that there was a massive conspiracy among the disciples to steal Jesus's body and start a new religion? Well, try to start a similar conspiracy of your own! See how well it holds up over the years when people rightly accuse you of being liars, and rightly threaten your reputation and wealth - perhaps even your life and limb!
You think that the Christian resurrection stories started through a mass hallucination, caused by eating a psychoactive plant native to Jerusalem? Well, go find that plant, feed it to a bunch of people, and see if they have the exact same hallucinations about the resurrection of one person!
You think that some gullible religious people couldn't learn to cope with the death of their charismatic leader, and therefore made up the resurrection story? Well, start such a religion yourself, pretend to die, and see what happens!
Don't complain about the scope of the problem, or the amount of people, time, or money you need. Christianity started with Jesus and a handful of his disciples. You and your circle of friends could easily out-scope this group. This is not an experiment that's too big to be attempted. In fact, real-world, large-scale studies on health or sociology regularly out-scope the humble beginning of Christianity.
Don't complain about the right combination of social circumstances needed for Christianity to take off. If you can read this, you probably have access to world-wide travel and possible exposure to an enormous variety of the world's cultures - an advantage that Jesus's disciples did not have. You think that the right set of circumstances only exist in one particular tribal group in Papua New Guinea, or in a specific small town near the outskirt suburbs of Kyoto? You can actually travel to these places, and access the right social and cultural circumstances.
I am being serious here. This is not some cheap taunts against skeptics. If you've read my other writings - if you've even just read everything I've written above - you know that I welcome the testing of my ideas, and that I'm ready to change my beliefs as a result. If you really do come up with a plausible, naturalistic, reproducible way for Jesus's resurrection reports to have been generated, I will change my mind.
But remember that this works the other way too. We've already seen that the failures of the non-Christian resurrection stories have only made Jesus's resurrection more certain. In the same way, failure in an attempt to replicate Jesus's resurrection reports must, of logical necessity, change your mind. You must become more certain of Christ's resurrection.
Of course, abject failure is in fact the most likely outcome of such an experiment. The experiment will produce something - but that something is not likely to be any better than the many other examples in world history, where a "resurrection" was said to have occurred. It will fall pathetically short of the level of evidence established by Christ's resurrection.
And that is why I, personally, won't conduct this experiment: I think the result will be negative - that it will not really add anything new to the data we already have. Furthermore, I have already done my due diligence, and am already well convinced that Jesus rose from the dead. This only cements my expectation of a negative result. I therefore have little reason to conduct this experiment, no more than I have a reason to reproduce the Michelson-Morley experiment to search for the luminiferous aether - I would rather believe in special relativity.
But the situation is exactly the opposite for a skeptic: they should expect a positive result, that there actually is a way to naturalistically reproduce Jesus's resurrection reports. This would, furthermore, be a new result with high impact, which overturns all the historical accounts thus far. They therefore have every reason to conduct this experiment - just as Michelson and Morley did for their famous experiment.
So, that is the challenge: if you are a skeptic, you have every reason - including scientific obligation - to try to replicate Jesus's resurrection reports, to achieve the same level of evidence. Refusing the challenge will have its own consequences, concerning your rationality or your actual beliefs.
The conditions for the challenge
So then, what would count as replicating the evidence for Christ's resurrection?
It's simple. The replication would be a new religious movement based on a "resurrection", which must match or exceed all of the essential components of the original evidence for Christ's resurrection. These components are merely what we've been discussing throughout this work. They consist of the following:
First, the replicated "resurrection" must have sufficient evidence, given as a set of personal testimonies. This must be enough to match or exceed the evidence for Jesus's resurrection. This consists of the following six testimonies summarized in 1 Corinthians 15, with their "matching" conditions.
To match Peter, James, or Paul's testimonies, we will require a sincere, insistent, and enduring personal testimony by a single named individual. They must have been a public figure whose entire life (choice of profession, place of residence, etc.) was lived in complete alignment with that testimony. History must be able to locate this person with great precision, and have a good amount of information available about them.
To match the testimonies of "the twelve", we will require a sincere, insistent, and enduring personal testimony by a group of about a dozen named individuals. They must have been public figures whose entire lives were lived in complete alignment with that testimony. History must be able to locate these people with good precision, and have a good amount of information on at least some of them.
To match the testimonies of "the other apostles", we will require a sincere and enduring personal testimony by a group of individuals. At least some of them must be named. At least some of them must have been public figures on the matter of their testimony. History must be able to locate these people with good precision, but not a lot of historical data is required of them.
To match the testimonies of "the 500", we will require a sincere personal testimony by a large group of people. They need not be named, or be public figures, or endure in their testimony, or have any additional information known about them. But history must be able to locate these people precisely enough, so that at least some of them could be theoretically pointed out by a well-known figure like Apostle Paul.
This is merely a repeat of the same conditions that we've previously used. This covers the raw amount of evidence you need.
Second, this evidence for the replicated "resurrection" must have the certain qualities which make conspiracies and other crackpot theories unlikely. Again, this is only what we've covered before - but here it takes on added importance, since we're specifically talking about artificially replicating the evidence.
One of the prominent, public witnesses must be someone who was publicly known for being strongly opposed to this new "resurrection" movement from the beginning. This person must have done real, material, public harm to that movement, prior to his or her change of heart. That change of heart must come from a conviction that this "resurrection" really happened.
There must not be an obvious prior connection or common cause among the prominent, public witnesses. They must be reasonably independent.
Within, say, 50 years of its beginning, this "resurrection" movement must cover a wide geographical area with great cultural and linguistic diversity - an appropriate region might be "the Middle East", "the Mediterranean", or "Southeast Asia". Its numerous followers, too, must reflect this diversity. As a corollary, the movement cannot be entirely directed by a central authority, and different parts of it must be in severe contention with one another.
The major witnesses must be "sincere, insistent, and enduring" for those 50 years. They must staunchly testify to the replicated "resurrection" for at least that long.
Material wealth or political power cannot be a tangible, or even likely, reward for joining the movement.
There must be no evidence against this "resurrection" which endures past the 50 year mark.
Third, there are some further implicit factors which now need to be spelled out.
This movement cannot be built on Christianity. Otherwise, the strong dependency factors would ruin the experiment. It must achieve everything from scratch, without a preexisting foundation guiding how things ought be or ought to turn out - just as Christianity itself did. It's okay for the movement to get started in a "Christian nation", it just can't be directly based on or inspired by Christianity.
The entire replication must be plausible. If you successfully start such resurrection-based movement, but it requires circumstances which occur once in a trillion years, that would not be considered plausible. For example, let's say you find a way to reproducibly convince people of a "resurrection". But it only works on quintuplets who were struck by a ball lightning at the moment of their conception, and it can furthermore only take place when twelve comets brighter than Venus simultaneously show up in the night sky. Such an explanation for the original, Christian resurrection is not plausible, even if it may be naturalistic. Whatever mechanism you use to generate your replication must be likely enough to have had a decent chance of actually occurring in history.
Lastly, you may not brute force the problem with an overwhelming amount of resources. Recall that Christianity started with Jesus and a handful of his followers, with no great wealth, political power, or specialized scientific knowledge. Your effort must start with similarly humble circumstances. You cannot, for instance, enlist a billionaire to pay off the population of a whole city in some poverty-stricken country, to get them to act out a "resurrection" for your first set of witnesses. You cannot become the dictator of a country and force people to comply with your lies. You cannot impress some primitive, hidden tribe with modern science to get them to believe. You must play fair - using the same means that were available to the early Christians, if indeed their movement actually started naturalistically.
So, that is the challenge. You think you can fake a movement appearing to meet all of the above conditions? Go ahead and try. If you succeed, I will change my mind about the resurrection.
Chapter 17:
Conclusion and epilogue
Conclusion
At long last, we can summarize this entire series.
First, we calculated the prior odds for the resurrection of Jesus Christ. This prior cannot be zero. That would violate one of the fundamental tenets of Bayesian thinking. Nor is it empirically justified, since we haven't observed an infinite number of people who didn't come back from the dead. Instead, empiricism requires this prior to be about the same as the reciprocal of the total number of non-resurrecting people we have observed, if we have observed zero resurrections. Rather generously, this could be placed at 1e-11 - roughly corresponding to observing the non-resurrection of the total number of humans that have ever lived.
Second, we calculated the Bayes factor for an earnest, insistent human testimony. Human testimony has value. This is not just an opinion or a hypothesis: human testimony must have value because your odds for an event actually changes when someone makes a testimony. Therefore, it must have a Bayes factor. Thus the value of a human testimony can be calculated on a mathematical and empirical footing. As it turns out, for an individual testimony like the ones appearing in Jesus's resurrection accounts, the Bayes factor is about 1e8. This is validated by multiple empirical observations, natural experiments, and thought experiments. There are several ways to modify this value depending on the exact nature of the testimony, which include things like dependency factors, incentives to lie, and the "stretchiness" of human testimony. All of these can be understood, and were taken into account in the Bayes factor.
We next evaluated the amount of evidence for Jesus's resurrection. Just from the testimonies summarized in 1 Corinthians 15, we saw that six individuals or groups - Peter, James, Paul, the twelve disciples, the other apostles, and a crowd numbering more than 500 - all testified to Jesus's resurrection. Applying the Bayes factor calculated above - with the appropriate modifications - to this set of evidence gave an enormous total Bayes factor, easily enough to completely overwhelm the prior odds of 1e-11 against the resurrection. We therefore concluded that Jesus almost certainly rose from the dead.
This calculation was then double checked against other historical reports of a resurrection. By comparing against the non-Christian resurrection reports, we saw that the level of evidence behind Jesus's resurrection is a clear outlier, again to an absolutely overwhelm degree. This comparison therefore validated our earlier conclusion that Jesus rose from the dead.
Furthermore, because of the nature of this calculation, its conclusion is immune from many of the common skeptical arguments against the resurrection. The various possibilities - all the likely ways that the testimonies could have been wrong - have been already taken into account. No amount of speculation about how the resurrection reports could have been generated by naturalistic chance has any effect on the conclusion. We don't need to play 'what-if whack-a-mole' against the skeptic's speculations. This is a Bayesian argument. Speculations do absolutely nothing against it. Only evidence moves the odds.
However, because the Bayes factor for Jesus's resurrection is so large, we then had to start worrying about crackpot theories - conspiracies, vivid mass hallucinations, alien interference, and the like. At the level of certainty which was implied by our calculation, we had to take even such things into account. This required a recalculation, specifically to take into account every possibility, up to and including the near-total interdependency of evidence implied by such theories.
Fortunately, we have the historical data about other, non-Christian resurrection reports. This allowed us to explicitly construct the "skeptic's distribution", which is the probability distribution that generates the other historical resurrection reports through naturalistic means. This construction explicitly took into account the possibility of crackpot theories. This is the distribution that the skeptic must use, if they are to hold on to empiricism and naturalism - for this distribution incorporates the empirical, historical results of all such crackpot theories at the rate which actually occurred throughout history, and furthermore continues the distribution beyond the empirical end of the distribution using an exceedingly generous set of assumptions for the skeptic.
But even after taking even the crackpot theories into account, with a set of highly favorable assumptions for the skeptic, we saw that the Bayes factor for the testimonies for Jesus's resurrection still enough to amply overpower the 1e-11 prior odds. Combining this with the anti-crackpot defenses built into Christianity, we got a "final" probability for Jesus's resurrection of greater than 99.9999%. So this recalculation again affirmed our previous conclusion: Jesus almost certainly rose from the dead, even when we consider all possible alternative theories.
But we did not stop there. As yet another test of our methodology, we used it to tackle a number of other, non-Christian, non-resurrection miracle stories. In each case we reached the reasonable conclusion - that probably nothing supernatural took place in these cases. It was a simple matter of quantifying the fact that the Christian miracles had ample evidence behind it, whereas the other miracles did not. Because this double-check reaches the same conclusions as the skeptic, the skeptic must therefore count this as an additional validation of the methodology.
But if anyone is still not convinced of Jesus's resurrection, I have the following challenge for them: naturalistically replicate the resurrection reports. Using the same means that were available to Jesus and his disciples - no political power, no great wealth, and no modern science - generate multiple, detailed, independent, earnest, insistent, enduring, and life-changing personal testimonies, from a great number of diverse types of people, unanimously testifying to a singular resurrection event. And this must be achieved in spite of deadly persecution, in a fractious movement with no central control, along with a host of other difficulties and conditions. If you still doubt Jesus's resurrection, the scientific method demands that you take up this challenge.
Alternatively, you can follow the logic of the methodology outlined above. It is based on mathematics and empirical data, has been validated and double-checked multiple times, and gives the correct answer in all cases where the answer could be agreed upon. Short of embracing epistemological obliteration, you must accept its conclusion: Jesus almost certainly rose from the dead.
Epilogue
This work is now at the end of its "second draft" form.
It all began as a post on March 21, 2016 - The week of Easter. When I started writing it, I only had the idea that you can actually calculate a value - a Bayes factor - for a human testimony. In a demonstration of my poor long-term planning skills, I initially just wanted to write that one post for Easter, about the likelihood of the resurrection based on the Bayes factor of the disciple's testimonies. But the ideas kept coming, they all required thorough explanations, and the posts just kept on writing themselves.
I finished my series of posts on April 10th, 2017 - the week of the next Easter. That was a milestone for wrapping up the first phase of the work, which only existed as a sequence of scattered posts at the time. By this time, I was surprised to find that I had written a book-length work, totaling tens of thousands of words. I suppose I could call this the "first draft".
And now this work is facing its third Easter, which will be on April 1st, 2018. Over that last year I've collected the posts into one place and edited them a great deal, to get them to this "second draft" state. I think this work is now ready for a small, not quite "public" release. Over the next year, I plan to gather feedback and make any other changes that come up.
There are a number of things I want to say about the experience of writing all this.
First, I want to point out that in this whole time, I've never thrown out bad results or concealed disadvantageous conclusions. Every time a new idea came to me - every time there was a new way to test the veracity of the resurrection - I explored it, quantified the essential thoughts, thoroughly performed the necessary calculations, and presented the results. There were some thought branches that did not make it into the final work, but these were all because of reasons like the initial idea was mathematically unworkable, or the thought ended up being mistaken or redundant. Again, there was never a single instance where I reached a conclusion against the resurrection, and decided to hide or ignore it. There is no selection or confirmation bias here. The resurrection was validated each and every time.
Second, I'd like to thank and acknowledge Aaron Wall of Undivided Looking for meaningfully affecting my thoughts and the shape of this work. He was of great help on a number of different subjects covered in this work, such as the handling of dependency factors and extreme odds values, and the "stretchiness" of human testimony.
Lastly, I'm again deeply thankful and gratified to have reached this next milestone with another year's effort, and I would appreciate your continued support and readership as I improve this work. Happy Easter to you all - Christ is risen indeed!
You may next want to read:
3 comments on “Bayesian evaluation for the likelihood of Christ's resurrection (frozen copy)”
Leave a Reply
You must be logged in to post a comment.
Post Importance
Post Category
• humanities (22)
• current events (26)
• fiction (10)
• history (33)
• pop culture (13)
• frozen (8)
• math (57)
• personal update (19)
• logic (65)
• science (56)
• computing (16)
• theology (100)
• bible (38)
• christology (10)
• gospel (7)
• morality (16)
• uncategorized (2)
I have to admit that I haven't (yet?) made it through the whole thing. But right off the bat, the 1e-11 number feels arbitrary. To get a better number, I think you have to define what's meant by "death" and "back from death".
When Jesus died, did he decompose? The odds against insects eating his eyeballs are manageable, but what about mold spores growing in his lungs? His blood congealing? I don't know exactly what happens to a brain when it's left at room temperature for a few days, but I'm guessing it's not pretty.
If the body did decompose, what does it mean for him to come back to life? Was the damage undone at a cellular/molecular level? Or was he walking and talking despite a liquified nervous system?
These processes all take place at a very small scale. The numbers at play will be much larger than human population scales, and the entropic barriers correspondingly higher.
Sorry, I just got through a big backlog of comments.
Your comment here is actually valuable, because it illustrates the difference between a likelihood, and prior odds. The probability you want to use - on that's much smaller than 1e-11 - is actually the likelihood, given your hypothesis (that a resurrection requires a entropic reversal at a microscopic level). That likelihood is indeed tiny, as you point out.
Of course, under a different hypothesis - say, if Jesus was supernaturally resurrected - then the likelihood would be very much larger.
So, how could you judge between these hypothesis? You could be the kind of person that says "well my hypothesis is obviously right, so therefore we should use its probablility!"
Or, you can do what I did: go with the empirical facts, and use an empirical prior. The great thing about this is that it doesn't require either of us to debate about the various hypotheses for the prior. We just let the empirical data set the prior odds.
Also, the latest version of this is posted at http://www.naclhv.com/2017/04/bayesian-evaluation-for-likelihood-of_17.html, if you want to read the latest version. It's being constantly updated.