NaClhv

This post is a consolidation of a whole series of posts into one post. It's fairly long. Click on the following entries in the table of contents below to jump directly there:

• The future is completely unpredictable
• No, really, the future is COMPLETELY unpredictable
• The future will be like the past, and therefore "predictable"
• The future contains both best and worst extremes from all possible worlds
• Conclusion

---

The future is completely unpredictable

It turns out that the future is completely unpredictable.

That may seem like a trivial statement. But most people have no idea just how true it is. A simple physics calculation may shed some light on this subject.

In the film franchise "Back to the Future", the main characters Marty McFly and Doctor "Doc" Emmett Brown travel through time and engage in various hijinks. Their travels alter the timeline in various ways, causing sometimes subtle, and sometimes drastic changes. Their goal throughout much of the films is to preserve the timeline they originally knew, with perhaps some small positive improvements.

But how likely are they to actually succeed? Can Marty, for instance, expect to return to 1985 and find things more or less as he left it, with perhaps a nicer car in his garage and an improved family? Let's say that Marty travels back in 1955, disturbs absolutely nothing by his direct contact, stays for only one second, then comes back to 1985. What can he expect to find?

Well, even if nothing is altered by his direct physical contact, Marty still exerts an additional gravitational force on his surroundings simply due to his mass. Since this is going to be an order of magnitude calculation, let's say that Marty has a mass of 100 kgs.

Now, consider the air molecules on the other side of the Earth from Marty. The Earth's diameter is 12.7e6 m. That is 12.7 million meters - I'll be using scientific notation (using e for the power of 10) and meters-kilograms-seconds throughout this calculation. Knowing that the universal gravitational constant is 6.67e-11 in mks units, we can use Newton's law of Universal Gravitation (a = Gm/r^2) to find that Marty causes an acceleration of 4.13e-23 m/s^2 to these air molecules.

Wow, that's like, nothing, right? In the one second that Marty is in 1955, that additional acceleration causes these air molecules to move an additional 2.07e-23 m that they would have otherwise not traveled. That's 0.000 000 000 000 000 000 000 0207 meters. What difference could be made by some air molecules moving such a small additional distance?

Consider a surface of one square meter on that other side of the Earth. The air molecules within 2.07e-23 m of that surface will have then collided with that surface and bounced back, due to Marty's additional gravitational pull. One square meter with 2.07e-23 m of thickness gives a volume of 2.07e-23 m^3, and this is the volume of air we're concerned about. There is 1 mole (6.022e23) of air in 22.4e-3 m^3 of volume in typical conditions, so that amounts to perhaps 556 air molecules that have bounced back against the surface.

Now, the important point here is that these air molecules are now OUT OF PLACE. They're somewhere that they're not suppose to be. As far as anyone from 1955 is concerned, the states of these 556 air molecules are unpredictable. If Marty had not traveled back in time, they would be somewhere completely different on the atomic scale. That means that they would collide with other air molecules that they were not supposed to collide with, while missing collisions that they were suppose to make.

The focus on collisions here is important. As long as the change that Marty causes is all smooth - say, by moving everything over by 2.07e-23 m - then any predictions made from 1955 would only be off by that much. Only negligible changes would occur from moving things by a negligible distance. But collisions change all that. If an air molecule makes a collision that it wasn't suppose to make, or misses a collision that was suppose to happen, then your predictions about the trajectory of the molecules are not just slightly off, they're completely wrong. That's why we're focused on the air molecules that bounced off the surface (whose trajectories are now completely unpredictable), rather than all the other air molecules (whose positions are now just all off by 2.07e-23 m).

Now, each air molecule experiences something like 1e10 collisions with another molecule each second. Each collision changes the trajectory of BOTH molecules. That is to say, 1e10 time a second, the number of air molecules whose trajectory is now completely unpredictable DOUBLES.

If we ignore the fact that a molecules may run into the same molecule multiple times, the number of molecules whose trajectory is now completely unpredictable would be 2^1e10 in one second. This is an absurdly large number, even when you're counting molecules. For all practical purposes, you can consider the microstate of ALL the air molecules around these original 556 molecules to have become completely unpredictable, in far less than one second. This cloud of unpredictability would rapidly spread, limited only by the diffusion and mixing of the air.

Furthermore, remember that this happens to every square meter of surface on the Earth. The upshot is that, from Marty's one second stay in 1955, even if he affects nothing by his direct touch, the microstate of the Earth's ENTIRE atmosphere rapidly becomes COMPLETELY UNPREDICTABLE, completely different than how it otherwise would have been. Something similar must also happen to all the liquids on the Earth, although the calculation will of course differ in detail.

Okay, so the future trajectory of all these molecules are completely unpredictable at this point. But really, can these molecular changes actually affect anything? What does it matter whether some molecules - or all molecules, for that matter - are here instead of there?

In the short term, it won't affect much. But there would be small changes. Microscopic differences can often affect the macroscopic world. The twinkling of stars, for example, is caused by the fluctuations in the momentary, localized density of the air. Photomultiplier tubes are devices specifically designed to cause macroscopically detectable measurements from microscopic events. Cosmic rays travelling through the atmosphere can be affected by the microstate of the air molecules, and they can cause neurons to fire when they hit your brain - perhaps causing a thought or a feeling you otherwise would not have had. The microscopic collisions of the molecules with other small particles causes Brownian motion - the random, jiggling movement of small particles suspended in water. So, a pollen grain would take a different random path in water, because Marty traveled back in time, for one second.

Now, do you think these changes still can't make any real differences? Try replacing "pollen grain" in the previous paragraph with "sperm".

Each sperm carries a different genetic code. Unless a specific sperm made it to the egg at the moment of your conception, you would not have been born. And the trajectory of that sperm would have been different, because the molecular motions in the fluids would have been different, all because Marty was in 1955 for one second and exerted a little bit of extra gravity. Forget about trying to get his parents to fall in love with one another - Marty was in all likelihood doomed to not exist the moment that he popped into 1955.

If you go review the argument and the calculations above, you'll see that the situation is actually far worse, in terms of predictability, than what I have presented. For example, altering the path of a sperm is not a particularly special way of affecting the future. In general, small changes - even microscopic changes - will eventually grow to cause macroscopic differences in time. This is called the butterfly effect: the name comes from an example where a butterfly flapping its wings can cause a hurricane on the other side of the Earth some time later.

The magnitude of the initial perturbation is also unnecessarily large in the example above. Remember, this doesn't affect just Marty; similar changes would happen all over the entire Earth. As if that wasn't enough, I once carried out another calculation which showed that even at INTERSTELLAR DISTANCES, a small change at another star will easily affect the microstate of things on Earth, which will then soon grows to make macroscopic differences.

So, that is the magnitude of the future's unpredictability: your very existence hinged on small changes happening in other parts of the galaxy.

But I don't want this to be one of those "see how wrong the movies are" articles. I like the "Back to the Future" trilogy. I don't like tearing things down just for the sake of tearing them down. So I'll end this section with the following:

I think the truest thing in those movies is what Doc says at the very end: "your future hasn't been written yet. No one's has. Your future is whatever you make it. So make it a good one." That may or may not be philosophically true in the deepest sense. But what is absolutely certain is that Doc's advice is effectively true in a practical sense. What the above calculation shows is that you cannot hope to indefinitely predict the details of your future. No one can. In that sense, your future is not yet written. What you can do, however, is act in the present to control the short moments ahead, and focus on the bigger picture to extend the lifetime of our planning before the butterfly effect kicks in. We then adapt and adjust, one step at a time. That is how we make the future.

---

No, really, the future is COMPLETELY unpredictable

I demonstrated earlier that even if Marty McFly went back in time for just one second, just his gravitational influence would irrevocably change the future. I then claimed that this is actually overkill: that even small changes at interstellar distances will affect things on Earth. Let's see about that.

(Most of this section is a walk-through of a calculation. Jump to the end if you just want to see the conclusion. The conventions here are the same as in the last section: all numbers and equations are in mks units. The powers of 10 for scientific notation is indicated following 'e'.)

The distance to the Andromeda Galaxy is 2.5 million light-years. That's 2.4e22 meters.

Consider a single water molecule somewhere in that galaxy. Water is a polar molecule - that is, it has positive and negative parts, separated by some distance. This creates an electric dipole moment. For a single water molecule, this dipole moment has a magnitude of 6.2e-30 coulomb-meters.

Now, dipole moments exert an electric field that depends on their spatial orientation. The full equation for that electric field can be found at any number of places, but we're really only interested in how much this field can change depending on the orientation. It turns out that it can change by about 1/(4 π ε0) * p/r^3. "p" is the magnitude of the dipole moment, "r" is the distance from that dipole, and ε0 is the permittivity of free space; it's a physical constant with a value of 8.85e-12. Plugging in all these numbers, we get a value of 4.0e-87 newtons/coulomb as the electric field.

So, depending on the orientation of a single water molecule in the Andromeda Galaxy, the electric field on Earth can change by about as much as 4.0e-87 newtons/coulomb. That electric field will exert a force of 6.4e-106 newtons on an elementary electric charge here on Earth.

Now, let's take a moment to appreciate how minuscule this number is. Some mathematical tools will simply not compute a number this small, and just display it as "0". It is on the order of a millionth of a googolth of a newton. After all, what can we expect from changing the orientation of a single water molecule in the Andromeda Galaxy? How can that possibly affect us on Earth?

If this force acts on an ionized air molecule - say, a singly ionized nitrogen molecule - that would cause an acceleration of F/m = 1.4e-80 m/s^2. If this acceleration acts for 1.4e-10 seconds (0.14 nanoseconds), it will move this ion by 1.4e-100 meters. This, then, will affect the future collisions of this ion with other air molecules.

So, to review: we are changing the orientation of a single water molecule, somewhere in the Andromeda Galaxy, for 0.14 nanoseconds. We are considering how this would affect the Earth. So far, we've determined that it would move an ionized nitrogen molecule by 1.4e-100 meters (1.4 googolth of a meter), which would then influence the future collisions of that molecule.

We'll model the collisions as if they were classical collisions with hard spheres - basically, as if the molecules were billiard balls. In such collisions, changing the position of the incoming billiard ball by x causes it to bounce off at a different angle. That difference, under some simplifying assumptions, is given by 2 x/d, where d is the diameter of the "billiard balls". In our case these are air molecules, which have a diameter of about 4e-10 meters. So, after bouncing off, our original ionized molecule is now moving at a different angle - one that's 2 x/d = 6.9e-91 radians away from the direction it would have otherwise bounced to. This difference in angle will then cause it to run into the next air molecule from a different position, which will then cause another difference in bouncing angle, and so on and so forth, in an iterative fashion. Eventually, after enough bounces, the deflection will be large enough that our ionized molecule will entirely miss another molecule that it would have otherwise hit. How many collisions does this take? I wrote a small bit of code in Python to find out, using typical values for the relevant conditions for air (such as the time between collisions, or the path length that a molecule travels between collisions):

acceleration = 1.4e-80
path_length = 7e-8
time = 1.4e-10
diameter = 4e-10

n = 0
impact_parameter = 0.5*acceleration*time**2

def one_collision(incoming_d_impact_parameter):
    new_deflection = 2*incoming_d_impact_parameter/diameter
    new_d_impact_parameter = new_deflection*path_length
    return {
        "deflection":new_deflection, 
        "impact_parameter":new_d_impact_parameter}

collision = True
while collision:
    result = one_collision(impact_parameter)
    impact_parameter = result["impact_parameter"]
    n += 1
    out_string = 
        "collisions:" + "{:2d}".format(n) + 
        "  impact_parameter:" + "{:.2e}".format(result["impact_parameter"]) + 
        "  deflection:" + "{:.2e}".format(result["deflection"])
    print out_string
    if result["impact_parameter"] > diameter:
        collision = False
        print "done in " + "{:2d}".format(n) + " collisions"

Running this code gives the following output:

collisions: 1  impact_parameter:4.80e-98  deflection:6.86e-91
collisions: 2  impact_parameter:1.68e-95  deflection:2.40e-88
collisions: 3  impact_parameter:5.88e-93  deflection:8.40e-86
collisions: 4  impact_parameter:2.06e-90  deflection:2.94e-83
collisions: 5  impact_parameter:7.21e-88  deflection:1.03e-80
collisions: 6  impact_parameter:2.52e-85  deflection:3.60e-78
collisions: 7  impact_parameter:8.83e-83  deflection:1.26e-75
collisions: 8  impact_parameter:3.09e-80  deflection:4.41e-73
collisions: 9  impact_parameter:1.08e-77  deflection:1.54e-70
collisions:10  impact_parameter:3.78e-75  deflection:5.41e-68
collisions:11  impact_parameter:1.32e-72  deflection:1.89e-65
collisions:12  impact_parameter:4.64e-70  deflection:6.62e-63
collisions:13  impact_parameter:1.62e-67  deflection:2.32e-60
collisions:14  impact_parameter:5.68e-65  deflection:8.11e-58
collisions:15  impact_parameter:1.99e-62  deflection:2.84e-55
collisions:16  impact_parameter:6.96e-60  deflection:9.94e-53
collisions:17  impact_parameter:2.44e-57  deflection:3.48e-50
collisions:18  impact_parameter:8.52e-55  deflection:1.22e-47
collisions:19  impact_parameter:2.98e-52  deflection:4.26e-45
collisions:20  impact_parameter:1.04e-49  deflection:1.49e-42
collisions:21  impact_parameter:3.65e-47  deflection:5.22e-40
collisions:22  impact_parameter:1.28e-44  deflection:1.83e-37
collisions:23  impact_parameter:4.48e-42  deflection:6.39e-35
collisions:24  impact_parameter:1.57e-39  deflection:2.24e-32
collisions:25  impact_parameter:5.48e-37  deflection:7.83e-30
collisions:26  impact_parameter:1.92e-34  deflection:2.74e-27
collisions:27  impact_parameter:6.72e-32  deflection:9.60e-25
collisions:28  impact_parameter:2.35e-29  deflection:3.36e-22
collisions:29  impact_parameter:8.23e-27  deflection:1.18e-19
collisions:30  impact_parameter:2.88e-24  deflection:4.11e-17
collisions:31  impact_parameter:1.01e-21  deflection:1.44e-14
collisions:32  impact_parameter:3.53e-19  deflection:5.04e-12
collisions:33  impact_parameter:1.23e-16  deflection:1.76e-09
collisions:34  impact_parameter:4.32e-14  deflection:6.17e-07
collisions:35  impact_parameter:1.51e-11  deflection:2.16e-04
collisions:36  impact_parameter:5.29e-09  deflection:7.56e-02
done in 36 collisions

"impact_parameter" is the difference in position as our ion approaches the next molecule. You see that, after the 36th collision, this value exceeds the diameter of a molecule, causing our ion to miss a collision that would have otherwise taken place. Since air molecules make a collision about every 1.4e-10 seconds, these 36 collisions only take 5.0e-9 seconds - that is, 5 nanoseconds.

The rest of the story is similar to how they occurred in the last section. A missed collision means that the states of the two molecules that would have been involved are now completely different, completely changed from what they would otherwise be. Then each of their future collisions also completely changes the states of these new molecules they run into. This means that with each round of collisions, the number of affected air molecules doubles. In far less than a second, all of the molecules around the original ion are affected. Furthermore, this is happening starting from every single ionized molecule in Earth's atmosphere - so in no time at all, the microstate of Earth's entire atmosphere is completely changed. This then leads to macroscopic changes (like Marty McFly never being born) soon enough.

So, that is the magnitude of the future's unpredictability. That is the degree of the universe's inter-connectivity and complexity. A SINGLE WATER MOLECULE, located in ANOTHER GALAXY, changing its orientation for a FRACTION OF A NANOSECOND, will completely alter the microstate of the Earth's atmosphere nearly instantly (after accounting for the speed-of-light delay), and change our macroscopic future in short order. Your life, brain, and very existence is the result of all these effects combining to create you in your current state. "Subtle is the LORD" indeed.

---

The future will be like the past, and therefore "predictable"

And now for something completely different: It's easy to predict the future. It'll be basically like the past.

How could that be? Didn't I just explain how unpredictable the future is? Isn't everything changing all the time? Are we not living in an era of unprecedented growth and technological advancement? Isn't the world not only changing, but changing faster and faster? I mean, just look at the graph of the world population: look at that absolute explosion in recent years. And similar graphs can be made for technological progress as well. Isn't it absolutely clear that the present is completely different than the past, that we are on the cusp of something disastrous or transcendent, and that the future will be far more different still?

Well, in a sense, I guess that's true. But that's pretty much always been true. That's what I mean by saying the future will be like the past. All those hyperbolic things said about the future could have been said at most other times in the past - A hundred, two hundred, or perhaps even thousands of years ago. And they would have been about as right then as we are now in saying these things. That is simply the nature of exponential growth.

Here's what I mean: if we assume that humanity's growth - whether it's measured in population or in technological development - follows an exponential curve, then the future can always be exactly mapped onto the present, or the past, through some simple transformations. Let me demonstrate: consider the following graph:

That exponential graph may represent world population or technological growth or whatever. Isn't it clear that there are two distinct regions in that graph, the flat region and the rapid rise before the end? Let's zoom in to the transition between the two regions:

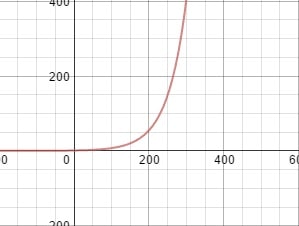
Isn't that where we are in history? At that unique and crucial point where we leave off being "flat" to where everything blows up and goes off the scale?

Well, let's not do anything hasty based on that line of thinking. After all, I can make any other region of the graph look exactly like that "unique and crucial point" by simply focusing on that new point and re-normalizing the y-axis. The following is a graph of the exact same function as before at a different x-value, viewed according to the treatment I just prescribed:

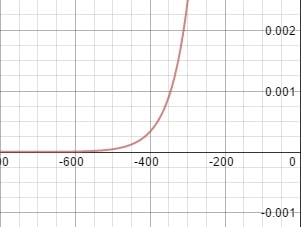
Note that the view window is now focused around -400 instead of +200, and the y-axis has been re-scaled. But the shape of the function is exactly the same as it was before. You can try this for yourself  - desmos is a good online graphing calculator - and see that it's true, starting with any exponential growth function. Algebraically, this is because if you

1. take any exponential function (e^kx),
2. and translate it horizontally by any amount (e^k(x+x0)),
3. and vertically scale it to re-normalize things so that the new y-value becomes the new normal ( 1/e^kx0 * e^k(x+x0))
4. then you get back your original function. (e^kx)

This means that if you transport yourself to any time in history, and get used to your environment so that this new time period becomes your new "normal", then the pace of the changes around you will look the same as it does now. In fact, it'll look the same at any other time in history. There is therefore nothing new under the sun, and the future will be like the past.

You can even test this with events within living memory. Consider that archetypal example of modern progress: the advancements in computers. Back when I first started to really look at computers, a "gigabyte" was an unheard amount of storage for a computer to have. If you had told me then of a future of such rapid progress where a computer's storage increased by many gigabytes in a couple of years, I might have marveled at this hyper-technological future where such wonders were possible. I might have thought that everything I knew would be thrown out and be completely changed in this new world. But of course, this is the future we live in now, and the increase from 64 to 128 GB between iPhone 5 and 6 is not some world-shattering news.

Now, one may argue that the pace of growth has actually been even faster than merely exponential growth - there may be something to be said for that. Certainly, this graph of the world's population has distinct regions, and the growth is not only exponential, but there are period where the exponential growth rate itself increases. But I think it's too much to conclude that this is the definitive, continuous pattern in human progress. I think that the graph can be best interpreted by saying that human growth rate is usually exponential, but that at the rate of growth jumps to a new value at certain moments in history (the beginning of civilization, the industrial revolution, etc). We may be in such a moment in history, but even so, the rate of change would not be fundamentally different than they were during the industrial revolution. It's exciting, to be sure, but it's not the end of the world, and the future will continue to be more or less like the past.

---

The future contains both good and bad extremes from all possible worlds

What I am about to say here is less certain than what I have said in the previous sections; it's a half-formed thought, which I only post because I think it may be more important than my other thoughts, especially regarding the future of human society. In short, this section will more nebulous compared to the others in the series.

The thought here is simple:

I wonder if humans only learn through experiments.

That doesn't seem important? Let me rephrase that:

I wonder if humans only learn through experiences. Or, to put in a little bit more detail: I wonder if humans only learn through experiencing mistakes.

Note that I intend for this to apply to human societies as a whole rather than to just individuals. You see, when we start talking about the future, we sometimes have this idea that we'll reach some kind utopia, where all our social problems will be solved. We just have to learn to treat each other as fellow humans, right? Surely with the right education and policies, and with our ever increasing powers of science and technology, we'll eventually reach that eternally perfect society?

If there were some cap to the amount of beauty in the universe, or a limit to human potential, perhaps. Then we will eventually reach that maximum and stay there. Then we could maybe be like those space-faring aliens whom I mentioned before, who are fated merely to rule the stars then perish with the universe.  But I don't think that this is the case. I don't think that we are bounded in that way.

So, if we have infinite potential, and we can only learn though experiencing mistakes, what will the future be like? Well, here is one possible future history:

One thing that it's popular to wonder about nowadays is whether we should make a computer that's smarter than a human (whatever that means). Now, this is a question of immense importance, but if we can only learn through experiencing mistakes, the only way to settle the question would be to try it, then see the results: there is no historically analogous previous situation to draw on. And getting the question wrong may be disastrous.

So then, after the disastrous Skynet wars of 2050, humanity might finally agree on what kind of artificial intelligence to build. This will allow us to colonize the other planets in the solar system - and we'll then ask questions like "how should we share the resources between the different planets?" This type of question has never been asked on a planetary scale, so there will be no historically analogous previous situation to draw on. Even a super-intelligent AI might not be able to reason with no data. So again, we'll just have to try out different models of planetary economics, and learn by trial and error. So, Mars and Jupiter may prosper, but then the citizens of Mercury and Earth may suffer for generations under an oppressive system of planetary trade.

But eventually, that will get sorted out, after a great deal of human suffering. But at this point, the differences between the haves and the have-nots, at the planetary scale, subject to different planetary conditions, combined with the genetic tinkering that's been going on, may threaten to tear apart our conception of "the human race". What should we do about this? Should we allow a portion of humanity to evolve separately from the rest? Again, this will be the first time in history where we can meaningfully ask this question, and the only recourse may be to try different policies and make our mistakes. And given the momentous nature of the question, the consequences for getting them wrong will be correspondingly tragic.

Of course, these scenarios are only projected from the mind of an early 21st century human. More likely, the kinds of issues faced by these future people will be completely incomprehensible to us; just as, say, the 20th century's nuclear ideology of Mutually Assured Destruction would be unimaginable to a prehistoric human. And each time, because of this unimaginable newness and lack of historical precedence, it may be that mistakes may have to be made in order to learn from them. And each time, because of humanity's increased power and the larger scale and scope of the problem, the mistakes will be more costly, even as the benefits of getting it right propels us further into the future.

What kind of future is this? It's actually a strangely hopeful but tragic one. Our powers and glory will perpetually increase - but with many steps being paid for with ever more costly mistakes. You think that slavery or the killing fields were bad? The instances of such tragedies will only increase in enormity. On the other hand, you think that modern democracies and smartphones are good? The overall trajectory for history as a whole will continue to be upwards, as all these things will perpetually be superseded by something better.

As I said, I'm unsure about all this; but it seems likely, as it's been the case thus far in history.

---

Conclusion

Trying to predict the future is a good way to put your foot in your mouth. It's hard enough to just think about the future, let alone predict it. Even in this series of posts, all I have done is provide some rules of thumb: the future is impossible to predict. The future will be like the past. The future will have both good and bad in it, in ever increasing measure.

Some of these rules are vague, and may even seem contradictory. How could the future be unpredictable yet still be like the past? But the possibilities concerning the future are rich enough that such superficial paradoxes can be easily resolved. And when we harmonize these rules, a coherent pattern does seem to emerge: the future cannot be predicted in detail, and ever larger events are considered "details" as we consider times further into the future. However, there are real patterns that repeat themselves in time. The details get averaged out if you consider them in aggregate. So in these ways, at a sufficiently broad level, the future can be predicted from the past. As far as humans are concerned, this means that our history will grow in both glory and tragedy in ever larger degree. We will continue to grow, yet the inevitable, unpredictable, fluctuating "details" will cause disasters in correspondingly larger degrees.

So what should we do? What can we predict or plan for? I really don't know. I don't know how right I am in my thoughts about the future. So, in the end, as it often happens, all I can say is what has already been said in the Bible:

Now listen, you who say, "Today or tomorrow we will go to this or that city, spend a year there, carry on business and make money." Why, you do not even know what will happen tomorrow. What is your life? You are a mist that appears for a little while and then vanishes. Instead, you ought to say, "If it is the Lord's will, we will live and do this or that."

I am indeed only a mist - a "detail" that's here by accident and whose affect will be averaged out by other similarly fluctuating detail. That is why I can only say, "If it is the Lord's will, I will live and do this or that." But in that Divine will, I believe that I am a positive part of the pattern that's woven throughout time, a pattern that continues into eternity.

---

You may next want to read:
Christian predictions on the future of science (part 1)
The biblical timeline of the universe
Another post, from the table of contents